“Anisotropic elasticity for inversion-safety and element rehabilitation” by Kim, Goes and Iben
Conference:
Type(s):
Title:
- Anisotropic elasticity for inversion-safety and element rehabilitation
Session/Category Title: Deformation and FEM
Presenter(s)/Author(s):
Abstract:
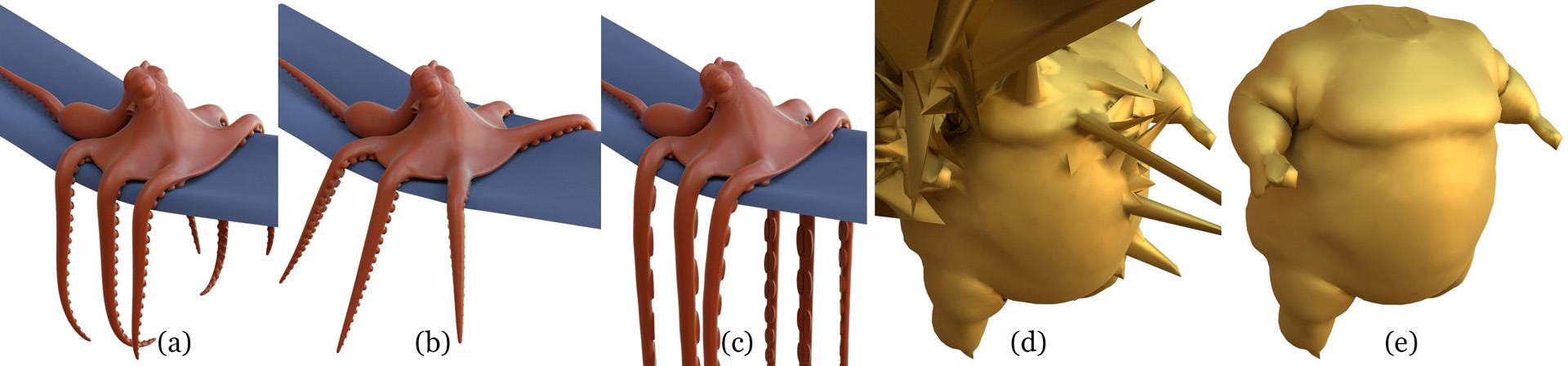
We present an analysis of anisotropic hyperelasticity, specifically transverse isotropy, that obtains closed-form expressions for the eigendecompositions of many common energies. We then use these to build fast and concise Newton implementations. We leverage our analysis in two separate applications. First, we show that existing anisotropic energies are not inversion-safe, and contain spurious stable states under large deformation. We then propose a new anisotropic strain invariant that enables the formulation of a novel, robust, and inversion-safe energy. The new energy fits completely within our analysis, so closed-form expressions are obtained for its eigensystem as well. Secondly, we use our analysis to rehabilitate badly-conditioned finite elements. Using this method, we can robustly simulate large deformations even when a mesh contains degenerate, zero-volume elements. We accomplish this by swapping the badly-behaved isotropic direction with a well-behaved anisotropic term. We validate our approach on a variety of examples.
References:
1. V. Alastru?, E. Pe?a, M. Mart?nez, and M. Doblar?. 2008. Experimental study and constitutive modelling of the passive mechanical properties of the ovine infrarenal vena cava tissue. J. of Biomechanics 41, 14 (2008), 3038–3045.Google ScholarCross Ref
2. M. Alexa, D. Cohen-Or, and D. Levin. 2000. As-rigid-as-possible Shape Interpolation. In Proceedings of SIGGRAPH. 157–164. Google ScholarDigital Library
3. D. Baraff and A. Witkin. 1998. Large Steps in Cloth Simulation. In Proceedings of SIGGRAPH. 43–54. Google ScholarDigital Library
4. J. Barbi? and Doug L. James. 2005. Real-Time Subspace Integration for St. Venant-Kirchhoff Deformable Models. ACM Trans. Graph. 24, 3 (2005), 982–990. Google ScholarDigital Library
5. A. Bargteil, C. Wojtan, J. Hodgins, and G. Turk. 2007. A Finite Element Method for Animating Large Viscoplastic Flow. ACM Trans. Graph. 26, 3 (2007). Google ScholarDigital Library
6. T. Belytschko, W. Liu, B. Moran, and K. Elkhodary. 2013. Nonlinear finite elements for continua and structures. John Wiley & Sons.Google Scholar
7. S. Blemker, P. Pinsky, and S. Delp. 2005. A 3D model of muscle reveals the causes of nonuniform strains in the biceps brachii. J. of Biomechanics 38, 4 (2005), 657–665.Google ScholarCross Ref
8. J. Bonet and R. D. Wood. 2008. Nonlinear continuum mechanics for finite element analysis. Cambridge University Press.Google Scholar
9. S. Bouaziz, S. Martin, T. Liu, L. Kavan, and M. Pauly. 2014. Projective Dynamics: Fusing Constraint Projections for Fast Simulation. ACM Trans. Graph. 33, 4 (2014). Google ScholarDigital Library
10. J. Cai. 2016. Simulating Deformable Models with Anisotropic Materials. Ph.D. Dissertation. Nanyang Technological University.Google Scholar
11. G. Chagnon, M. Rebouah, and D. Favier. 2015. Hyperelastic energy densities for soft biological tissues: a review. J. of Elasticity 120, 2 (2015), 129–160.Google ScholarCross Ref
12. J. Chen, H. Bao, T. Wang, M. Desbrun, and J. Huang. 2018. Numerical Coarsening Using Discontinuous Shape Functions. ACM Trans. Graph. 37, 4, Article 120 (July 2018), 12 pages. Google ScholarDigital Library
13. P. Ciarletta, I. Izzo, S. Micera, and F. Tendick. 2011. Stiffening by fiber reinforcement in soft materials: a hyperelastic theory at large strains and its application. Journal of the Mechanical Behavior of Biomedical Materials 4, 7 (2011), 1359–1368.Google ScholarCross Ref
14. C. Forest, H. Delingette, and N. Ayache. 2002. Removing tetrahedra from a manifold mesh. In Proceedings of Computer Animation. 225–229. Google ScholarDigital Library
15. T. Gast, C. Schroeder, A. Stomakhin, C. Jiang, and J. Teran. 2015. Optimization Integrator for Large Time Steps. IEEE Transactions on Visualization and Computer Graphics 21, 10 (2015), 1103–1115. Google ScholarDigital Library
16. G. H. Golub and C. F. Van Loan. 2012. Matrix computations. Vol. 3. JHU Press.Google Scholar
17. C. Gonzalez-Ochoa, D. Eberle, and R. Dressel. 2002. Dynamic Simulation of Wing Motion on “Reign of Fire”. In ACM SIGGRAPH Sketches. 174–174. Google ScholarDigital Library
18. G. Holzapfel. 2005. Similarities between soft biological tissues and rubberlike materials. In Constitutive Models for Rubber IV, Vol. 4. 607.Google Scholar
19. G. Holzapfel, T. Gasser, and R. Ogden. 2000. A new constitutive framework for arterial wall mechanics and a comparative study of material models. Journal of Elasticity and the Physical Science of Solids 61, 1–3 (2000), 1–48.Google ScholarCross Ref
20. C. Horgan and G. Saccomandi. 2005. A new constitutive theory for fiber-reinforced incompressible nonlinearly elastic solids. Journal of the Mechanics and Physics of Solids 53, 9 (2005), 1985–2015.Google ScholarCross Ref
21. Y. Hu, Q. Zhou, X. Gao, A. Jacobson, D. Zorin, and D. Panozzo. 2018. Tetrahedral Meshing in the Wild. ACM Trans. Graph. 37, 4, Article 60 (July 2018), 14 pages. Google ScholarDigital Library
22. H. Iben. 2007. Generating Surface Crack Patterns. Ph.D. Dissertation. University of California, Berkeley. Google ScholarDigital Library
23. G. Irving, R. Kautzman, G. Cameron, and J. Chong. 2008. Simulating the Devolved: Finite Elements on WALL-E. In ACM SIGGRAPH Talks. Article 54, 1 pages. Google ScholarDigital Library
24. G. Irving, J. Teran, and R. Fedkiw. 2004. Invertible Finite Elements for Robust Simulation of Large Deformation. In SIGGRAPH/Eurog. Symp. on Comp. Anim. 131–140. Google ScholarDigital Library
25. A. Jacobson, Z. Deng, L. Kavan, and J. P. Lewis. 2014. Skinning: Real-time Shape Deformation. In ACM SIGGRAPH Courses. Article 24, 24:1–24:1 pages. Google ScholarDigital Library
26. P. M. Knupp. 2003. Algebraic mesh quality metrics for unstructured initial meshes. Finite Elements in Analysis and Design 39, 3 (2003), 217–241. Google ScholarDigital Library
27. D. Koschier, J. Bender, and N. Thuerey. 2017. Robust extended Finite Elements for Complex Cutting of Deformables. ACM Trans. Graph. 36, 4, Article 55 (July 2017). Google ScholarDigital Library
28. F. Labelle and J. Shewchuk. 2007. Isosurface Stuffing: Fast Tetrahedral Meshes with Good Dihedral Angles. ACM Trans. Graph. 26, 3, Article 57 (July 2007). Google ScholarDigital Library
29. S. Lee and D. Terzopoulos. 2006. Heads Up!: Biomechanical Modeling and Neuromuscular Control of the Neck. ACM Trans. Graph. 25, 3 (July 2006), 1188–1198. Google ScholarDigital Library
30. S. Lee, R. Yu, J. Park, M. Aanjaneya, E. Sifakis, and J. Lee. 2018. Dexterous Manipulation and Control with Volumetric Muscles. ACM Trans. Graph. 37, 4, Article 57 (July 2018), 13 pages. Google ScholarDigital Library
31. Y. Lee, M. Park, T. Kwon, and J. Lee. 2014. Locomotion Control for Many-muscle Humanoids. ACM Trans. Graph. 33, 6, Article 218 (Nov. 2014), 11 pages. Google ScholarDigital Library
32. Y. Li and J. Barbi?. 2015. Stable anisotropic materials. IEEE Transactions on Visualization and Computer Graphics 21, 10 (2015), 1129–1137. Google ScholarDigital Library
33. Y. Li, H. Xu, and J. Barbi?. 2017. Enriching Triangle Mesh Animations with Physically Based Simulation. IEEE Transactions on Visualization and Computer Graphics 23, 10 (2017), 2301–2313.Google ScholarDigital Library
34. T. Liu, S. Bouaziz, and L. Kavan. 2017. Quasi-Newton Methods for Real-Time Simulation of Hyperelastic Materials. ACM Trans. Graph. 36, 3 (2017). Google ScholarDigital Library
35. P.-L. Manteaux, C. Wojtan, R. Narain, S. Redon, F. Faure, and M.-P. Cani. 2017. Adaptive Physically Based Models in Computer Graphics. Comput. Graph. Forum 36, 6 (Sept. 2017), 312–337. Google ScholarDigital Library
36. A. McAdams, Y. Zhu, A. Selle, M. Empey, R. Tamstorf, J. Teran, and E. Sifakis. 2011. Efficient Elasticity for Character Skinning with Contact and Collisions. ACM Trans. Graph. 30, 4 (2011). Google ScholarDigital Library
37. A. Milne, M. McLaughlin, R. Tamstorf, A. Stomakhin, N. Burkard, M. Counsell, J. Canal, D. Komorowski, and E. Goldberg. 2016. Flesh, Flab, and Fascia Simulation on Zootopia. In ACM SIGGRAPH Talks. Article 34, 2 pages. Google ScholarDigital Library
38. N. Molino, Z. Bao, and R. Fedkiw. 2004. A Virtual Node Algorithm for Changing Mesh Topology During Simulation. ACM Trans. Graph. 23, 3 (Aug. 2004), 385–392. Google ScholarDigital Library
39. N. Molino, R. Bridson, J. Teran, and R. Fedkiw. 2003. A Crystalline, Red Green Strategy for Meshing Highly Deformable Objects with Tetrahedra.. In International Meshing Roundtable. 103–114.Google Scholar
40. D. Nolan, A. Gower, M. Destrade, R. Ogden, and J. McGarry. 2014. A robust anisotropic hyperelastic formulation for the modelling of soft tissue. Journal of the Mechanical Behavior of Biomedical Materials 39 (2014), 48–60.Google ScholarCross Ref
41. G. Picinbono, J. Lombardo, H. Delingette, and N. Ayache. 2000. Anisotropic elasticity and force extrapolation to improve realism of surgery simulation. In IEEE International Conference on Robotics and Automation. IEEE, 596–602.Google Scholar
42. Y. Qiu. 2018. Spectra, a C++ Library For Large Scale Eigenvalue Problems. https://spectralib.org.Google Scholar
43. K. Rupp, P. Tillet, F. Rudolf, J. Weinbub, A. Morhammer, T. Grasser, A. J?ngel, and S. Selberherr. 2016. ViennaCL, Linear Algebra Library for Multi-and Many-Core Architectures. SIAM Journal on Scientific Computing 38, 5 (2016), 412–439.Google ScholarCross Ref
44. P. Sachdeva, S. Sueda, S. Bradley, M. Fain, and D. K. Pai. 2015. Biomechanical Simulation and Control of Hands and Tendinous Systems. ACM Trans. Graph. 34, 4, Article 42 (July 2015). Google ScholarDigital Library
45. S. Saito, Z. Zhou, and L. Kavan. 2015. Computational Body building: Anatomically-based Modeling of Human Bodies. ACM Trans. Graph. 34, 4, Article 41 (July 2015). Google ScholarDigital Library
46. T. Schneider, Y. Hu, J. Dumas, X. Gao, D. Panozzo, and D. Zorin. 2018. Decoupling Simulation Accuracy from Mesh Quality. ACM Trans. Graph. (2018). Google ScholarDigital Library
47. J. Shewchuk. 2002. What is a good linear finite element? interpolation, conditioning, anisotropy, and quality measures. University of California at Berkeley 73 (2002).Google Scholar
48. B. Smith, F. de Goes, and T. Kim. 2018. Stable Neo-Hookean Flesh Simulation. ACM Trans. Graph. 37, 2 (2018). Google ScholarDigital Library
49. B. Smith, F. de Goes, and T. Kim. 2019. Analytic Eigensystems For Isotropic Distortion Energies. ACM Trans. Graph. (2019). Google ScholarDigital Library
50. O. Sorkine and M. Alexa. 2007. As-rigid-as-possible surface modeling. In Eurog. Symposium on Geometry processing, Vol. 4. Google ScholarDigital Library
51. A. Stomakhin, R. Howes, C. Schroeder, and J. M. Teran. 2012. Energetically Consistent Invertible Elasticity. In ACM SIGGRAPH/Eurog. Symp. Comp. Anim. 25–32. Google ScholarDigital Library
52. J. Teran, S. Blemker, V. Hing, and R. Fedkiw. 2003. Finite volume methods for the simulation of skeletal muscle. In SIGGRAPH/Eurog. Symp. on Comp. Anim. 68–74. Google ScholarDigital Library
53. J. Teran, E. Sifakis, G. Irving, and R. Fedkiw. 2005. Robust Quasistatic Finite Elements and Flesh Simulation. In ACM SIGGRAPH/Eurog. Symp. on Comp. Anim. 181–190. Google ScholarDigital Library
54. Y. Tong, S. Lombeyda, A. N. Hirani, and M. Desbrun. 2003. Discrete Multiscale Vector Field Decomposition. ACM Trans. Graph. 22, 3 (July 2003), 445–452. Google ScholarDigital Library
55. B. Wang, Y. Zhao, and J. Barbi?. 2017. Botanical Materials Based on Biomechanics. ACM Trans. Graph. 36, 4, Article 135 (July 2017), 13 pages. Google ScholarDigital Library
56. H. Wang and Y. Yang. 2016. Descent Methods for Elastic Body Simulation on the GPU. ACM Trans. Graph. 35, 6 (2016). Google ScholarDigital Library
57. J. Weiss, B. Maker, and S. Govindjee. 1996. Finite element implementation of incompressible, transversely isotropic hyperelasticity. Computer Methods in Applied Mechanics and Engineering 135, 1–2 (1996), 107–128.Google ScholarCross Ref
58. C. Wojtan and G. Turk. 2008. Fast viscoelastic behavior with thin features. ACM Trans. Graph. 27, 3 (2008), 47. Google ScholarDigital Library
59. A. Wong, D. Eberle, and T. Kim. 2018. Clean Cloth Inputs: Removing Character Self-intersections with Volume Simulation. In ACM SIGGRAPH Talks. Article 42. Google ScholarDigital Library
60. H. Xu, F. Sin, Y. Zhu, and J. Barbi?. 2015. Nonlinear Material Design Using Principal Stretches. ACM Trans. Graph. 34, 4 (2015). Google ScholarDigital Library