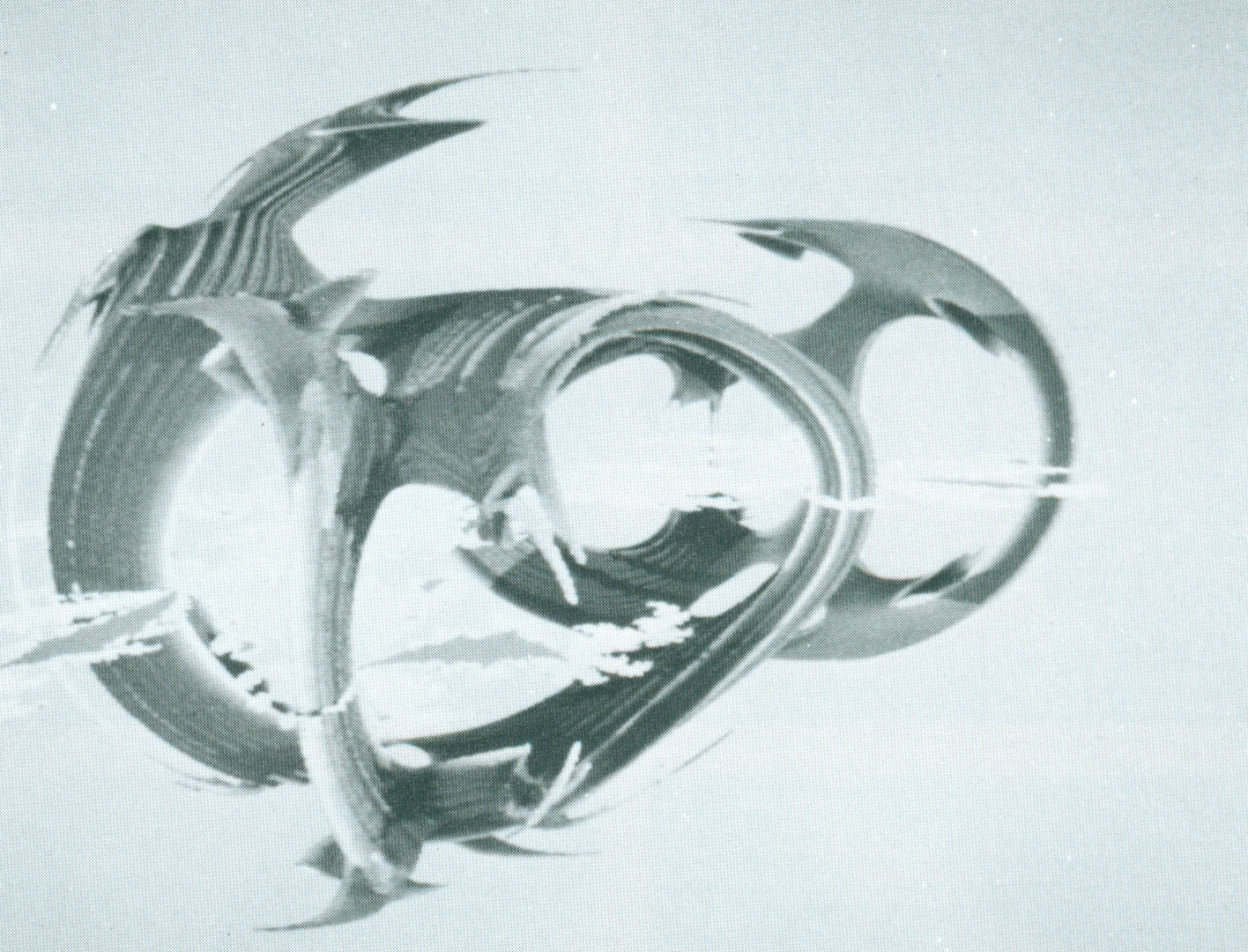
“A Close Encounter in the Fourth Dimension” by Norton and Melton
Notice: Pod Template PHP code has been deprecated, please use WP Templates instead of embedding PHP. has been deprecated since Pods version 2.3 with no alternative available. in /data/siggraph/websites/history/wp-content/plugins/pods/includes/general.php on line 518
Conference:
- SIGGRAPH 1988 More animation videos from SIGGRAPH 1988:
Notice: Array to string conversion in /data/siggraph/websites/history/wp-content/plugins/siggraph-archive-plugin/src/next_previous/source.php on line 345
Notice: Array to string conversion in /data/siggraph/websites/history/wp-content/plugins/siggraph-archive-plugin/src/next_previous/source.php on line 345
SIGGRAPH Video Review:
Track:
- 30
Title:
- A Close Encounter in the Fourth Dimension
Director(s):
Company / Institution / Agency:
- IBM Corporation
Description:
This animation illustrates the Julia set of the formula (1.475+ 906i)x(1-×). The Julia set, a geometric object intrinsically associated with the formula, naturally resides in the 4-D algebra known as the quaternions. A 3-D slice of the Julia set is illustrated emerging from a planar (2-D) slice. The Julia set is a fractal; i.e., a shape that possesses detail at all scales of magnification. One research purpose is to explore the introduction of additional detail into a computer image as the viewer approaches a surface. In the animation, portions of the Julia set are computed at two and four times the original resolution, and are merged into the original image as the viewer approaches the surface.
Additional Contributors:
Modeling and rendering: Alan Norton
Animation: Evelyn Melton
Fractal music: Curtis Bahn, Center for Computer Music,Brooklyn College, CUNY, C .Dodge, director
Special Thanks: G.Braudaway, J .Hall, T.Kay, F.Chan, S.Harvey, P.Zackowski, O.Goggins, M.Henderson, J .Zienda
