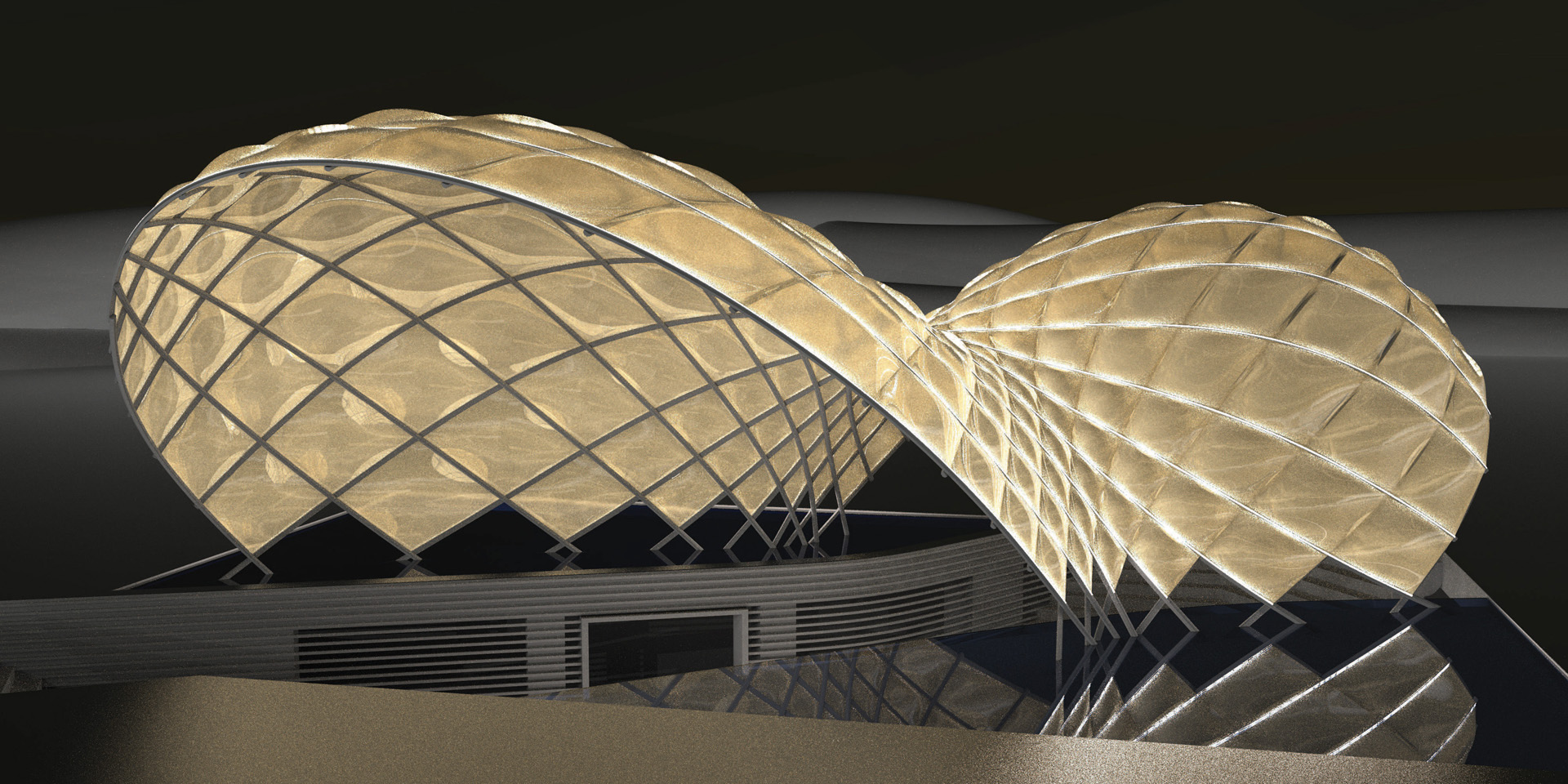
“X-Shells: a new class of deployable beam structures” by Panetta, Konaković-Luković, Isvoranu, Bouleau and Pauly
Conference:
Type(s):
Title:
- X-Shells: a new class of deployable beam structures
Session/Category Title: Fabrication
Presenter(s)/Author(s):
Abstract:
We present X-shells, a new class of deployable structures formed by an ensemble of elastically deforming beams coupled through rotational joints. An X-shell can be assembled conveniently in a flat configuration from standard elastic beam elements and then deployed through force actuation into the desired 3D target state. During deployment, the coupling imposed by the joints will force the beams to twist and buckle out of plane to maintain a state of static equilibrium. This complex interaction of discrete joints and continuously deforming beams allows interesting 3D forms to emerge. Simulating X-shells is challenging, however, due to unstable equilibria at the onset of beam buckling. We propose an optimization-based simulation framework building on a discrete rod model that robustly handles such difficult scenarios by analyzing and appropriately modifying the elastic energy Hessian. This real-time simulation method forms the basis of a computational design tool for X-shells that enables interactive design space exploration by varying and optimizing design parameters to achieve a specific design intent. We jointly optimize the assembly state and the deployed configuration to ensure the geometric and structural integrity of the deployable X-shell. Once a design is finalized, we also optimize for a sparse distribution of actuation forces to efficiently deploy it from its flat assembly state to its 3D target state. We demonstrate the effectiveness of our design approach with a number of design studies that highlight the richness of the X-shell design space, enabling new forms not possible with existing approaches. We validate our computational model with several physical prototypes that show excellent agreement with the optimized digital models.
References:
1. Eugene L Allgower and Kurt Georg. 2012. Numerical continuation methods: an introduction. Vol. 13. Springer Science & Business Media.Google Scholar
2. Artelys. 2019. Artelys Knitro – Nonlinear optimization solver. (2019). https://www.artelys.com/en/optimization-tools/knitroGoogle Scholar
3. Marco Attene, Marco Livesu, Sylvain Lefebvre, Thomas Funkhouser, Stefano Ellero, Szymon Rusinkiewicz, Jonàs Martínez, and Amit Haim Bermano. 2018. Design, Representations, and Processing for Additive Manufacturing. Vol. 10. Morgan & Claypool Publishers. 146 pages. https://hal.inria.fr/hal-01836525 Google ScholarDigital Library
4. Moritz Bächer, Stelian Coros, and Bernhard Thomaszewski. 2015. LinkEdit: Interactive Linkage Editing Using Symbolic Kinematics. ACM Trans. Graph. 34, 4, Article 99 (July 2015), 8 pages. Google ScholarDigital Library
5. Changyeob Baek, Andrew O. Sageman-Furnas, Mohammad K. Jawed, and Pedro M. Reis. 2017. Form finding in elastic gridshells. Proceedings of the National Academy of Sciences (2017). arXiv:http://www.pnas.org/content/early/2017/12/13/1713841115.full.pdfGoogle Scholar
6. Miklós Bergou, Basile Audoly, Etienne Vouga, Max Wardetzky, and Eitan Grinspun. 2010. Discrete Viscous Threads. ACM Trans. Graph. 29, 4, Article 116 (July 2010), 10 pages. Google ScholarDigital Library
7. Miklós Bergou, Max Wardetzky, Stephen Robinson, Basile Audoly, and Eitan Grinspun. 2008. Discrete Elastic Rods. ACM Trans. Graph. 27, 3, Article 63 (Aug. 2008), 12 pages. Google ScholarDigital Library
8. Amit H. Bermano, Thomas Funkhouser, and Szymon Rusinkiewicz. 2017. State of the Art in Methods and Representations for Fabrication-Aware Design. Comput. Graph. Forum 36, 2 (May 2017), 509–535. Google ScholarDigital Library
9. Florence Bertails, Basile Audoly, Marie-Paule Cani, Bernard Querleux, Frédéric Leroy, and Jean-Luc Lévêque. 2006. Super-helices for Predicting the Dynamics of Natural Hair. ACM Trans. Graph. 25, 3 (July 2006), 1180–1187. Google ScholarDigital Library
10. Bernd Bickel, Paolo Cignoni, Luigi Malomo, and Nico Pietroni. 2018. State of the Art on Stylized Fabrication. Computer Graphics Forum (2018).Google Scholar
11. Emmanuel J Candes, Michael B Wakin, and Stephen P Boyd. 2008. Enhancing sparsity by reweighted ℓ1 minimization. Journal of Fourier analysis and applications 14, 5–6 (2008), 877–905.Google ScholarCross Ref
12. Jean-François Caron, Olivier Baverel, Frédéric Tayeb, and Lionel Du Peloux. 2012. Gridshells in composite materials: Construction of a 300 m(2) Forum for the Solidays’ Festival in Paris. Physical Review E: Statistical, Nonlinear, and Soft Matter Physics 22, 3 (2012), 408–414. https://hal-enpc.archives-ouvertes.fr/hal-00799095Google Scholar
13. Paolo Celli, Connor McMahan, Brian Ramirez, Anton Bauhofer, Christina Naify, Douglas Hofmann, Basile Audoly, and Chiara Daraio. 2018. Shape-morphing architected sheets with non-periodic cut patterns. Soft matter (2018).Google Scholar
14. Duygu Ceylan, Wilmot Li, Niloy J. Mitra, Maneesh Agrawala, and Mark Pauly. 2013. Designing and Fabricating Mechanical Automata from Mocap Sequences. ACM Trans. Graph. 32, 6, Article 186 (Nov. 2013), 11 pages. Google ScholarDigital Library
15. Will Chang, Hao Li, Niloy Mitra, Mark Pauly, and Michael Wand. 2012. Dynamic Geometry Processing. In Eurographics 2012 – Tutorials. The Eurographics Association.Google Scholar
16. R. Chartrand. 2007. Exact Reconstruction of Sparse Signals via Nonconvex Minimization. IEEE Signal Processing Letters 14, 10 (Oct 2007), 707–710.Google ScholarCross Ref
17. Xiang Chen, Changxi Zheng, Weiwei Xu, and Kun Zhou. 2014. An Asymptotic Numerical Method for Inverse Elastic Shape Design. ACM Trans. Graph. 33, 4, Article 95 (July 2014), 11 pages. Google ScholarDigital Library
18. Yanqing Chen, Timothy A Davis, William W Hager, and Sivasankaran Rajamanickam. 2008. Algorithm 887: CHOLMOD, supernodal sparse Cholesky factorization and update/downdate. ACM Transactions on Mathematical Software (TOMS) 35, 3 (2008), 22. Google ScholarDigital Library
19. Lionel Du Peloux. 2017. Modeling of bending-torsion couplings in active-bending structures. Application to the design of elastic gridshells. Ph.D. Dissertation. Université Paris Est, École des Ponts Paris Tech.Google Scholar
20. Mingbin Feng, John E Mitchell, Jong-Shi Pang, Xin Shen, and Andreas Wächter. 2013. Complementarity formulations of ℓ0-norm optimization problems. Industrial Engineering and Management Sciences. Technical Report. Northwestern University, Evanston, IL, USA (2013).Google Scholar
21. Akash Garg, Andrew O. Sageman-Furnas, Bailin Deng, Yonghao Yue, Eitan Grinspun, Mark Pauly, and Max Wardetzky. 2014. Wire Mesh Design. ACM Trans. Graph. 33, 4, Article 66 (July 2014), 12 pages. Google ScholarDigital Library
22. Philip E Gill, Walter Murray, and Margaret H Wright. 1981. Practical optimization. (1981).Google Scholar
23. Ruslan Guseinov, Eder Miguel, and Bernd Bickel. 2017. CurveUps: Shaping Objects from Flat Plates with Tension-actuated Curvature. ACM Trans. Graph. 36, 4, Article 64 (July 2017), 12 pages. Google ScholarDigital Library
24. Elisa Hernández, Stefan Sechelmann, Thilo Rörig, and Christoph Gengnagel. 2012. Topology Optimisation of Regular and Irregular Elastic Gridshells by Means of a Non-linear Variational Method. Advances in Architectural Geometry, 2012.Google Scholar
25. Elisa Lafuente Hernández, Olivier Baverel, and Christoph Gengnagel. 2013. On the Design and Construction of Elastic Gridshells with Irregular Meshes. International Journal of Space Structures 28, 3–4 (2013), 161–174.Google ScholarCross Ref
26. Thomas JR Hughes. 2012. The finite element method: linear static and dynamic finite element analysis. Courier Corporation.Google Scholar
27. Martin Kilian, Aron Monszpart, and Niloy J. Mitra. 2017. String Actuated Curved Folded Surfaces. ACM Trans. Graph. 36, 4, Article 64a (May 2017). Google ScholarDigital Library
28. Mina Konaković, Keenan Crane, Bailin Deng, Sofien Bouaziz, Daniel Piker, and Mark Pauly. 2016. Beyond Developable: Computational Design and Fabrication with Auxetic Materials. ACM Trans. Graph. 35, 4, Article 89 (July 2016), 11 pages. Google ScholarDigital Library
29. Mina Konaković-Luković, Pavle Konaković, and Mark Pauly. 2018a. Computational Design of Deployable Auxetic Shells. In Advances in Architectural Geometry 2018. 94–111.Google Scholar
30. Mina Konaković-Luković, Julian Panetta, Keenan Crane, and Mark Pauly. 2018b. Rapid Deployment of Curved Surfaces via Programmable Auxetics. ACM Trans. Graph. (Aug. 2018). Google ScholarDigital Library
31. G. Kreisselmeier and R. Steinhauser. 1979. Systematic Control Design by Optimizing a Vector Performance Index. IFAC Proceedings Volumes 12, 7 (1979), 113 — 117.Google Scholar
32. L.D. Landau, E.M. Lifshitz, and J.B. Sykes. 1989. Theory of Elasticity. Pergamon Press. https://books.google.ch/books?id=YjDiQwAACAAJGoogle Scholar
33. Ian Liddell. 2015. Frei Otto and the development of gridshells. Case Studies in Structural Engineering 4 (2015), 39 — 49.Google ScholarCross Ref
34. J. Lienhard. 2014. Bending-Active Structures: Form-finding Strategies Using Elastic Deformation in Static and Kinetic Systems and the Structural Potentials Therein. Universität Stuttgart Inst. f. Tragkonstr.Google Scholar
35. Alessandro Liuti, Alberto Pugnale, and Sofia Colabella. 2017. The Airshell prototype: a timber gridshell erected through a pneumatic formwork. (09 2017).Google Scholar
36. Luigi Malomo, Jesús Peréz, Emmanuel Iarussi, Nico Pietroni, Eder Miguel, Paolo Cignoni, and Bernd Bickel. 2018. FlexMaps: Computational Design of Flat Flexible Shells for Shaping 3D Objects. ACM Trans. on Graphics – Siggraph Asia 2018 37, 6 (dec 2018), 14. Google ScholarDigital Library
37. JRRA Martins and Nicholas MK Poon. 2005. On structural optimization using constraint aggregation. In VI World Congress on Structural and Multidisciplinary Optimization WCSMO6, Rio de Janeiro, Brasil.Google Scholar
38. Romain Mesnil. 2013. Stability of elastic gridshells. Ph.D. Dissertation.Google Scholar
39. Romain Mesnil, Cyril Douthe, and Olivier Baverel. 2017. Non-standard patterns for gridshells: fabrication and structural optimization. 4 (12 2017), 277–286.Google Scholar
40. Jorge Nocedal and Stephen J. Wright. 2006. Numerical Optimization (second ed.). Springer, New York, NY, USA.Google Scholar
41. Dinesh K. Pai. 2002. STRANDS: Interactive Simulation of Thin Solids using Cosserat Models. Computer Graphics Forum (2002).Google Scholar
42. Jesús Pérez, Miguel A. Otaduy, and Bernhard Thomaszewski. 2017. Computational Design and Automated Fabrication of Kirchhoff-plateau Surfaces. ACM Trans. Graph. 36, 4, Article 62 (July 2017), 12 pages. Google ScholarDigital Library
43. Jesús Pérez, Bernhard Thomaszewski, Stelian Coros, Bernd Bickel, José A. Canabal, Robert Sumner, and Miguel A. Otaduy. 2015. Design and Fabrication of Flexible Rod Meshes. ACM Trans. Graph. 34, 4, Article 138 (July 2015), 12 pages. Google ScholarDigital Library
44. Nico Pietroni, Davide Tonelli, Enrico Puppo, Maurizio Froli, Roberto Scopigno, and Paolo Cignoni. 2015. Statics Aware Grid Shells. Comput. Graph. Forum 34, 2 (May 2015), 627–641. Google ScholarDigital Library
45. Helmut Pottmann, Michael Eigensatz, Amir Vaxman, and Johannes Wallner. 2015. Architectural Geometry. Computers and Graphics 47 (2015), 145–164. Google ScholarDigital Library
46. G Quinn and C Gengnagel. 2014. A review of elastic grid shells, their erection methods and the potential use of pneumatic formwork. Mob Rapidly Assem Struct IV 136 (2014), 129–143.Google ScholarCross Ref
47. Christian Schüller, Roi Poranne, and Olga Sorkine-Hornung. 2018. Shape Representation by Zippables. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH) 37, 4 (Aug. 2018). Google ScholarDigital Library
48. Christian Schumacher, Steve Marschner, Markus Cross, and Bernhard Thomaszewski. 2018. Mechanical Characterization of Structured Sheet Materials. ACM Trans. Graph. 37, 4, Article 148 (July 2018), 15 pages. Google ScholarDigital Library
49. Jonathan Richard Shewchuk. 1996. Triangle: Engineering a 2D Quality Mesh Generator and Delaunay Triangulator. In WACG (Lecture Notes in Computer Science), Vol. 1148. Springer, 203–222. Google ScholarDigital Library
50. Ole Sigmund. 2001. A 99 line topology optimization code written in Matlab. Structural and multidisciplinary optimization 21, 2 (2001), 120–127. Google ScholarDigital Library
51. J. Spillmann and M. Teschner. 2007. CORDE: Cosserat Rod Elements for the Dynamic Simulation of One-Dimensional Elastic Objects. In Eurographics/SIGGRAPH Symposium on Computer Animation. Google ScholarDigital Library
52. Tomohiro Tachi. 2013. Composite Rigid-Foldable Curved Origami Structure.Google Scholar
53. Chengcheng Tang, Xiang Sun, Alexandra Gomes, Johannes Wallner, and Helmut Pottmann. 2014. Form-finding with Polyhedral Meshes Made Simple. ACM Trans. Graph. 33, 4, Article 70 (July 2014), 9 pages. Google ScholarDigital Library
54. Frédéric Tayeb, Jean-François Caron, Olivier Baverel, and Lionel Du Peloux. 2013. Stability and robustness of a 300 m2 Composite Gridshell Structure. Construction and Building Materials (2013).Google Scholar
55. Bernhard Thomaszewski, Stelian Coros, Damien Gauge, Vittorio Megaro, Eitan Grinspun, and Markus Gross. 2014. Computational Design of Linkage-based Characters. ACM Trans. Graph. 33, 4, Article 64 (July 2014), 9 pages. Google ScholarDigital Library
56. Davide Tonelli, Nico Pietroni, Paolo Cignoni, and Roberto Scopigno. 2016. Design and Fabrication of Grid-shells Mockups. In Proceedings of the Conference on Smart Tools and Applications in Computer Graphics (STAG ’16). 21–27. Google ScholarDigital Library
57. Nobuyuki Umetani, Ryan Schmidt, and Jos Stam. 2014. Position-based Elastic Rods. In ACM SIGGRAPH 2014 Talks (SIGGRAPH ’14). ACM, New York, NY, USA, Article 47, 1 pages. Google ScholarDigital Library
58. Jonas Zehnder, Stelian Coros, and Bernhard Thomaszewski. 2016. Designing Structurally-sound Ornamental Curve Networks. ACM Trans. Graph. 35, 4, Article 99 (July 2016), 10 pages. Google ScholarDigital Library
59. Changxi Zheng, Timothy Sun, and Xiang Chen. 2016. Deployable 3D Linkages with Collision Avoidance. In Proceedings of the ACM SIGGRAPH/Eurographics Symposium on Computer Animation (SCA ’16). 179–188. http://dl.acm.org/citation.cfm?id=2982818.2982843 Google ScholarDigital Library