“Weaving geodesic foliations” by Vekhter, Zhuo, Fandino, Huang and Vouga
Conference:
Type(s):
Title:
- Weaving geodesic foliations
Session/Category Title: Shape Science
Presenter(s)/Author(s):
Abstract:
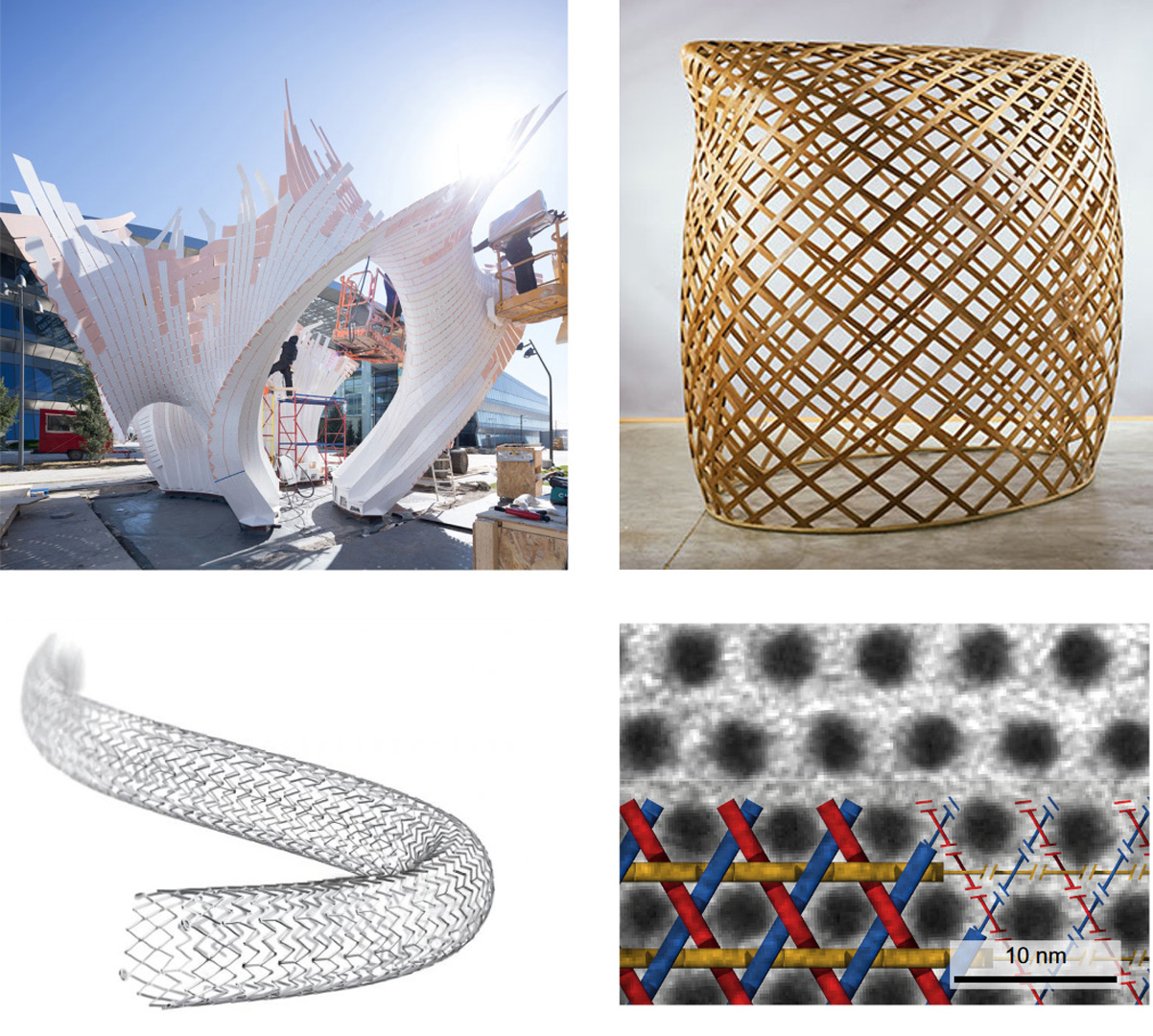
We study discrete geodesic foliations of surfaces—foliations whose leaves are all approximately geodesic curves—and develop several new variational algorithms for computing such foliations. Our key insight is a relaxation of vector field integrability in the discrete setting, which allows us to optimize for curl-free unit vector fields that remain well-defined near singularities and robustly recover a scalar function whose gradient is well aligned to these fields. We then connect the physics governing surfaces woven out of thin ribbons to the geometry of geodesic foliations, and present a design and fabrication pipeline for approximating surfaces of arbitrary geometry and topology by triaxially-woven structures, where the ribbon layout is determined by a geodesic foliation on a sixfold branched cover of the input surface. We validate the effectiveness of our pipeline on a variety of simulated and fabricated woven designs, including an example for readers to try at home.
References:
1. Hillel Aharoni, Desislava V. Todorova, Octavio Albarrán, Lucas Goehring, Randall D. Kamien, and Eleni Katifori. 2017. The smectic order of wrinkles. In Nature communications.Google Scholar
2. Ergun Akleman, Jianer Chen, YenLin Chen, Qing Xing, and Jonathan L. Gross. 2011. Cyclic twill-woven objects. Computers & Graphics 35, 3 (2011), 623 — 631. Shape Modeling International (SMI) Conference 2011. Google ScholarDigital Library
3. Ergun Akleman, Jianer Chen, Qing Xing, and Jonathan L. Gross. 2009. Cyclic Plain-weaving on Polygonal Mesh Surfaces with Graph Rotation Systems. In ACM SIGGRAPH 2009 Papers (SIGGRAPH ’09). ACM, New York, NY, USA, Article 78, 8 pages. Google ScholarDigital Library
4. Marc Alexa and Max Wardetzky. 2011. Discrete Laplacians on General Polygonal Meshes. ACM Trans. Graph. 30, 4, Article 102 (July 2011), 10 pages. Google ScholarDigital Library
5. Patricio Aviles and Yoshikazu Giga. 1987. A mathematical problem related to the physical theory of liquid crystal configurations. In Miniconference on geometry/partial differential equations, 2. Centre for Mathematical Analysis, The Australian National University, Canberra AUS, 1–16. https://projecteuclid.org/euclid.pcma/1416336633Google Scholar
6. Phil Ayres, Alison Grace Martin, and Mateusz Zwierzycki. 2018. Beyond the basket case: A principled approach to the modelling of kagome weave patterns for the fabrication of interlaced lattice structures using straight strips. In Advances in Architectural Geometry.Google Scholar
7. Omri Azencot, Maks Ovsjanikov, Frédéric Chazal, and Mirela Ben-Chen. 2015. Discrete Derivatives of Vector Fields on Surfaces – An Operator Approach. ACM Trans. Graph. 34, 3, Article 29 (May 2015), 13 pages. Google ScholarDigital Library
8. Mirela Ben-Chen, Adrian Butscher, Justin Solomon, and Leonidas Guibas. 2010. On Discrete Killing Vector Fields and Patterns on Surfaces. Computer Graphics Forum 29, 5 (2010), 1701–1711.Google ScholarCross Ref
9. Miklós Bergou, Basile Audoly, Etienne Vouga, Max Wardetzky, and Eitan Grinspun. 2010. Discrete Viscous Threads. ACM Trans. Graph. 29, 4, Article 116 (July 2010), 10 pages. Google ScholarDigital Library
10. Miklós Bergou, Max Wardetzky, Stephen Robinson, Basile Audoly, and Eitan Grinspun. 2008. Discrete Elastic Rods. ACM Trans. Graph. 27, 3, Article 63 (Aug. 2008), 12 pages. Google ScholarDigital Library
11. Florence Bertails, Basile Audoly, Marie-Paule Cani, Bernard Querleux, Frédéric Leroy, and Jean-Luc Lévêque. 2006. Super-helices for Predicting the Dynamics of Natural Hair. In ACM SIGGRAPH 2006 Papers (SIGGRAPH ’06). ACM, New York, NY, USA, 1180–1187. Google ScholarDigital Library
12. H. Bhatia, S. Jadhav, P. Bremer, G. Chen, J. A. Levine, L. G. Nonato, and V. Pascucci. 2011. Edge maps: Representing flow with bounded error. In 2011 IEEE Pacific Visualization Symposium. IEEE, Hong Kong, China, 75–82. Google ScholarDigital Library
13. E Boeckx and L Vanhecke. 2000. Harmonic and minimal vector fields on tangent and unit tangent bundles. Differential Geometry and its Applications 13 (07 2000), 77–93.Google Scholar
14. David Bommes, Bruno Lévy, Nico Pietroni, Enrico Puppo, Claudio Silva, Marco Tarini, and Denis Zorin. 2013. Quad-Mesh Generation and Processing: A Survey. Comput. Graph. Forum 32, 6 (Sept. 2013), 51–76. Google ScholarDigital Library
15. David Bommes, Henrik Zimmer, and Leif Kobbelt. 2009. Mixed-integer Quadrangulation. ACM Trans. Graph. 28, 3, Article 77 (July 2009), 10 pages. Google ScholarDigital Library
16. David Bommes, Henrik Zimmer, and Leif Kobbelt. 2012. Practical Mixed-integer Optimization for Geometry Processing. In Proceedings of the 7th International Conference on Curves and Surfaces. Springer-Verlag, Berlin, Heidelberg, 193–206. Google ScholarDigital Library
17. Boston Scientific. 2015. Innova: Self-expanding nitinol stent system for Superficial Femoral Arterty. http://www.bostonscientific.com/en-EU/products/stents-vascular/innova-self-expanding-stent-system.html. Accessed: 2018-05-27.Google Scholar
18. J. B. Boyling. 1968. Carathéodory’s principle and the existence of global integrating factors. Communications in Mathematical Physics 10, 1 (01 Feb 1968), 52–68.Google Scholar
19. P.-T. Brun, Basile Audoly, Neil M. Ribe, T. S. Eaves, and John R. Lister. 2015. Liquid Ropes: A Geometrical Model for Thin Viscous Jet Instabilities. Phys. Rev. Lett. 114 (Apr 2015), 174501. Issue 17.Google ScholarCross Ref
20. Marcel Campen, David Bommes, and Leif Kobbelt. 2012. Dual Loops Meshing: Quality Quad Layouts on Manifolds. ACM Trans. Graph. 31, 4, Article 110 (July 2012), 11 pages. Google ScholarDigital Library
21. Marcel Campen, Moritz Ibing, Hans-Christian Ebke, Denis Zorin, and Leif Kobbelt. 2016. Scale-Invariant Directional Alignment of Surface Parametrizations. Computer Graphics Forum 35, 5 (2016), 1–10.Google ScholarDigital Library
22. Marcel Campen and Leif Kobbelt. 2014a. Dual Strip Weaving: Interactive Design of Quad Layouts Using Elastica Strips. ACM Trans. Graph. 33, 6, Article 183 (Nov. 2014), 10 pages. Google ScholarDigital Library
23. M. Campen and L. Kobbelt. 2014b. Quad Layout Embedding via Aligned Parameterization. Comput. Graph. Forum 33, 8 (Dec. 2014), 69–81. Google ScholarDigital Library
24. Paolo Cignoni, Nico Pietroni, Luigi Malomo, and Roberto Scopigno. 2014. Field-aligned Mesh Joinery. ACM Trans. Graph. 33, 1, Article 11 (Feb. 2014), 12 pages. Google ScholarDigital Library
25. Gabriel Cirio, Jorge Lopez-Moreno, David Miraut, and Miguel A. Otaduy. 2014. Yarn-level Simulation of Woven Cloth. ACM Trans. Graph. 33, 6, Article 207 (Nov. 2014), 11 pages. Google ScholarDigital Library
26. Keenan Crane, Mathieu Desbrun, and Peter Schröder. 2010. Trivial Connections on Discrete Surfaces. Computer Graphics Forum 29, 5 (2010), 1525–1533.Google ScholarCross Ref
27. Keenan Crane, Clarisse Weischedel, and Max Wardetzky. 2013. Geodesics in Heat: A New Approach to Computing Distance Based on Heat Flow. ACM Trans. Graph. 32, 5, Article 152 (Oct. 2013), 11 pages. Google ScholarDigital Library
28. Fernando de Goes, Mathieu Desbrun, and Yiying Tong. 2016. Vector Field Processing on Triangle Meshes. In ACM SIGGRAPH 2016 Courses (SIGGRAPH ’16). ACM, New York, NY, USA, Article 27, 49 pages. Google ScholarDigital Library
29. Olga Diamanti, Amir Vaxman, Daniele Panozzo, and Olga Sorkine-Hornung. 2014. Designing N-PolyVector Fields with Complex Polynomials. Comput. Graph. Forum 33, 5 (Aug. 2014), 1–11. Google ScholarDigital Library
30. Olga Diamanti, Amir Vaxman, Daniele Panozzo, and Olga Sorkine-Hornung. 2015. Integrable PolyVector Fields. ACM Trans. Graph. 34, 4, Article 38 (July 2015), 12 pages. Google ScholarDigital Library
31. N. M. Ercolani and S. C. Venkataramani. 2009. A Variational Theory for Point Defects in Patterns. Journal of Nonlinear Science 19, 3 (01 Jun 2009), 267–300.Google ScholarCross Ref
32. Nahum Farchi and Mirela Ben-Chen. 2018. Integer-only Cross Field Computation. ACM Trans. Graph. 37, 4, Article 91 (July 2018), 13 pages. Google ScholarDigital Library
33. Matthew Fisher, Peter Schröder, Mathieu Desbrun, and Hugues Hoppe. 2007. Design of Tangent Vector Fields. ACM Trans. Graph. 26, 3 (July 2007), 1–9. Google ScholarDigital Library
34. Marc Fornes. 2017. Minima | Maxima. World Expo 2017.Google Scholar
35. Akash Garg, Andrew O. Sageman-Furnas, Bailin Deng, Yonghao Yue, Eitan Grinspun, Mark Pauly, and Max Wardetzky. 2014. Wire Mesh Design. ACM Trans. Graph. 33, 4, Article 66 (July 2014), 12 pages. Google ScholarDigital Library
36. Michel X. Goemans and David P. Williamson. 1995. Improved Approximation Algorithms for Maximum Cut and Satisfiability Problems Using Semidefinite Programming. J. ACM 42, 6 (Nov. 1995), 1115–1145. Google ScholarDigital Library
37. Aaron Hertzmann and Denis Zorin. 2000. Illustrating Smooth Surfaces. In Proceedings of the 27th Annual Conference on Computer Graphics and Interactive Techniques (SIGGRAPH ’00). ACM Press/Addison-Wesley Publishing Co., New York, NY, USA, 517–526. Google ScholarDigital Library
38. W. S. Howard and V. Kumar. 1993. A minimum principle for the dynamic analysis of systems with frictional contacts. In {1993} Proceedings IEEE International Conference on Robotics and Automation. IEEE, Atlanta, GA, 437–442 vol.3.Google Scholar
39. Yuki Igarashi, Takeo Igarashi, and Hiromasa Suzuki 2008 Knitting a 3D Model. Comput. Graph. Forum 27 (10 2008), 1737–1743.Google Scholar
40. Pierre-Emmanuel Jabin, Felix Otto, and Benoî t Perthame. 2002. Line-energy Ginzburg-Landau models : zero-energy states. Annali della Scuola Normale Superiore di Pisa – Classe di Scienze Ser. 5, 1, 1 (2002), 187–202.Google Scholar
41. Wenzel Jakob, Marco Tarini, Daniele Panozzo, and Olga Sorkine-Hornung. 2015. Instant Field-aligned Meshes. ACM Trans. Graph. 34, 6, Article 189 (Oct. 2015), 15 pages. Google ScholarDigital Library
42. Felix Kälberer, Matthias Nieser, and Konrad Polthier. 2007. QuadCover – Surface Parameterization using Branched Coverings. Comput. Graph. Forum 26 (09 2007), 375–384.Google Scholar
43. Jonathan M. Kaldor, Doug L. James, and Steve Marschner. 2008. Simulating Knitted Cloth at the Yarn Level. In ACM SIGGRAPH 2008 Papers (SIGGRAPH ’08). ACM, New York, NY, USA, Article 65, 9 pages. Google ScholarDigital Library
44. W. Klingenberg. 1978. Lectures on Closed Geodesies. Springer-Verlag. https://books.google.com/books?id=t1nvAAAAMAAJGoogle Scholar
45. Felix Knöppel, Keenan Crane, Ulrich Pinkall, and Peter Schröder. 2013. Globally Optimal Direction Fields. ACM Trans. Graph. 32, 4, Article 59 (July 2013), 10 pages. Google ScholarDigital Library
46. Felix Knöppel, Keenan Crane, Ulrich Pinkall, and Peter Schröder. 2015. Stripe Patterns on Surfaces. ACM Trans. Graph. 34 (2015), 1–11. Issue 4. Google ScholarDigital Library
47. Robert Kohn. 2006. Energy-driven pattern formation. In International Congress of Mathematicians, ICM 2006, Vol. 1. 359–383.Google Scholar
48. Jonathan Leaf, Rundong Wu, Eston Schweickart, Doug L. James, and Steve Marschner. 2018. Interactive Design of Periodic Yarn-level Cloth Patterns. ACM Trans. Graph. 37, 6, Article 202 (Dec. 2018), 202:1–202:15 pages. Google ScholarDigital Library
49. Urszula Lewandowska, Wojciech Zajaczkowski, Stefano Corra, Junki Tanabe, Ruediger Borrmann, Edmondo M. Benetti, Sebastian Stappert, Kohei Watanabe, Nellie A. K. Ochs, Robin Schaeublin, Chen Li, Eiji Yashima, Wojciech Pisula, Klaus Müllen, and Helma Wennemers. 2017. A triaxial supramolecular weave. Nature Chemistry 9 (24 Jul 2017), 1068 EP -. Article.Google Scholar
50. Binbin Lin, Xiaofei He, Chiyuan Zhang, and Ming Ji. 2013. Parallel Vector Field Embedding. Journal of Machine Learning Research 14 (2013), 2945–2977. Google ScholarDigital Library
51. Beibei Liu, Yiying Tong, Fernando De Goes, and Mathieu Desbrun. 2016. Discrete Connection and Covariant Derivative for Vector Field Analysis and Design. ACM Trans. Graph. 35, 3, Article 23 (March 2016), 17 pages. Google ScholarDigital Library
52. Thomas Machon, Hillel Aharoni, Yichen Hu, and Randall D. Kamien. 2019. Aspects of Defect Topology in Smectic Liquid Crystals. Communications in Mathematical Physics (20 Feb 2019).Google Scholar
53. Alison Martin. 2018. Alison Grace Martin. http://gallery.bridgesmathart.org/exhibitions/2013-bridges-conference/alison-martin. Accessed: 2018-01-23.Google Scholar
54. James McCann, Lea Albaugh, Vidya Narayanan, April Grow, Wojciech Matusik, Jennifer Mankoff, and Jessica Hodgins. 2016. A Compiler for 3D Machine Knitting. ACM Trans. Graph. 35, 4, Article 49 (July 2016), 11 pages. Google ScholarDigital Library
55. Eder Miguel, Mathias Lepoutre, and Bernd Bickel. 2016. Computational Design of Stable Planar-rod Structures. ACM Trans. Graph. 35, 4, Article 86 (July 2016), 11 pages. Google ScholarDigital Library
56. Joseph S. B. Mitchell, David M. Mount, and Christos H. Papadimitriou. 1987. The Discrete Geodesic Problem. SIAM J. Comput. 16, 4 (Aug. 1987), 647–668. Google ScholarDigital Library
57. Vidya Narayanan, Lea Albaugh, Jessica Hodgins, Stelian Coros, and James Mccann. 2018. Automatic Machine Knitting of 3D Meshes. ACM Trans. Graph. 37, 3, Article 35 (Aug. 2018), 15 pages. Google ScholarDigital Library
58. M. Nieser, J. Palacios, K. Polthier, and E. Zhang. 2012. Hexagonal Global Parameterization of Arbitrary Surfaces. IEEE Transactions on Visualization and Computer Graphics 18, 6 (June 2012), 865–878. Google ScholarDigital Library
59. Jonathan Palacios and Eugene Zhang. 2007. Rotational Symmetry Field Design on Surfaces. ACM Trans. Graph. 26, 3, Article 55 (July 2007), 10 pages. Google ScholarDigital Library
60. Daniele Panozzo, Enrico Puppo, Marco Tarini, and Olga Sorkine-Hornung. 2014. Frame Fields: Anisotropic and Non-orthogonal Cross Fields. ACM Trans. Graph. 33, 4, Article 134 (July 2014), 11 pages. Google ScholarDigital Library
61. Jesús Pérez, Bernhard Thomaszewski, Stelian Coros, Bernd Bickel, José A. Canabal, Robert Sumner, and Miguel A. Otaduy. 2015. Design and Fabrication of Flexible Rod Meshes. ACM Trans. Graph. 34, 4, Article 138 (July 2015), 12 pages. Google ScholarDigital Library
62. Henri Poincare. 1905. Sur Les Lignes Geodesiques Des Surfaces Convexes. Trans. Amer. Math. Soc. 6, 3 (1905), 237–274. http://www.jstor.org/stable/1986219Google ScholarCross Ref
63. S. Poljak and Z. Tuza. 1994. The Max-cut Problem: A Survey. Institute of Mathematics, Academia Sinica, Taipei, Taiwan, China. https://books.google.com/books?id=-AaZMwEACAAJGoogle Scholar
64. Konrad Polthier and Eike Preuß. 2003. Identifying Vector Field Singularities Using a Discrete Hodge Decomposition. In Visualization and Mathematics III, Hans-Christian Hege and Konrad Polthier (Eds.). Springer Berlin Heidelberg, Berlin, Heidelberg, 113–134.Google Scholar
65. Konrad Polthier and Markus Schmies. 2006. Straightest Geodesics on Polyhedral Surfaces. In ACM SIGGRAPH 2006 Courses (SIGGRAPH ’06). ACM, New York, NY, USA, 30–38. Google ScholarDigital Library
66. Mariana Popescu, Matthias Rippmann, Tom Van Mele, and Philippe Block. 2018. Automated Generation of Knit Patterns for Non-developable Surfaces. Springer Singapore, Singapore, 271–284.Google Scholar
67. Helmut Pottmann, Qixing Huang, Bailin Deng, Alexander Schiftner, Martin Kilian, Leonidas Guibas, and Johannes Wallner. 2010. Geodesic Patterns. ACM Trans. Graph. 29, 4, Article 43 (July 2010), 10 pages. Google ScholarDigital Library
68. Martin Puryear. 1998. Brunhilde. Cedar and rattan.Google Scholar
69. Qmechanic. 2018. Is every vector field locally integrable up to a rescaling? Mathematics Stack Exchange. URL:https://math.stackexchange.com/q/2716593 (version: 2018-04-10).Google Scholar
70. Nicolas Ray, Wan Chiu Li, Bruno Lévy, Alla Sheffer, and Pierre Alliez. 2006. Periodic Global Parameterization. ACM Trans. Graph. 25, 4 (Oct. 2006), 1460–1485. Google ScholarDigital Library
71. Nicolas Ray and Dmitry Sokolov. 2014. Robust Polylines Tracing for N-Symmetry Direction Field on Triangulated Surfaces. ACM Trans. Graph. 33, 3, Article 30 (June 2014), 11 pages. Google ScholarDigital Library
72. Nicolas Ray, Bruno Vallet, Laurent Alonso, and Bruno Levy. 2009. Geometry-aware Direction Field Processing. ACM Trans. Graph. 29, 1, Article 1 (Dec. 2009), 11 pages. Google ScholarDigital Library
73. Tristan Rivière and Sylvia Serfaty. 2001. Limiting domain wall energy for a problem related to micromagnetics. Communications on Pure and Applied Mathematics 54, 3 (2001), 294–338. <294::AID-CPA2>3.0.CO;2-SarXiv:https://onlinelibrary.wiley.com/doi/pdf/10.1002/1097-0312%28200103%2954%3A3%3C294%3A%3AAID-CPA2%3E3.0.CO%3B2-SGoogle ScholarCross Ref
74. Lawrence Roy, Prashant Kumar, Sanaz Golbabaei, Yue Zhang, and Eugene Zhang. 2018. Interactive Design and Visualization of Branched Covering Spaces. IEEE Trans. Vis. Comput. Graph. 24, 1 (2018), 843–852.Google ScholarCross Ref
75. Zhongwei Shen, Jin Huang, Wei Chen, and Hujun Bao. 2015. Geometrically Exact Simulation of Inextensible Ribbon. Comput. Graph. Forum 34, 7 (Oct. 2015), 145–154. Google ScholarDigital Library
76. Mélina Skouras, Stelian Coros, Eitan Grinspun, and Bernhard Thomaszewski. 2015. Interactive Surface Design with Interlocking Elements. ACM Trans. Graph. 34, 6, Article 224 (Oct. 2015), 224:1–224:7 pages. Google ScholarDigital Library
77. Peng Song, Chi-Wing Fu, Prashant Goswami, Jianmin Zheng, Niloy J. Mitra, and Daniel Cohen-Or. 2013. Reciprocal Frame Structures Made Easy. ACM Trans. Graph. 32, 4, Article 94 (July 2013), 13 pages. Google ScholarDigital Library
78. Vitaly Surazhsky, Tatiana Surazhsky, Danil Kirsanov, Steven J. Gortler, and Hugues Hoppe. 2005. Fast Exact and Approximate Geodesics on Meshes. ACM Trans. Graph. 24, 3 (July 2005), 553–560. Google ScholarDigital Library
79. Masahito Takezawa, Takuma Imai, Kentaro Shida, and Takashi Maekawa. 2016. Fabrication of Freeform Objects by Principal Strips. ACM Trans. Graph. 35, 6, Article 225 (Nov. 2016), 12 pages. Google ScholarDigital Library
80. Ye Tao, Nannan Lu, Caowei Zhang, Guanyun Wang, Cheng Yao, and Fangtian Ying. 2016. CompuWoven: A Computer-Aided Fabrication Approach to Hand-Woven Craft. In Proceedings of the 2016 CHI Conference Extended Abstracts on Human Factors in Computing Systems (CHIEA ’16). ACM, New York, NY, USA, 2328–2333. Google ScholarDigital Library
81. Ye Tao, Guanyun Wang, Caowei Zhang, Nannan Lu, Xiaolian Zhang, Cheng Yao, and Fangtian Ying. 2017. WeaveMesh: A Low-Fidelity and Low-Cost Prototyping Approach for 3D Models Created by Flexible Assembly. In Proceedings of the 2017 CHI Conference on Human Factors in Computing Systems (CHI ’17). ACM, New York, NY, USA, 509–518. Google ScholarDigital Library
82. Hugues Vandeparre, Miguel Piñeirua, Fabian Brau, Benoit Roman, José Bico, Cyprien Gay, Wenzhong Bao, Chun Ning Lau, Pedro M. Reis, and Pascal Damman. 2011. Wrinkling Hierarchy in Constrained Thin Sheets from Suspended Graphene to Curtains. Phys. Rev. Lett. 106 (Jun 2011), 224301. Issue 22.Google ScholarCross Ref
83. Amir Vaxman, Marcel Campen, Olga Diamanti, Daniele Panozzo, David Bommes, Klaus Hildebrandt, and Mirela Ben-Chen. 2016. Directional Field Synthesis, Design, and Processing. In Proceedings of the 37th Annual Conference of the European Association for Computer Graphics: State of the Art Reports (EG ’16). Eurographics Association, Goslar Germany, Germany, 545–572. Google ScholarDigital Library
84. R. Viertel and B. Osting. 2019. An Approach to Quad Meshing Based on Harmonic Cross-Valued Maps and the Ginzburg-Landau Theory. SIAM Journal on Scientific Computing 41, 1 (2019), A452–A479.Google ScholarDigital Library
85. Kui Wu, Xifeng Gao, Zachary Ferguson, Daniele Panozzo, and Cem Yuksel. 2018. Stitch Meshing. ACM Trans. Graph. 37, 4 (2018). Google ScholarDigital Library
86. Qing Xing, Gabriel Esquivel, Ergun Akleman, Jianer Chen, and Jonathan Gross. 2011. Band Decomposition of 2-manifold Meshes for Physical Construction of Large Structures. In ACM SIGGRAPH 2011 Posters (SIGGRAPH ’11). ACM, New York, NY, USA, Article 58, 1 pages. Google ScholarDigital Library
87. Alexander Yampolsky. 2005. On extrinsic geometry of unit normal vector fields of Riemannian hyperfoliations. (2005). arXiv:arXiv:math/0503566Google Scholar
88. Cem Yuksel, Jonathan M. Kaldor, Doug L. James, and Steve Marschner. 2012. Stitch Meshes for Modeling Knitted Clothing with Yarn-level Detail. ACM Trans. Graph. 31, 4, Article 37 (July 2012), 12 pages. Google ScholarDigital Library
89. Jonas Zehnder, Stelian Coros, and Bernhard Thomaszewski. 2016. Designing Structurally-sound Ornamental Curve Networks. ACM Trans. Graph. 35, 4, Article 99 (July 2016), 10 pages. Google ScholarDigital Library
90. Eugene Zhang, Konstantin Mischaikow, and Greg Turk. 2006. Vector Field Design on Surfaces. ACM Trans. Graph. 25, 4 (Oct. 2006), 1294–1326. Google ScholarDigital Library
91. Mateusz Zwierzycki, Petras Vestartas, Mary Katherine Heinrich, and Phil Ayres. 2017. High Resolution Representation and Simulation of Braiding Patterns. In Acadia 2017: Disciplines and Disruption, Vol. MA 2–4. Acadia Publishing Company, Boston, MA, 670–679.Google Scholar