“Visual smoothness of polyhedral surfaces” by Pellis, Kilian, Dellinger, Wallner and Pottmann
Conference:
Type(s):
Title:
- Visual smoothness of polyhedral surfaces
Session/Category Title: Shape Science
Presenter(s)/Author(s):
Abstract:
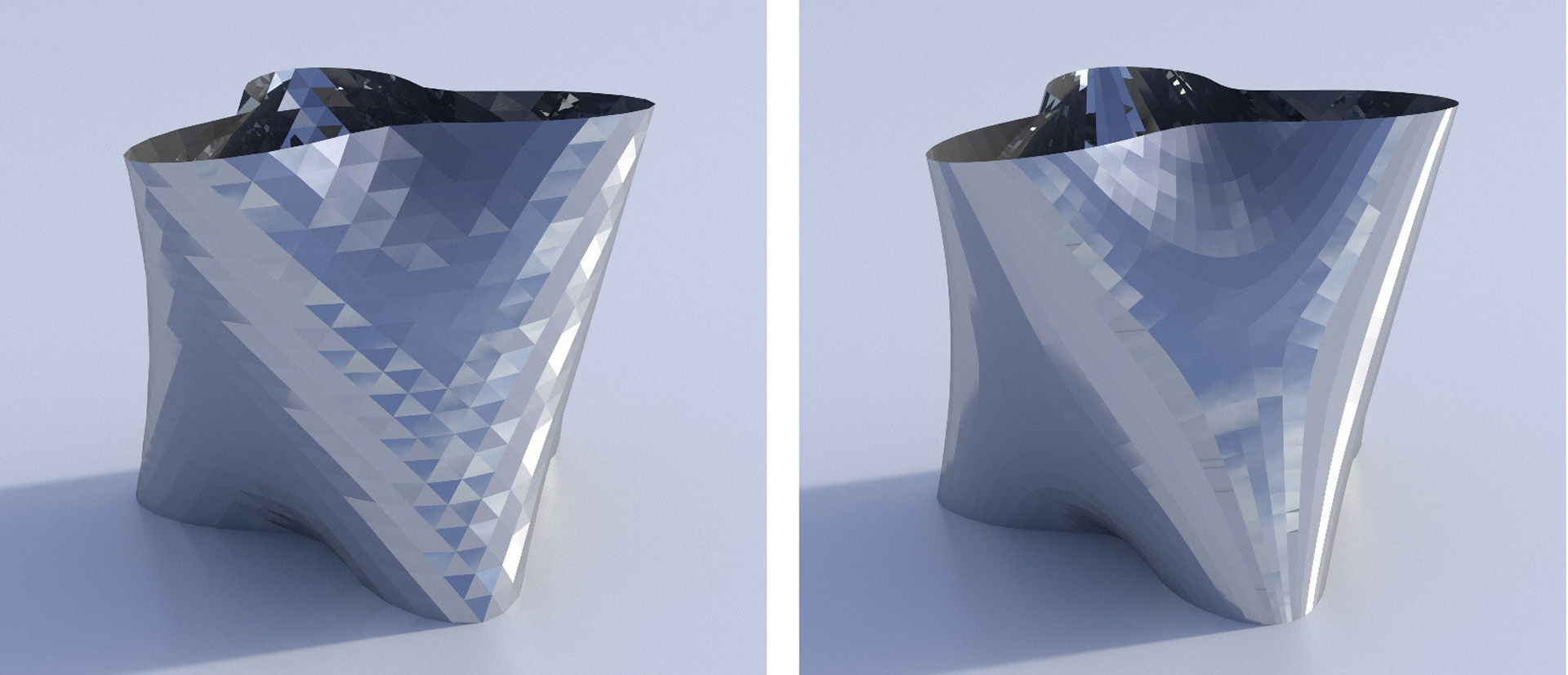
Representing smooth geometric shapes by polyhedral meshes can be quite difficult in situations where the variation of edges and face normals is prominently visible. Especially problematic are saddle-shaped areas of the mesh, where typical vertices with six incident edges are ill suited to emulate the more symmetric smooth situation. The importance of a faithful discrete representation is apparent for certain special applications like freeform architecture, but is also relevant for simulation and geometric computing.In this paper we discuss what exactly is meant by a good representation of saddle points, and how this requirement is stronger than a good approximation of a surface plus its normals. We characterize good saddles in terms of the normal pyramid in a vertex.We show several ways to design meshes whose normals enjoy small variation (implying good saddle points). For this purpose we define a discrete energy of polyhedral surfaces, which is related to a certain total absolute curvature of smooth surfaces. We discuss the minimizers of both functionals and in particular show that the discrete energy is minimal not for triangle meshes, but for principal quad meshes. We demonstrate our procedures for optimization and interactive design by means of meshes intended for architectural design.
References:
1. Lyuba Alboul and Ruud van Damme. 1996. Polyhedral metrics in surface reconstruction. In The Mathematics of Surfaces VI, G. Mullineux (Ed.). Clarendon Press, 171–200. Google ScholarDigital Library
2. Ben Andrews. 1999. Gauss curvature flow: the fate of the rolling stones. Invent. math. 138 (1999), 151–161.Google Scholar
3. Ulrich Bauer, Konrad Polthier, and Max Wardetzky. 2010. Uniform convergence of discrete curvatures from nets of curvature lines. Discr. Comp. Geom. 43 (2010), 798–823. Google ScholarDigital Library
4. Ronny Bergmann, Marc Herrmann, Roland Herzog, Stephan Schmidt, and José Vidal Núñez. 2019. Total variation of the normal vector field as shape prior with applications in geometric inverse problems. preprint. http://arxiv.org/abs/1902.07240.Google Scholar
5. Alexander Bobenko and Yuri Suris. 2009. Discrete differential geometry: Integrable Structure. American Math. Soc.Google Scholar
6. Alexander I. Bobenko and Peter Schröder. 2005. Discrete Willmore flow. In Geometry Processing 2005 (Symposium Proceedings). 101–110. Google ScholarDigital Library
7. David Bommes, Henrik Zimmer, and Leif Kobbelt. 2009. Mixed-integer quadrangulation. ACM Trans. Graph. 28, 3 (2009), 77:1–77:10. Google ScholarDigital Library
8. David Cohen-Steiner and Jean-Marie Morvan. 2003. Restricted Delauney triangulations and normal cycle. In Proc. 19th SoCG. 237–246. Google ScholarDigital Library
9. Manfredo P. do Carmo. 1976. Differential geometry of curves and surfaces. Prentice-Hall.Google Scholar
10. Nira Dyn, Kai Hormann, Sun-Jeong Kim, and David Levin. 2001. Optimizing 3D triangulations using discrete curvature analysis. In Mathematical Methods for Curves and Surfaces: Oslo 2000. Vanderbilt University Press, 135–146. Google ScholarDigital Library
11. Vladimir A. Garanzha. 2010. Discrete extrinsic curvatures and approximation of surfaces by polar polyhedra. Comp. Math. & Math. Physics 50 (2010), 65–92.Google ScholarCross Ref
12. Felix Günther, Caigui Jiang, and Helmut Pottmann. 2017. Smooth polyhedral surfaces. preprint. http://arxiv.org/abs/1703.05318.Google Scholar
13. Klaus Hildebrandt, Konrad Polthier, and Max Wardetzky. 2006. On the convergence of metric and geometric properties of polyhedral surfaces. Geometriae Dedicata 123 (2006), 89–112.Google ScholarCross Ref
14. Gerhard Huisken. 1984. Flow by mean curvature of convex surfaces into spheres. J. Diff. Geometry 20 (1984), 237–266.Google ScholarCross Ref
15. Caigui Jiang, Felix Günther, Johannes Wallner, and Helmut Pottmann. 2016. Measuring and controlling fairness of triangulations. In Advances in Architectural Geometry 2016. vdf Hochschulverlag, Zürich, 24–39.Google Scholar
16. Caigui Jiang, Chengcheng Tang, Amir Vaxman, Peter Wonka, and Helmut Pottmann. 2015. Polyhedral patterns. ACM Trans. Graph. 34, 6 (2015), 172:1–172:12. Google ScholarDigital Library
17. Leif Kobbelt, Swen Campagna, Jens Vorsatz, and Hans-Peter Seidel. 1998. Interactive multi-resolution modeling on arbitrary meshes. In Proc. SIGGRAPH. 105–114. Google ScholarDigital Library
18. David Orden, Günter Rote, Francisco Santos, Brigitte Servatius, Herman Servatius, and Walter Whiteley. 2004. Non-crossing frameworks with non-crossing reciprocals. Discr. Comp. Geom. 32, 4 (2004), 567–600. Google ScholarDigital Library
19. Davide Pellis, Martin Kilian, Johannes Wallner, and Helmut Pottmann. 2017. Material-minimizing forms and structures. ACM Trans. Graphics 36, 6 (2017), 173:1–173:12. Google ScholarDigital Library
20. Helmut Pottmann, Michael Eigensatz, Amir Vaxman, and Johannes Wallner. 2015. Architectural geometry. Computers and Graphics 47 (2015), 145–164. Google ScholarDigital Library
21. Eike Schling, Martin Kilian, Hui Wang, Jonas Schikore, and Helmut Pottmann. 2018. Design and construction of curved support structures with repetitive parameters. In Proc. Advances in Architectural Geometry. Klein Publishing, 140–165.Google Scholar
22. Oded Stein, Eitan Grinspun, and Keenan Crane. 2018. Developability of triangle meshes. ACM Trans. Graph. 37, 4 (2018), 77:1–77:14. Google ScholarDigital Library
23. Rasmus Tamstorf. 2013. Derivation of discrete bending forces and their gradients. Technical Report 07/2013. Disney Research.Google Scholar
24. Rasmus Tamstorf and Eitan Grinspun. 2013. Discrete bending forces and their Jacobians. Graph. Models 75 (2013), 362–370. Google ScholarDigital Library
25. Chengcheng Tang, Xiang Sun, Alexandra Gomes, Johannes Wallner, and Helmut Pottmann. 2014. Form-finding with polyhedral meshes made simple. ACM Trans. Graphics 33, 4 (2014), 70:1–70:9. Google ScholarDigital Library
26. Huanxi Zhao and Guoliang Xu. 2006. Triangular surface mesh fairing via Gaussian curvature flow. J. Comput. Appl. Math. 195 (2006), 300–311. Google ScholarDigital Library