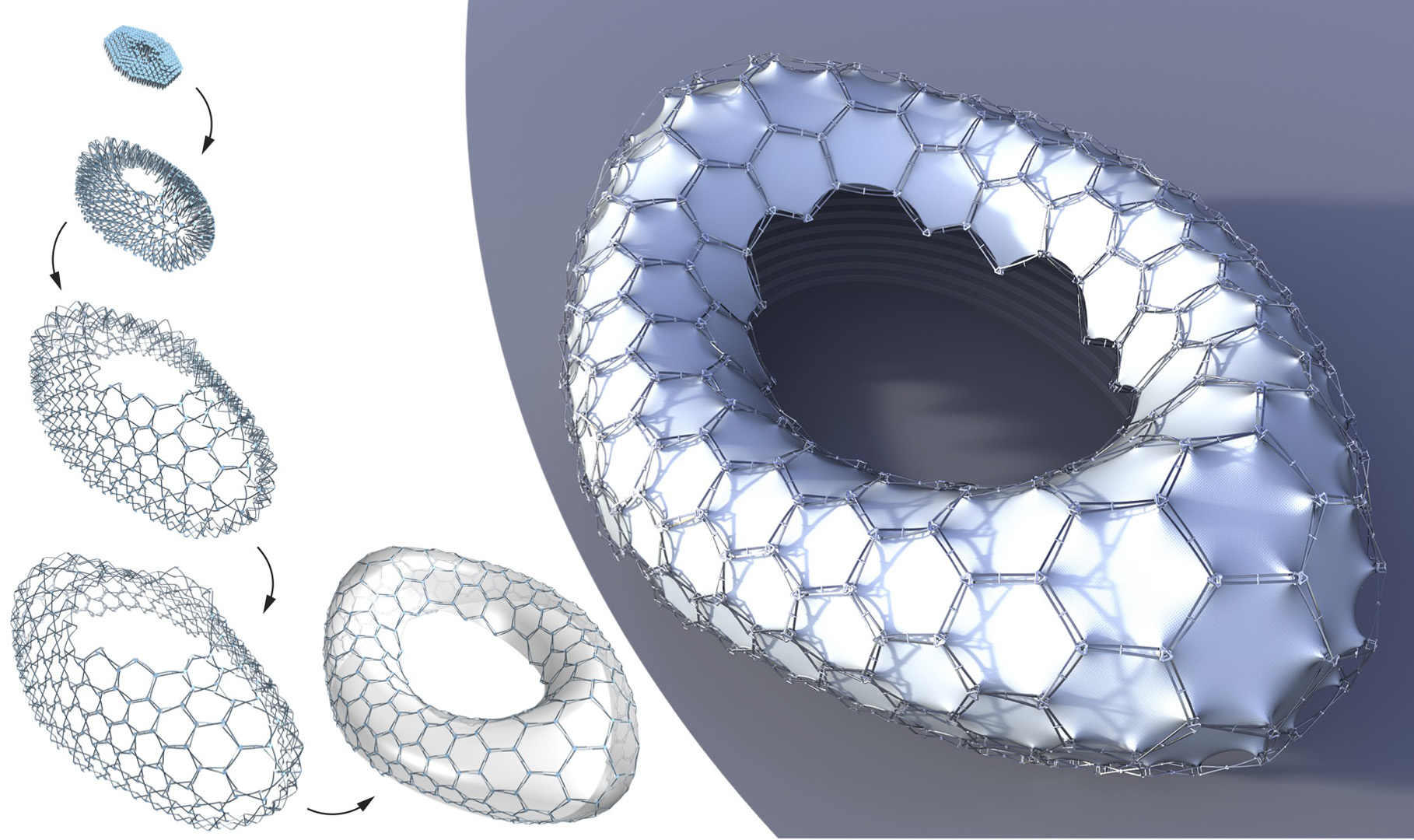
“Umbrella meshes: elastic mechanisms for freeform shape deployment” by Ren, Kusupati, Panetta, Isvoranu, Pellis, et al. …
Conference:
Type(s):
Title:
- Umbrella meshes: elastic mechanisms for freeform shape deployment
Presenter(s)/Author(s):
Abstract:
We present a computational inverse design framework for a new class of volumetric deployable structures that have compact rest states and deploy into bending-active 3D target surfaces. Umbrella meshes consist of elastic beams, rigid plates, and hinge joints that can be directly printed or assembled in a zero-energy fabrication state. During deployment, as the elastic beams of varying heights rotate from vertical to horizontal configurations, the entire structure transforms from a compact block into a target curved surface. Umbrella Meshes encode both intrinsic and extrinsic curvature of the target surface and in principle are free from the area expansion ratio bounds of past auxetic material systems.We build a reduced physics-based simulation framework to accurately and efficiently model the complex interaction between the elastically deforming components. To determine the mesh topology and optimal shape parameters for approximating a given target surface, we propose an inverse design optimization algorithm initialized with conformal flattening. Our algorithm minimizes the structure’s strain energy in its deployed state and optimizes actuation forces so that the final deployed structure is in stable equilibrium close to the desired surface with few or no external constraints. We validate our approach by fabricating a series of physical models at various scales using different manufacturing techniques.
References:
1. LIW Arnouts, Thierry J Massart, Niels De Temmerman, and PZ Berke. 2018. Computational modelling of the transformation of bistable scissor structures with geometrical imperfections. Engineering Structures 177 (2018), 409–420.Google ScholarCross Ref
2. Artelys. 2021. Artelys Knitro – Nonlinear optimization solver. https://www.artelys.com/en/optimization-tools/knitroGoogle Scholar
3. Miklós Bergou, Basile Audoly, Etienne Vouga, Max Wardetzky, and Eitan Grinspun. 2010. Discrete viscous threads. ACM T Graphic 29, 4 (2010), 116.Google ScholarDigital Library
4. Miklós Bergou, Max Wardetzky, Stephen Robinson, Basile Audoly, and Eitan Grinspun. 2008. Discrete elastic rods. ACM T Graphic 27, 3 (2008), 63.Google ScholarDigital Library
5. B. Bickel, M. Baecher, M. Otaduy, W. Matusik, H. Pfister, and M. Gross. 2009. Capture and Modeling of Non-Linear Heterogeneous Soft Tissue. Proc. ACM SIGGRAPH02+ 28, 3 (2009).Google Scholar
6. Tian Chen, Osama R Bilal, Robert Lang, Chiara Daraio, and Kristina Shea. 2019. Autonomous deployment of a solar panel using elastic origami and distributed shape-memory-polymer actuators. Physical Review Applied 11, 6 (2019), 064069.Google ScholarCross Ref
7. Tian Chen, Julian Panetta, Max Schnaubelt, and Mark Pauly. 2021. Bistable auxetic surface structures. ACM Transactions on Graphics (TOG) 40, 4 (2021), 1–9.Google ScholarDigital Library
8. Xiang Chen, Changxi Zheng, Weiwei Xu, and Kun Zhou. 2014. An Asymptotic Numerical Method for Inverse Elastic Shape Design. ACM Trans. Graph. 33, 4, Article 95 (jul 2014), 11 pages. Google ScholarDigital Library
9. Akash Garg, Andrew O Sageman-Furnas, Bailin Deng, Yonghao Yue, Eitan Grinspun, Mark Pauly, and Max Wardetzky. 2014. Wire mesh design. ACM Transactions on Graphics 33, 4 (2014).Google ScholarDigital Library
10. Ruslan Guseinov, Eder Miguel, and Bernd Bickel. 2017. CurveUps: Shaping Objects from Flat Plates with Tension-Actuated Curvature. ACM Trans. Graph. 36, 4, Article 64 (7 2017), 12 pages. Google ScholarDigital Library
11. Charles Hoberman. 1991. Radial expansion/retraction truss structures. US Patent 5,024,031.Google Scholar
12. David Jourdan, Melina Skouras, Etienne Vouga, and Adrien Bousseau. 2020. Printing-on-Fabric Meta-Material for Self-Shaping Architectural Models. In Advances in Architectural Geometry. http://www-sop.inria.fr/reves/Basilic/2020/JSVB20Google Scholar
13. Mina Konaković, Keenan Crane, Bailin Deng, Sofien Bouaziz, Daniel Piker, and Mark Pauly. 2016. Beyond developable: computational design and fabrication with auxetic materials. ACM Transactions on Graphics (TOG) 35, 4 (2016), 1–11.Google ScholarDigital Library
14. Mina Konaković-Luković, Julian Panetta, Keenan Crane, and Mark Pauly. 2018. Rapid deployment of curved surfaces via programmable auxetics. ACM Transactions on Graphics (TOG) 37, 4 (2018), 106.Google ScholarDigital Library
15. Julian Lienhard and Christoph Gengnagel. 2018. Recent developments in bending-active structures. In Proceedings of IASS Annual Symposia, Vol. 2018. International Association for Shell and Spatial Structures (IASS), 1–8.Google Scholar
16. Ligang Liu, Lei Zhang, Yin Xu, Craig Gotsman, and Steven J Gortler. 2008. A local/global approach to mesh parameterization. In Computer Graphics Forum, Vol. 27. Wiley Online Library, 1495–1504.Google ScholarDigital Library
17. Luigi Malomo, Jesús Pérez, Emmanuel Iarussi, Nico Pietroni, Eder Miguel, Paolo Cignoni, and Bernd Bickel. 2018. FlexMaps: computational design of flat flexible shells for shaping 3D objects. ACM Transactions on Graphics (TOG) 37, 6 (2018), 1–14.Google ScholarDigital Library
18. J Michael McCarthy and Gim Song Soh. 2010. Geometric design of linkages. Vol. 11. Springer Science & Business Media.Google Scholar
19. Robert McNeel et al. 2010. Rhinoceros 3D, Version 6.0. Robert McNeel & Associates, Seattle, WA (2010).Google Scholar
20. Giulio Molinari, Manfred Quack, Vitaly Dmitriev, Manfred Morari, Patrick Jenny, and Paolo Ermanni. 2011. Aero-structural optimization of morphing airfoils for adaptive wings. Journal of Intelligent Material Systems and Structures 22, 10 (2011), 1075–1089.Google ScholarCross Ref
21. Julian Panetta, Florin Isvoranu, Tian Chen, Emmanuel Siéfert, Benoît Roman, and Mark Pauly. 2021. Computational inverse design of surface-based inflatables. ACM Transactions on Graphics (TOG) 40, 4 (2021), 1–14.Google ScholarDigital Library
22. Julian Panetta, Mina Konaković-Luković, Florin Isvoranu, Etienne Bouleau, and Mark Pauly. 2019. X-Shells: A new class of deployable beam structures. ACM Transactions on Graphics (TOG) 38, 4 (2019), 1–15.Google ScholarDigital Library
23. Julian Panetta, Abtin Rahimian, and Denis Zorin. 2017. Worst-Case Stress Relief for Microstructures. ACM Trans. Graph. 36, 4, Article 122 (jul 2017), 16 pages. Google ScholarDigital Library
24. Jesús Pérez, Miguel A Otaduy, and Bernhard Thomaszewski. 2017. Computational design and automated fabrication of kirchhoff-plateau surfaces. ACM Transactions on Graphics (TOG) 36, 4 (2017), 1–12.Google ScholarDigital Library
25. Jesus Perez, Bernhard Thomaszewski, Stelian Coros, Bernd Bickel, José A. Canabal, Robert Sumner, and Miguel A. Otaduy. 2015. Design and Fabrication of Flexible Rod Meshes. ACM Trans. on Graphics (Proc. of ACM SIGGRAPH) 34, 4 (2015). http://www.gmrv.es/Publications/2015/PTCBCSO15Google Scholar
26. Stefan Pillwein, Kurt Leimer, Michael Birsak, and Przemyslaw Musialski. 2020. On Elastic Geodesic Grids and Their Planar to Spatial Deployment. ACM Trans. Graph. 39, 4, Article 125 (July 2020), 12 pages. Google ScholarDigital Library
27. Stefan Pillwein and Przemyslaw Musialski. 2021. Generalized Deployable Elastic Geodesic Grids. arXiv preprint arXiv:2111.08883 (2021).Google Scholar
28. Christina L Randall, Evin Gultepe, and David H Gracias. 2012. Self-folding devices and materials for biomedical applications. Trends in biotechnology 30, 3 (2012), 138–146.Google Scholar
29. Yingying Ren, Julian Panetta, Tian Chen, Florin Isvoranu, Samuel Poincloux, Christopher Brandt, Alison Martin, and Mark Pauly. 2021. 3D weaving with curved ribbons. ACM Transactions on Graphics (TOG) 40, 4 (2021), 1–15.Google ScholarDigital Library
30. Kelvin Roovers and Niels De Temmerman. 2017. Deployable scissor grids consisting of translational units. International Journal of Solids and Structures 121 (2017), 45–61.Google ScholarCross Ref
31. Kelvin Roovers, Lara Alegria Mira, and Niels De Temmerman. 2013. From surface to scissor structure. In Proceedings of the First Conference Transformables, Seville, Editorial Starbooks, Seville, Spain, Vol. 2.Google Scholar
32. Andrew O Sageman-Furnas, Albert Chern, Mirela Ben-Chen, and Amir Vaxman. 2019. Chebyshev nets from commuting PolyVector fields. ACM Transactions on Graphics (TOG) 38, 6 (2019), 1–16.Google ScholarDigital Library
33. Rohan Sawhney and Keenan Crane. 2017. Boundary first flattening. ACM Transactions on Graphics (ToG) 37, 1 (2017), 1–14.Google ScholarDigital Library
34. Christian Schumacher, Jonas Zehnder, and Moritz Bächer. 2018. Set-in-Stone: Worst-Case Optimization of Structures Weak in Tension. ACM Trans. Graph. 37, 6, Article 252 (Dec. 2018), 13 pages. Google ScholarDigital Library
35. Mélina Skouras, Bernhard Thomaszewski, Bernd Bickel, and Markus Gross. 2012. Computational Design of Rubber Balloons. Comput. Graphics Forum (Proc. Eurographics) (2012).Google Scholar
36. Mélina Skouras, Bernhard Thomaszewski, Stelian Coros, Bernd Bickel, and Markus Gross. 2013. Computational Design of Actuated Deformable Characters. ACM Trans. Graph. 32, 4, Article 82 (jul 2013), 10 pages. Google ScholarDigital Library
37. Yichao Tang, Gaojian Lin, Shu Yang, Yun Kyu Yi, Randall D Kamien, and Jie Yin. 2017. Programmable Kiri-Kirigami Metamaterials. Advanced Materials 29, 10 (2017), 1604262.Google ScholarCross Ref
38. Hideshi Tomita, Takashi Higaki, Toshiki Kobayashi, Takanari Fujii, and Kazuto Fujimoto. 2015. Stenting for curved lesions using a novel curved balloon: Preliminary experimental study. Journal of Cardiology 66, 2 (2015), 120–124. Google ScholarCross Ref
39. Ran Zhang, Thomas Auzinger, and Bernd Bickel. 2021. Computational Design of Planar Multistable Compliant Structures. ACM Transactions on Graphics (TOG) 40, 5 (2021), 1–16.Google ScholarDigital Library
40. Ran Zhang, Shiwei Wang, Xuejin Chen, Chao Ding, Luo Jiang, Jie Zhou, and Ligang Liu. 2015. Designing planar deployable objects via scissor structures. IEEE transactions on visualization and computer graphics 22, 2 (2015), 1051–1062.Google Scholar
41. Xiaoting Zhang, Guoxin Fang, Melina Skouras, Gwenda Gieseler, Charlie C. L. Wang, and Emily Whiting. 2019. Computational Design of Fabric Formwork. ACM Trans. Graph. 38, 4, Article 109 (jul 2019), 13 pages. Google ScholarDigital Library
42. Changxi Zheng, Timothy Sun, and Xiang Chen. 2016. Deployable 3D linkages with collision avoidance.. In Symposium on Computer Animation. 179–188.Google Scholar