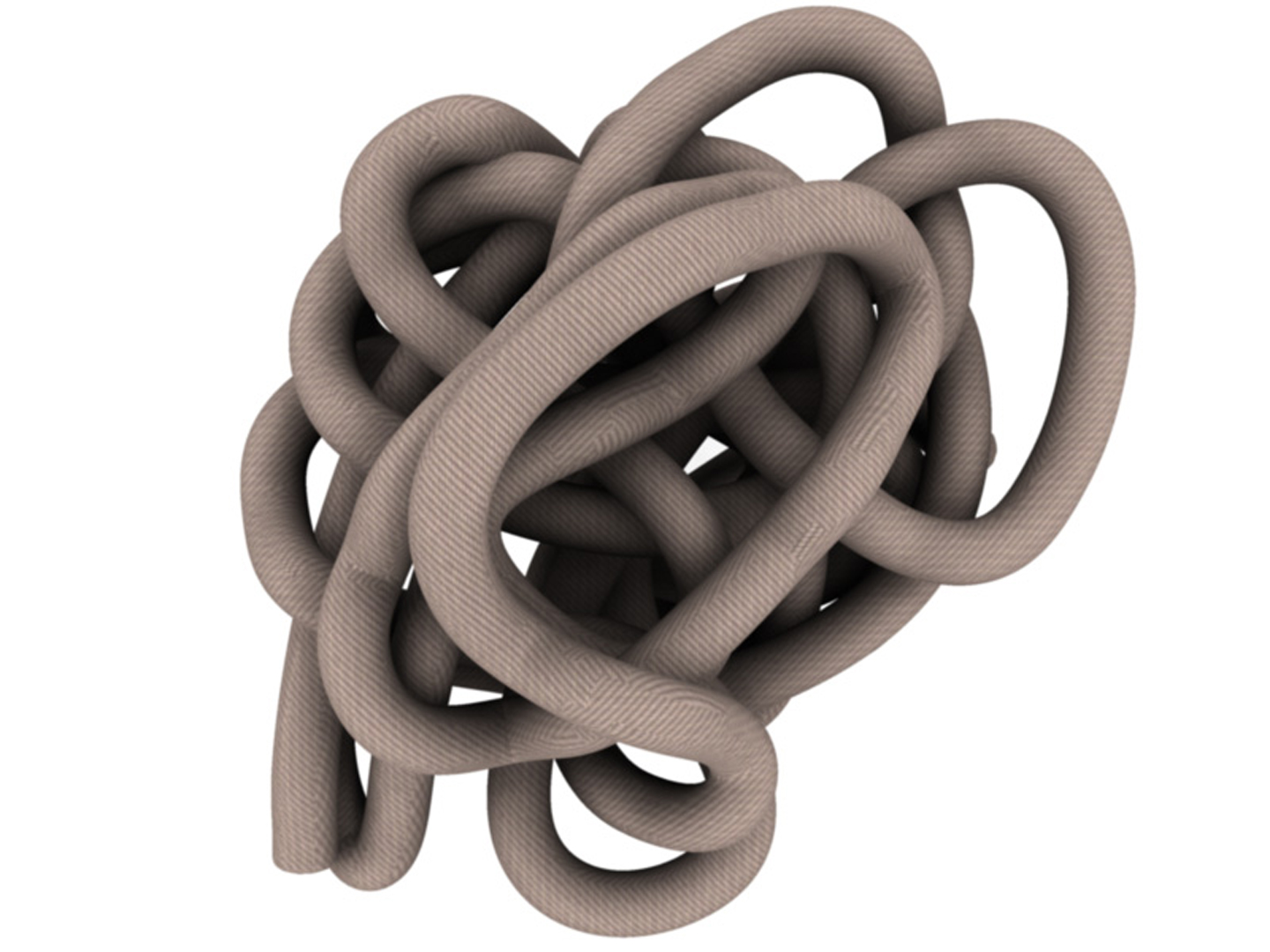
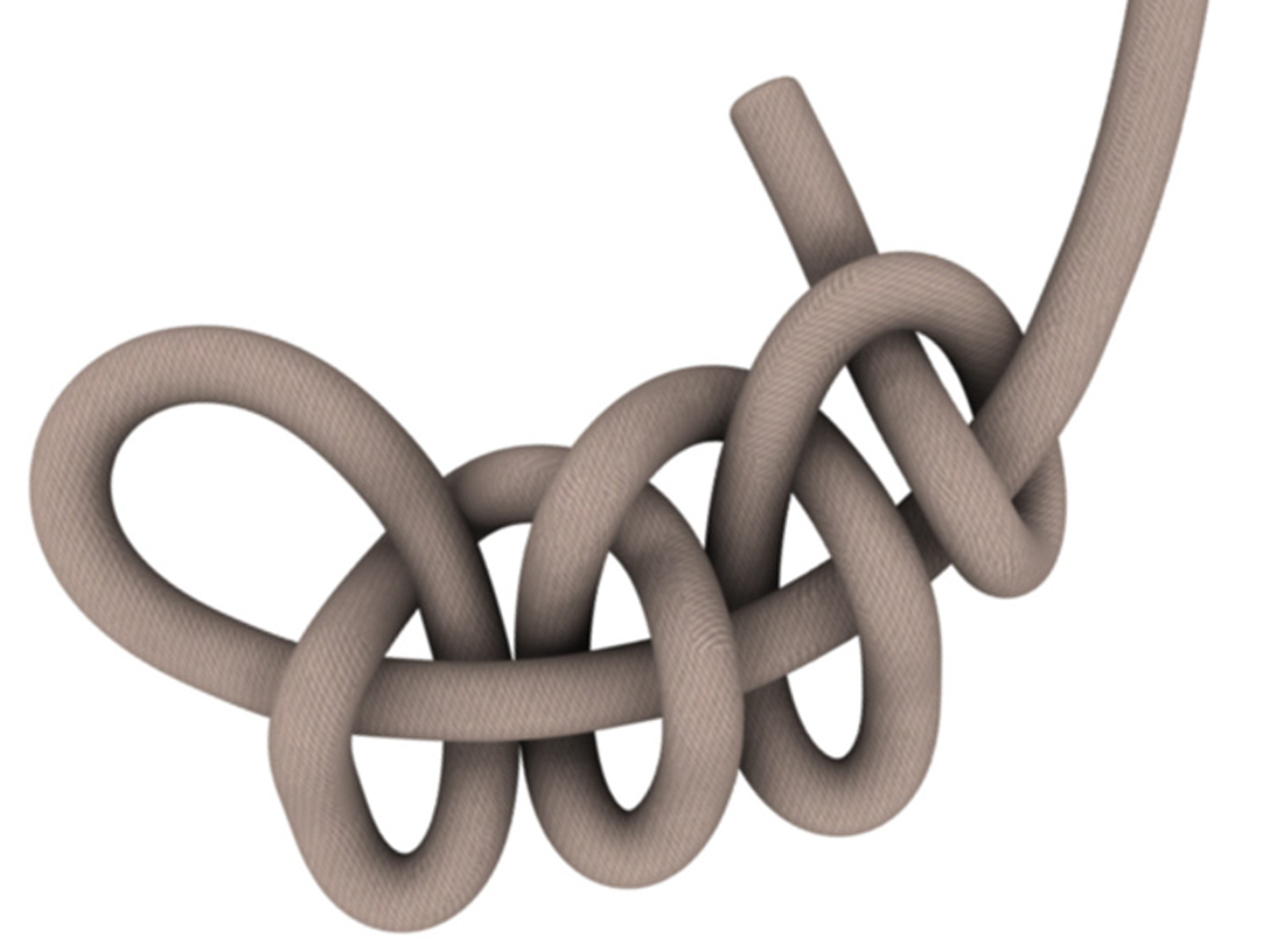
“Sketching knots” by Gonen and Akleman
Conference:
Type(s):
Title:
- Sketching knots
Presenter(s)/Author(s):
Abstract:
Physical knots have always fascinated people. For instance, Ashley Book of Knots contains some 7000 illustrations covering over 2000 knots. Physical knots usually associated with sailing, but they are also used in weaving, knitting, braiding and crocheting. Mathematical knots are introduced to formally classify the physical knots [Turner and de Griend 1996]. The significant difference with mathematical and physical knots is that in mathematical knots threads are closed curves. This property helps to formulate the problem more precisely and several knot polynomials such as Alexander and Jones polynomials are introduced to categorize the knots. In decorative arts, the most well-known knot form is Celtic knots, which are also good examples that shows how difficult to draw for humans knots. To draw Celtic knots and to obtain cyclic plain weaving on surfaces mesh based methods are introduced [Kaplan and Cohen 2003; Akleman et al. 2009]. Except mesh based methods, we do not know any other way to provide people to design knots. There exist software such as Mathematica’s knot theory package or Knotplot, but these software does not provide free form design of knots.
References:
1. Akleman, E., Chen, J., Xing, Q., and Gross, J. L. 2009. Cyclic plain-weaving on polygonal mesh surfaces with graph rotation systems. In ACM SIGGRAPH 2009 papers, SIGGRAPH ’09, 78:1–78:8.
2. Kaplan, M., and Cohen, E. 2003. Computer generated celtic design. In Proc. of 14th Eurographics Workshop on Rendering, 9–16.
3. Turner, J., and de Griend, P. V. 1996. History and Science of Knots. World Scientific Publishing, Singapore.
Additional Images: