“Predictive-corrective incompressible SPH” by Solenthaler and Pajarola
Conference:
Type(s):
Title:
- Predictive-corrective incompressible SPH
Presenter(s)/Author(s):
Abstract:
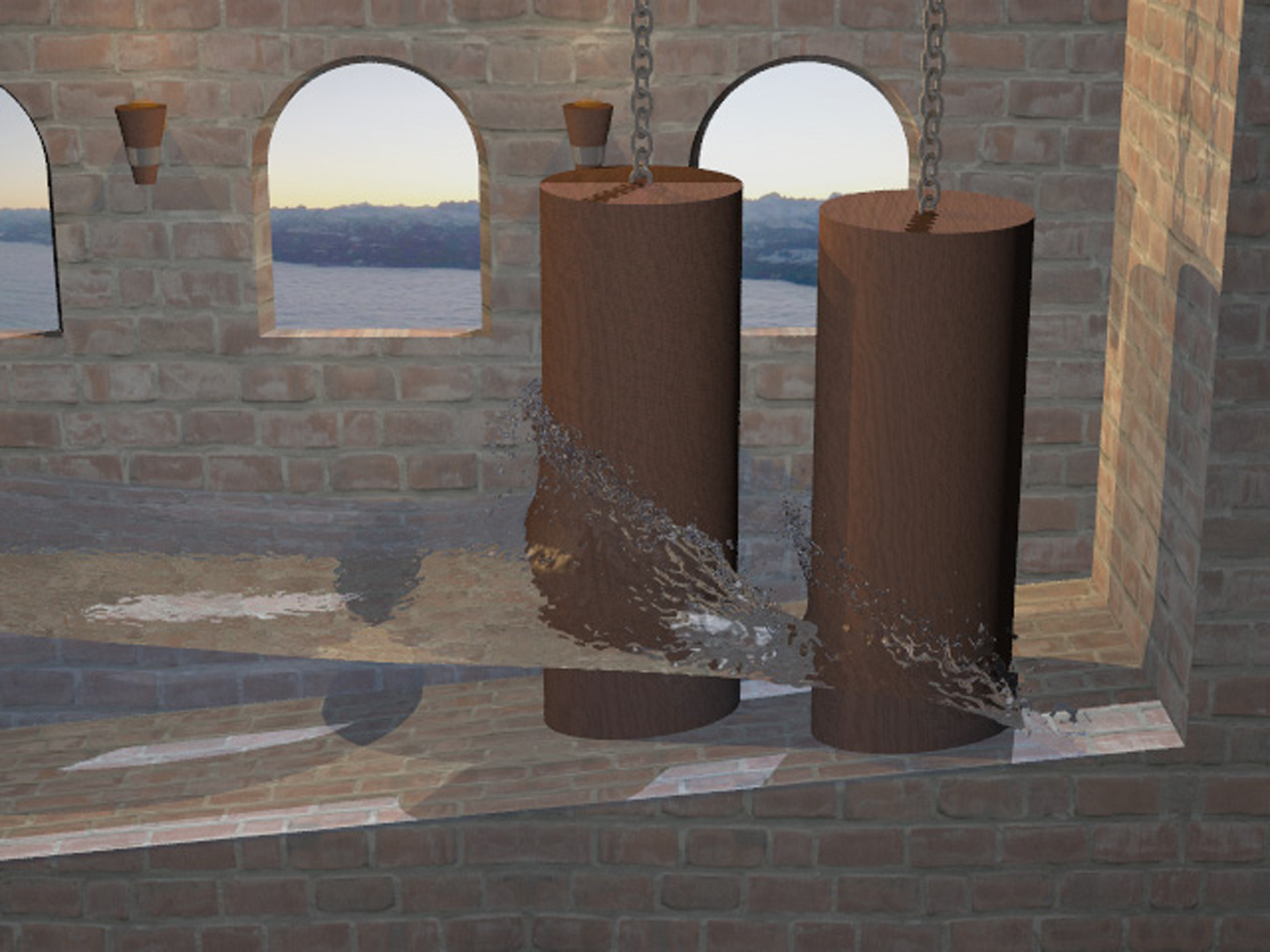
We present a novel, incompressible fluid simulation method based on the Lagrangian Smoothed Particle Hydrodynamics (SPH) model. In our method, incompressibility is enforced by using a prediction-correction scheme to determine the particle pressures. For this, the information about density fluctuations is actively propagated through the fluid and pressure values are updated until the targeted density is satisfied. With this approach, we avoid the computational expenses of solving a pressure Poisson equation, while still being able to use large time steps in the simulation. The achieved results show that our predictive-corrective incompressible SPH (PCISPH) method clearly outperforms the commonly used weakly compressible SPH (WCSPH) model by more than an order of magnitude while the computations are in good agreement with the WCSPH results.
References:
1. Adams, B., Pauly, M., Keiser, R., and Guibas, L. J. 2007. Adaptively sampled particle fluids. ACM Trans. Graph. 26, 3, 48–54. Google ScholarDigital Library
2. Batchelor, G. 1967. An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
3. Becker, M., and Teschner, M. 2007. Weakly compressible SPH for free surface flows. In Symposium on Computer Animation, 209–217. Google ScholarDigital Library
4. Becker, M., Tessendorf, H., and Teschner, M. 2009. Direct forcing for Lagrangian rigid-fluid coupling. IEEE Transactions on Visualization and Computer Graphics 15, 3, 493–503. Google ScholarDigital Library
5. Courant, R., Friedrichs, K., and Lewy, H. 1967. On the partial difference equations of mathematical physics. IBM J. 11, 215–234.Google ScholarDigital Library
6. Cummins, S. J., and Rudman, M. 1999. An SPH projection method. J. Comput. Phys. 152, 2, 584–607. Google ScholarDigital Library
7. Desbrun, M., and Cani, M.-P. 1996. Smoothed particles: A new paradigm for animating highly deformable bodies. In Eurographics Workshop on Computer Animation and Simulation, 61–76. Google ScholarDigital Library
8. Enright, D., Marschner, S., and Fedkiw, R. 2002. Animation and rendering of complex water surfaces. ACM Trans. Graph. 21, 3, 736–744. Google ScholarDigital Library
9. Hu, X. Y., and Adams, N. A. 2007. An incompressible multiphase SPH method. J. Comput. Phys. 227, 1, 264–278. Google ScholarDigital Library
10. J. Liu, S. K., and Oka, Y. 2005. A hybrid particle-mesh method for viscous, incompressible, multiphase flows. J. Comput. Phys. 202, 1, 65–93. Google ScholarDigital Library
11. Keiser, R., Adams, B., Gasser, D., Bazzi, P., Dutre, P., and Gross, M. 2005. A unified Lagrangian approach to solidfluid animation. In Proceedings of Eurographics Symposium on Point-Based Graphics, 125–133. Google ScholarDigital Library
12. Lenaerts, T., Adams, B., and Dutré, P. 2008. Porous flow in particle-based fluid simulations. ACM Trans. Graph. 27, 3, 1–8. Google ScholarDigital Library
13. Losasso, F., Talton, J., Kwatra, J., and Fedkiw, R. 2008. Two-way coupled SPH and particle level set fluid simulation. IEEE TVCG 14, 4, 797–804. Google ScholarDigital Library
14. Monaghan, J. 1992. Smoothed particle hydrodynamics. Annu. Rev. Astron. Physics 30, 543.Google ScholarCross Ref
15. Monaghan, J. 2005. Smoothed particle hydrodynamics. Rep. Prog. Phys. 68, 1703–1759.Google ScholarCross Ref
16. Müller, M., Charypar, D., and Gross, M. 2003. Particle-based fluid simulation for interactive applications. In Symposium on Computer Animation, 154–159. Google ScholarDigital Library
17. Müller, M., Keiser, R., Nealen, A., Pauly, M., Gross, M., and Alexa, M. 2004. Point based animation of elastic, plastic and melting objects. In Symposium on Computer Animation, 141–151. Google ScholarDigital Library
18. Müller, M., Schirm, S., Teschner, M., Heidelberger, B., and Gross, M. 2004. Interaction of fluids with deformable solids. Journal of Computer Animation and Virtual Worlds 15, 3–4, 159–171. Google ScholarDigital Library
19. Müller, M., Solenthaler, B., Keiser, R., and Gross, M. 2005. Particle-based fluid-fluid interaction. In Symposium on Computer Animation, 237–244. Google ScholarDigital Library
20. Premoze, S., Tasdizen, T., Bigler, J., Lefohn, A., and Whitaker, R. T. 2003. Particle-based simulation of fluids. In Proceedings of Eurographics, 401–410.Google Scholar
21. Selle, A., Rasmussen, N., and Fedkiw, R. 2005. A vortex particle method for smoke, water and explosions. ACM Trans. Graph. 24, 3, 910–914. Google ScholarDigital Library
22. Shao, S. 2006. Incompressible SPH simulation of wave breaking and overtopping with turbulence modelling. Int. J. Numer. Meth. Fluids 50, 597–621.Google ScholarCross Ref
23. Solenthaler, B., and Pajarola, R. 2008. Density contrast SPH interfaces. In Symposium on Computer Animation, 211–218. Google ScholarDigital Library
24. Solenthaler, B., Schläfli, J., and Pajarola, R. 2007. A unified particle model for fluid-solid interactions. Journal of Computer Animation and Virtual Worlds 18, 1, 69–82. Google ScholarDigital Library
25. Thürey, N., Keiser, R., Pauly, M., and Rüde, U. 2006. Detail-preserving fluid control. In Symposium on Computer Animation, 7–15. Google ScholarDigital Library
26. Zhu, Y., and Bridson, R. 2005. Animating sand as a fluid. ACM Trans. Graph. 24, 3, 965–972. Google ScholarDigital Library