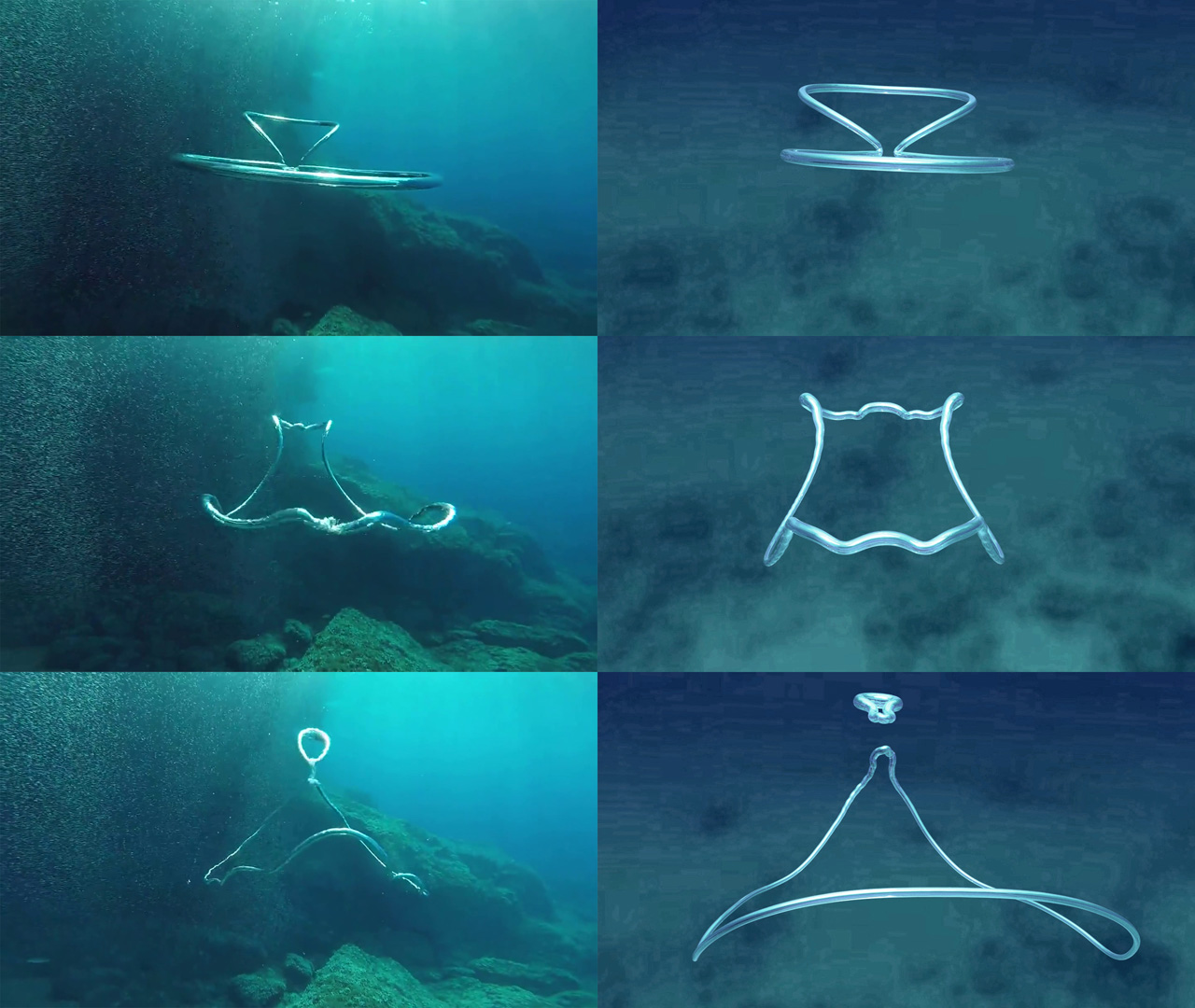
“On bubble rings and ink chandeliers” by Padilla, Chern, Knöppel, Pinkall and Schröder
Conference:
Type(s):
Title:
- On bubble rings and ink chandeliers
Session/Category Title: Fluids II
Presenter(s)/Author(s):
Abstract:
We introduce variable thickness, viscous vortex filaments. These can model such varied phenomena as underwater bubble rings or the intricate “chandeliers” formed by ink dropping into fluid. Treating the evolution of such filaments as an instance of Newtonian dynamics on a Riemannian configuration manifold we are able to extend classical work in the dynamics of vortex filaments through inclusion of viscous drag forces. The latter must be accounted for in low Reynolds number flows where they lead to significant variations in filament thickness and form an essential part of the observed dynamics. We develop and document both the underlying theory and associated practical numerical algorithms.
References:
1. Alexis Angelidis and Fabrice Neyret. 2005. Simulation of Smoke based on Vortex Filament Primitives. In Proc. Symp. Comp. Anim. ACM, 87–96. Google ScholarDigital Library
2. Vladimir I. Arnold and Boris A. Khesin. 1998. Topological Methods in Hydrodynamics. Springer. Google ScholarDigital Library
3. Harry Bateman. 1915. Some Recent Researches on the Motion of Fluids. Mon. Weath. R. 43, 4 (1915), 163–170.Google ScholarCross Ref
4. Mikl’os Bergou, Basile Audoly, Etienne Vouga, Max Wardetzky, and Eitan Grinspun. 2010. Discrete Viscous Threads. ACM Trans. Graph. 29, 4 (2010), 116:1–10. Google ScholarDigital Library
5. Peter S. Bernard. 2006. Turbulent Flow Properties of Large-scale Vortex Systems. PNAS 103, 27 (2006), 10174–10179.Google ScholarCross Ref
6. Peter S. Bernard. 2009. Vortex Filament Simulation of the Turbulent Coflowing Jet. Phys. Fluids 21, 2 (2009).Google Scholar
7. Danielis Bernoulli. 1738. Hydrodynamica, sive de viribus et motibus fluidorum commentarii. Argentorati. For a modern account see also {Grattan-Guinness 2005} and {Darrigol and Frisch 2008}.Google Scholar
8. Jean-Baptiste Biot and Nicolas-Pierre-Antoine Savart. 1820. Note sur le Magnétisme de la pile de Volta. Annal. Chimie et Phys. 15 (1820), 222–223.Google Scholar
9. Tyson Brochu, Todd Keeler, and Robert Bridson. 2012. Linear-Time Smoke Animation with Vortex Sheet Meshes. In Proc. Symp. Comp. Anim. Eurographics Assoc., 87–95. Google ScholarDigital Library
10. J. M. Burgers. 1948. A Mathematical Model Illustrating the Theory of Turbulence. Adv. Appl. Math. 1 (1948), 171–199.Google Scholar
11. Augustin-Louis Cauchy. 1815. Théorie de la Propagation des Ondes a la Surface d’un Fluide Pesant d’une Profondeur Indéfinie. In Oeuvres Complètes d’Augustin Cauchy. Vol. 1. Imprimerie Royale. Presented to the French Academy in 1815 (publ. 1827).Google Scholar
12. Ching Chang and Stefan G. Llewellyn Smith. 2018. The Motion of a Buoyant Vortex Filament. J. Fl. Mech. 857 (2018), R1:1–13.Google ScholarCross Ref
13. M. Cheng, J. Lou, and T. T. Lim. 2013. Motion of a Bubble Ring in a Viscous Fluid. Phys. Fluids 25, 6 (2013), 067104:1–19.Google Scholar
14. Stephen Childress. 2009. An Introduction to Theoretical Fluid Dynamics. AMS.Google Scholar
15. Alexandre Joel Chorin. 1990. Hairpin Removal in Vortex Interactions. J. Comput. Phys. 91, 1 (1990), 1–21. Google ScholarDigital Library
16. Alexandre Joel Chorin. 1993. Hairpin Removal in Vortex Interactions II. J. Comput. Phys. 107, 1 (1993), 1–9. Google ScholarDigital Library
17. Fang Da, Christopher Batty, Chris Wojtan, and Eitan Grinspun. 2015. Double Bubbles Sands Toil and Trouble: Discrete Circulation-Preserving Vortex Sheets for Soap Films and Foams. ACM Trans. Graph. 34, 4 (2015), 149:1–9. Google ScholarDigital Library
18. Fang Da, David Hahn, Christopher Batty, Chris Wojtan, and Eitan Grinspun. 2016. Surface-Only Liquids. ACM Trans. Graph. 35, 4 (2016), 78:1–12. Google ScholarDigital Library
19. O. Darrigol and U. Frisch. 2008. From Newton’s Mechanics to Euler’s Equations. Phy. D: Nonl. Phenom. 237, 14–17 (2008), 1855–1869.Google Scholar
20. Uriel Frisch and Barbara Villone. 2014. Cauchy’s almost Forgotten Lagrangian Formulation of the Euler Equation for 3D Incompressible Flow. Eu. Phy. J. H 39, 3 (2014), 325–351.Google ScholarCross Ref
21. Sylvestre Gallot, Dominique Hulin, and Jacques Lafontaine. 2004. Riemannian Geometry (3rd ed.). Springer.Google Scholar
22. S. K. Godunov. 1959. A Difference Method for Numerical Calculation of Discontinuous Solutions of the Equations of Hydrodynamics. Mat. Sb. (N.S.) 47(89), 3 (1959), 271–306.Google Scholar
23. Ivor Grattan-Guinness (Ed.). 2005. Landmark Writings in Western Mathematics 1640–1940. Elsevier, Chapter Daniel Bernoulli: Hydrodynamica (G. K. Mikhailov), 131–142.Google Scholar
24. Ernst Hairer, Syvert Paul Nørsett, and Gerhard Wanner. 1993. Solving Ordinary Differential Equations I: Nonstiff Problems (2nd ed.). Springer. Google ScholarDigital Library
25. Anton Izosimov and Boris Khesin. 2018. Vortex Sheets and Diffeomorphism Groupoids. Adv. Math. 338 (2018), 447–501.Google ScholarCross Ref
26. Theodor Kaluza. 1921. Zum Unitätsproblem der Physik. Sitzungsber. Preuss. Akad. Wiss. Berlin (1921), 966–972. English translation inGoogle Scholar
27. Oskar Klein. 1926. Quantentheorie und Fünfdimensionale Relativitätstheorie. Z. für Phys. 37, 12 (1926), 895–906.Google ScholarCross Ref
28. Eric Lauga and Thomas R. Powers. 2009. The Hydrodynamics of Swimming Microorganisms. Rep. Prog. Phys. 72 (2009), 096601:1–36.Google Scholar
29. Randall J. LeVeque. 2002. Finite-Volume Methods for Hyperbolic Problems. Cam. U. P.Google Scholar
30. Xiangyun Liao, Weixin Si, Zhiyong Yuan, Hanqiu Sun, Jing Qin, Qiong Wang, and Pheng-Ann Heng. 2018. Animating Wall-Bounded Turbulent Smoke via Filament-Mesh Particle-Particle Method. IEEE Trans. Vis. Comp. Graph. 24, 3 (2018), 1260–1273.Google ScholarCross Ref
31. Christian Loeschke. 2012. On the Relaxation of a Variational Principle for the Motion of a Vortex Sheet in Perfect Fluid. Ph.D. Dissertation. Rhein. Fried.-Wilh.-Univ. Bonn.Google Scholar
32. T. S. Lundgren and W. T. Ashurst. 1989. Area-Varying Waves on Curved Vortex Tuibes with Application to Vortex Breakdown. J. Fl. Mech. 200 (1989), 283–307.Google ScholarCross Ref
33. T. S. Lundgren and N. N. Mansour. 1988. Oscillations of Drops in Zero Gravity with Weak Viscous Effects. J. Fl. Mech. 194 (1988), 479–510.Google ScholarCross Ref
34. T. S. Lundgren and N. N. Mansour. 1991. Vortex Ring Bubbles. J. Fl. Mech. 224 (1991), 177–196.Google ScholarCross Ref
35. Jerrold Marsden and Alan Weinstein. 1983. Coadjoint Orbits, Vortices and Clebsch Variables for Incompressible Fluids. Phy. D: Nonl. Phenom. 7, 1–3 (1983), 305–323.Google Scholar
36. J. S. Marshall. 1991. A General Theory of Curved Vortices with Circular Cross-Section and Varialbe Core Area. J. Fl. Mech. 229 (1991), 311–338.Google ScholarCross Ref
37. V. V. Meleshko, A. A. Gourjii, and T. S. Krasnopolskaya. 2012. Vortex Rings: History and State of the Art. J. Math. Sc. 187, 6 (2012), 772–808.Google ScholarCross Ref
38. Derek William Moore and Philip Geoffrey Saffman. 1972. The Motion of a Vortex Filament with Axial Flow. Phil. Tr. R. Soc. Lond. A 272, 1226 (1972), 403–429.Google Scholar
39. T. J. Pedley. 1968. The Toroidal Bubble. J. Fl. Mech. 32, 1 (1968), 97–112.Google ScholarCross Ref
40. Tobias Pfaff, Nils Thuerey, and Markus Gross. 2012. Lagrangian Vortex Sheets for Animating Fluids. ACM Trans. Graph. 31, 4 (2012), 112:1–8. Google ScholarDigital Library
41. Bo Ren, Xu-Yun Yang, Ming C. Lin, Nils Thuerey, Matthias Teschner, and Chenfeng Li. 2018. Visual Simulation of Multiple Fluids in Computer Graphics: A State-of-the-Art Report. J. Comp. Sc. Tech. 33, 3 (2018), 431–451.Google Scholar
42. William B. Rogers. 1858. On the Formation of Rotating Rings by Air and Liquids under certain Conditions of Discharge. Am. J. Sc. A. 26, 77 (1858), 246–258.Google Scholar
43. Louis Rosenhead and Harold Jeffreys. 1930. The Spread of Vorticity in the Wake behind a Cylinder. Proc. R. Soc. Lond. A 127, 806 (1930), 590–612.Google ScholarCross Ref
44. P. G. Saffman. 1992. Vortex Dynamics. Cam. U. P.Google Scholar
45. Karim Shariff and Anthony Leonard. 1992. Vortex Rings. Ann. Rev. Fl. Mech. 24 (1992), 235–279.Google ScholarCross Ref
46. Michiko Shimokawa, Ryosei Mayumi, Taiki Nakamura, Toshiya Takami, and Hidetsugu Sakaguchi. 2016. Breakup and Deformation of a Droplet Falling in a Miscible Solution. Phys. R. E 93, 6 (2016), 062214:1–9.Google Scholar
47. Mark J. Stock, Werner J. A. Dahm, and Grétar Tryggvason. 2008. Impact of a Vortex Ring on a Density Interface using a Regularized Inviscid Vortex Sheet Method. J. Comput. Phys. 227, 21 (2008), 9021–9043. See also images at http://markjstock.com/#/chaoticescape/. Google ScholarDigital Library
48. G. I. Taylor. 1953. Formation of a Vortex Ring by Giving an Impulse to a Circular Disk and then Dissolving it Away. J. Appl. Ph. 24, 1 (1953), 104–105.Google ScholarCross Ref
49. J. J. Thomson. 1883. A Treatise on the Motion of Vortex Rings. Macmillan, London.Google Scholar
50. J. J. Thomson and H. F. Newall. 1886. On the Formation of Vortex Rings by Drops falling into Liquids, and some allied Phenomena. Proc. R. Soc. Lond. 39, 239–241 (1886), 417–436.Google Scholar
51. Charles Tomlinson. 1864. LXV. On a New Vareity of the Cohesion-Figures of Liquids. Lon. Edin. Dub. Phil. M. J. Sc. 27, 184 (1864), 425–432.Google Scholar
52. J. S. Turner. 1957. Buoyant Vortex Rings. Proc. R. Soc. Lond. A 239, 1216 (1957), 61–75.Google Scholar
53. Hermann von Helmholtz. 1858. Über Integrale der hydrodynamischen Gleichungen, welche den Wirbelbewegungen entsprechen. J. Reine Angew. Math. 55 (1858), 25–55.Google ScholarCross Ref
54. Steffen Weißmann and Ulrich Pinkall. 2010. Filament-based Smoke with Vortex Shedding and Variational Reconnection. ACM Trans. Graph. 29, 4 (2010), 115:1–12. Google ScholarDigital Library
55. G. B. Whitham. 1974. Linear and Nonlinear Waves. Wiley.Google Scholar
56. Sheila E. Widnall and Donald B. Bliss. 1971. Slender-body Analysis of the Motion and Stability of a Vortex Filament Containing an Axial Flow. J. Fl. Mech. 50, 2 (1971), 335–353.Google ScholarCross Ref