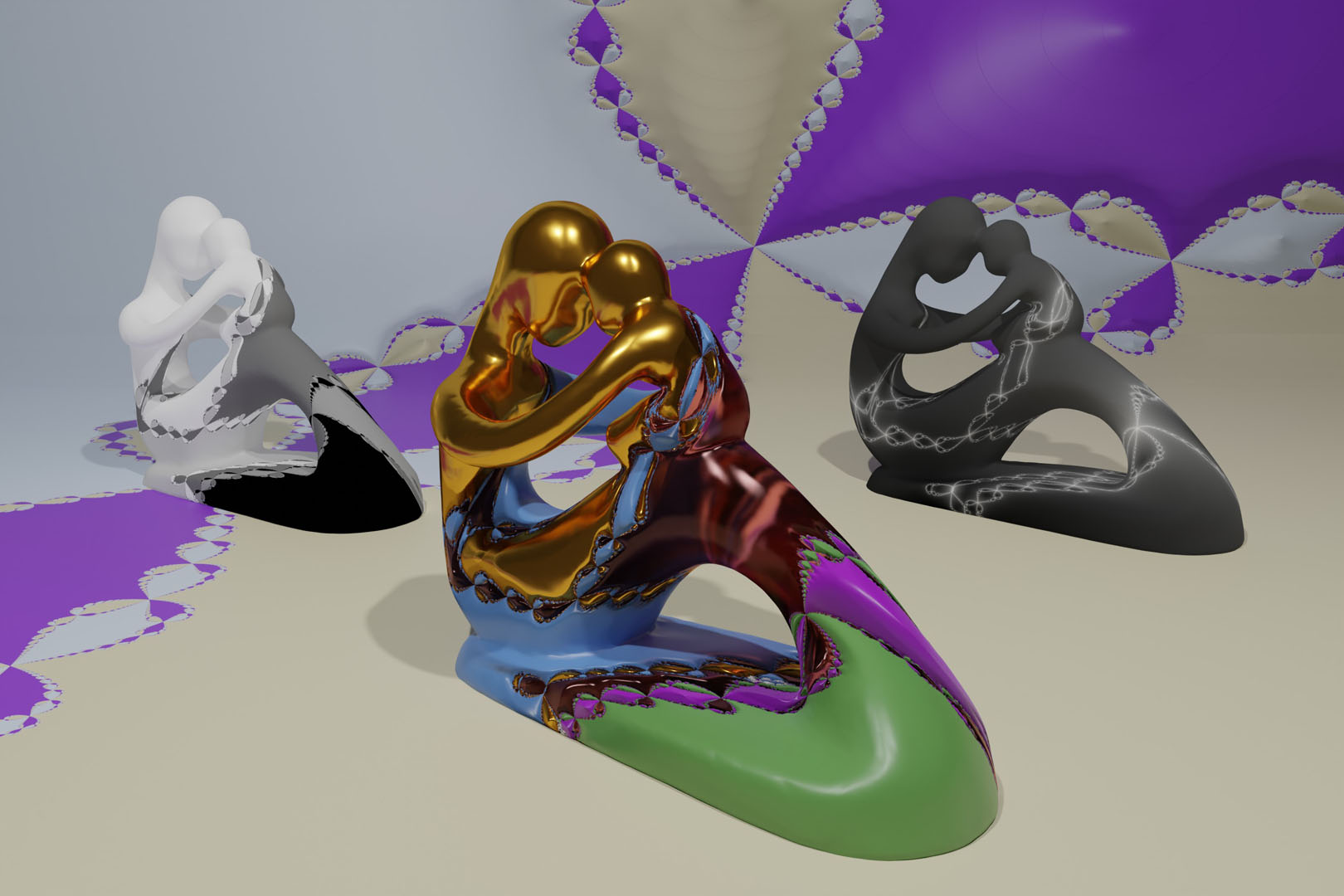
“Newton’s Fractals on Surfaces via Bicomplex Algebra” by Maggioli, Baieri, Melzi and Rodolà
Conference:
Type(s):
Entry Number: 65
Title:
- Newton’s Fractals on Surfaces via Bicomplex Algebra
Presenter(s)/Author(s):
References:
C.M. Davenport. 1991. A Commutative Hypercomplex Calculus with Applications to Special Relativity. Eigenverl.Google Scholar
C.M. Davenport. 1996. A Commutative Hypercomplex Algebra with Associated Function Theory. Birkhauser Boston Inc., USA, 213–227.Google Scholar
M.E. Luna-Elizarrarás, M. Saphiro, D.C. Struppa, and A. Vajiac. 2012. Bicomplex Numbers and their Elementary Functions. Cubo (Temuco) 14 (00 2012), 61 – 80.Google ScholarCross Ref
H.O. Peitgen, D. Saupe, Y. Fisher, M.F. Barnsley, Association for Computing Machinery Special Interest Group on Graphics, M. McGuire, B.B. Mandelbrot, R.L. Devaney, and R.F. Voss. 1988. The Science of Fractal Images. Springer New York.Google Scholar
A.A. Pogorui and R.M. Rodríguez-Dagnino. 2006. On the set of zeros of bicomplex polynomials. Complex Variables and Elliptic Equations 51, 7 (2006), 725–730.Google ScholarCross Ref
S. Rönn. 2001. Bicomplex algebra and function theory. arxiv:math/0101200 [math.CV]Google Scholar
X.-Y. Wang and W. Song. 2013. The generalized M–J sets for bicomplex numbers. Nonlinear Dynamics 72(2013), 17–26.Google ScholarCross Ref