“Moana: Crashing Waves”
Conference:
Type(s):
Entry Number: 41
Title:
- Moana: Crashing Waves
Presenter(s)/Author(s):
Abstract:
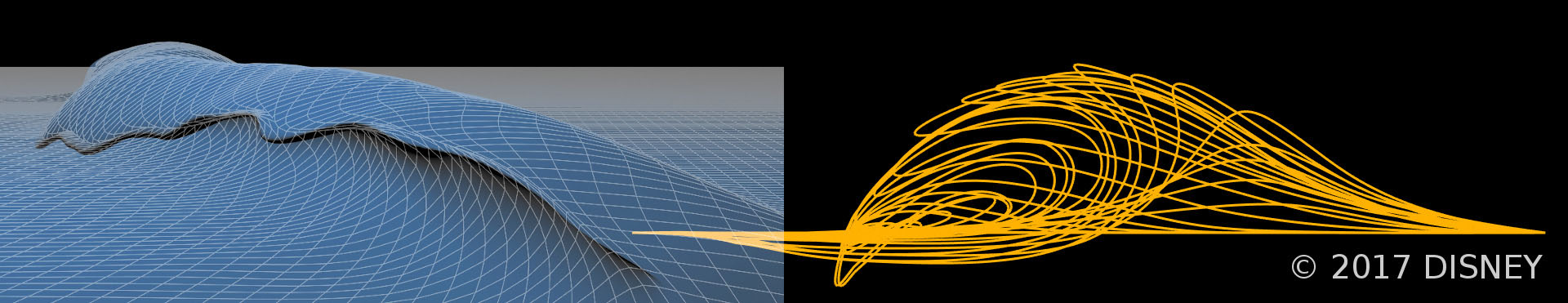
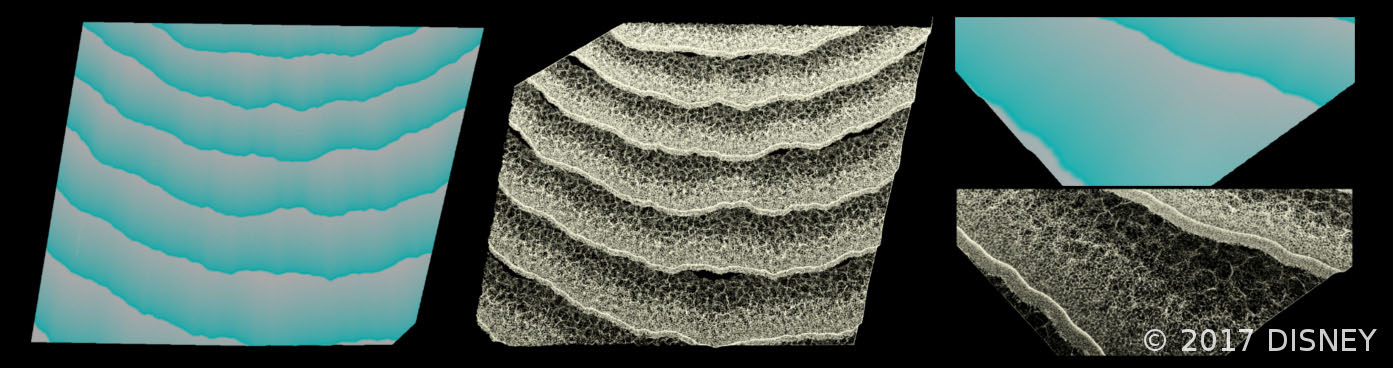
We used two different solutions for generating crashing waves for more than 40 shots in Moana. Our profile curve based wave deformer was developed and used for art-directed design of shapes, motion, and composition of running and crashing waves. In contrast to previously developed wave deformers, we designed a cross section shape animation by providing a series of profile curves which represented the animation keys. These profile curves could be hand plotted curves or mathematically calculated changing profiles, which means any kind of choreographic touch could be applied for designing the wave shapes. We could design multiple crashing waves for huge scale tsunami scenes and we could art direct the timing and composition of the waves which would fit well with the character animation and camera works.
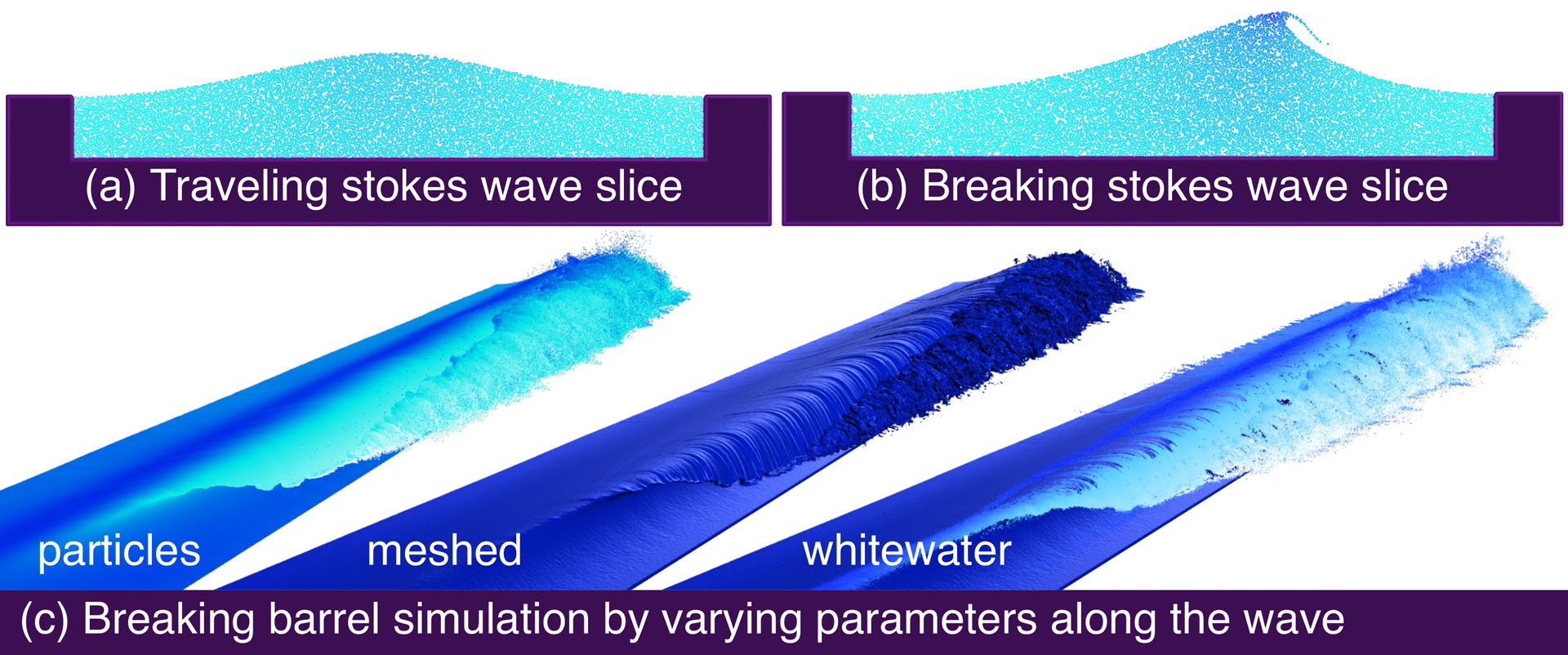
For scenarios demanding more realism, motion complexity and physical accuracy, we adopted a fully simulated approach. Our APIC-based fluid solver [Jiang et al. 2015] was equipped with control mechanisms allowing us to precisely choreograph the motion of breaking waves to the needs of a specific shot. Though more expensive than procedural approaches, this solution was much more preferable for “hero” shots with close up interaction with boats and characters.
References:
Chenfanfu Jiang, Craig Schroeder, Andrew Selle, Joseph Teran, and Alexey Stomakhin. 2015. The Affine Particle-in-cell Method. ACM Trans. Graph. 34, 4, Article 51 (July 2015), 10 pages. Google ScholarDigital Library
G.G. Stokes. 1847. On the theory of oscillatory waves. Trans. of the Cambridge Philosophical Society (184).Google Scholar
Alexey Stomakhin and Andrew Selle. 2017. Fluxed Animated Boundary Method. ACM Trans. Graph. 36, 4, Article 68 (July 2017), 68:1–68:11 pages.