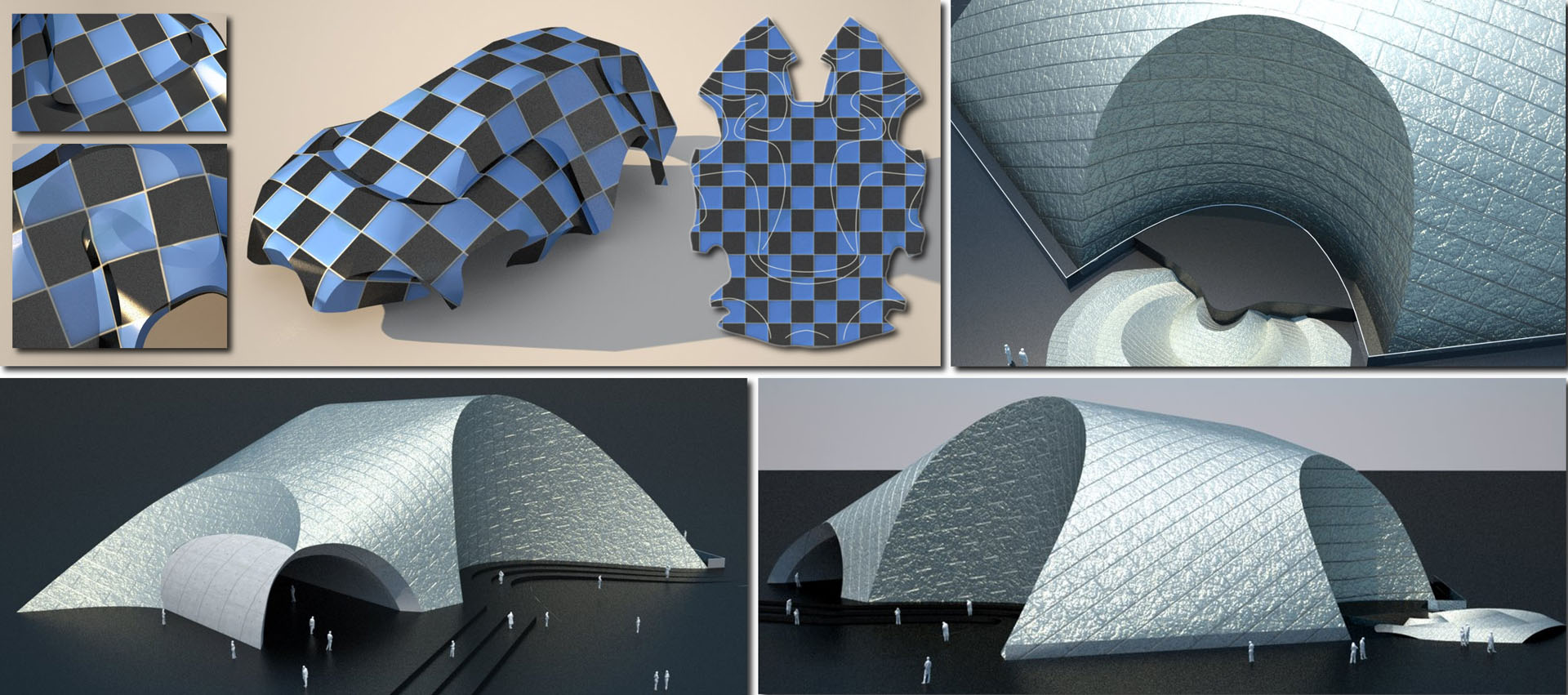
“Curved folding” by Kilian, Flöry, Chen, Mitra, Sheffer, et al. …

Fascinating and elegant shapes may be folded from a single planar sheet of material without stretching, tearing or cutting, if one incorporates curved folds into the design. We present an optimization-based computational framework for design and digital reconstruction of surfaces which can be produced by curved folding. Our work not only contributes to applications in architecture and industrial design, but it also provides a new way to study the complex and largely unexplored phenomena arising in curved folding.

1. Aumann, G. 2004. Degree elevation and developable Bézier surfaces. Comp. Aided Geom. Design 21, 661–670. Google ScholarDigital Library
2. Bo, P., and Wang, W. 2007. Geodesic-controlled developable surfaces for modeling paper bending. Comp. Graphics Forum 26, 3, 365–374.Google ScholarCross Ref
3. Bobenko, A., and Suris, Y., 2005. Discrete differential geometry. Consistency as integrability. Preprint, http://arxiv.org/abs/math.DG/0504358.Google Scholar
4. Botsch, M., Pauly, M., Gross, M., and Kobbelt, L. 2006. Primo: coupled prisms for intuitive surface modeling. In Symp. Geom. Processing, 11–20. Google ScholarDigital Library
5. Cerda, E., Chaieb, S., Melo, F., and Mahadevan, L. 1999. Conical dislocations in crumpling. Nature 401, 46–49.Google ScholarCross Ref
6. Cerda, E., Mahadevan, L., and Pasini, J. M. 2004. The elements of draping. Proc. Nat. Acad. Sciences 101, 7, 1806–1810.Google ScholarCross Ref
7. Chu, C. H., and Sequin, C. 2002. Developable Bézier patches: properties and design. Comp.-Aided Design 34, 511–528.Google ScholarCross Ref
8. Davis, T. A., and Hage, W. W. 2001. Multiple-rank modifications of a sparse cholesky factorization. SIAM Journal on Matrix Analysis and Applications 22, 4, 997–1013. Google ScholarDigital Library
9. Demaine, E., and O’Rourke, J. 2007. Geometric Folding Algorithms. Cambridge Univ. Press. Google ScholarDigital Library
10. Desbrun, M., Polthier, K., and Schröder, P. 2005. Discrete Differential Geometry. Siggraph Course Notes.Google Scholar
11. Do Carmo, M. 1976. Differential Geometry of Curves and Surfaces. Prentice-Hall.Google Scholar
12. Frey, W. 2004. Modeling buckled developable surfaces by triangulation. Comp.-Aided Design 36, 4, 299–313.Google ScholarCross Ref
13. Glaeser, G., and Gruber, F. 2007. Developable surfaces in contemporary architecture. J. of Math. and the Arts 1, 1–15.Google ScholarCross Ref
14. Huffman, D. A. 1976. Curvature and creases: a primer on paper. IEEE Trans. Computers C-25, 1010–1019. Google ScholarDigital Library
15. Julius, D., Kraevoy, V., and Sheffer, A. 2005. D-charts: Quasi-developable mesh segmentation. Computer Graphics Forum 24, 3, 581–590. Proc. Eurographics 2005.Google ScholarCross Ref
16. Kergosien, Y., Gotuda, H., and Kunii, T. 1994. Bending and creasing virtual paper. IEEE Comp. Graph. Appl. 14, 40–48. Google ScholarDigital Library
17. Kilian, M., Mitra, N., and Pottmann, H. 2007. Geometric modeling in shape space. ACM Trans. Graphics 26, 3, 64. Google ScholarDigital Library
18. Liu, Y., Pottmann, H., Wallner, J., Yang, Y.-L., and Wang, W. 2006. Geometric modeling with conical meshes and developable surfaces. ACM Trans. Graphics 25, 3, 681–689. Google ScholarDigital Library
19. Massarwi, F., Gotsman, C., and Elber, G. 2006. Papercraft models using generalized cylinders. In Pacific Graph., 148–157. Google ScholarDigital Library
20. Mitani, J., and Suzuki, H. 2004. Making papercraft toys from meshes using strip-based approximate unfolding. ACM Trans. Graphics 23, 3, 259–263. Google ScholarDigital Library
21. Ohtake, Y., Belyaev, A., and Seidel, H.-P. 2004. Ridge-valley lines on meshes via implicit surface fitting. ACM Trans. Graph. 23, 3 (August), 609–612. Google ScholarDigital Library
22. Pérez, F., and Suárez, J. A. 2007. Quasi-developable B-spline surfaces in ship hull design. Comp.-Aided Design 39, 853–862.Google ScholarCross Ref
23. Peternell, M. 2004. Developable surface fitting to point clouds. Comput. Aided Geom. Des. 21, 8, 785–803. Google ScholarDigital Library
24. Pottmann, H., and Wallner, J. 2001. Computational Line Geometry. Springer. Google ScholarDigital Library
25. Pottmann, H., Huang, Q.-X., Yang, Y.-L., and Hu, S.-M. 2006. Geometry and convergence analysis of algorithms for registration of 3D shapes. Int. J. Computer Vision 67, 3, 277–296. Google ScholarDigital Library
26. Pottmann, H., Asperl, A., Hofer, M., and Kilian, A. 2007. Architectural Geometry. Bentley Institute Press.Google Scholar
27. Rose, K., Sheffer, A., Wither, J., Cani, M.-P., and Thibert, B. 2007. Developable surfaces from arbitrary sketched boundaries. In Symp. Geometry Processing. 163–172. Google ScholarDigital Library
28. Sauer, R. 1970. Differenzengeometrie. Springer.Google Scholar
29. Shatz, I., Tal, A., and Leifman, G. 2006. Papercraft models from meshes. Vis. Computer 22, 825–834. Google ScholarDigital Library
30. Sheffer, A., Praun, E., and Rose, K. 2006. Mesh parameterization methods and their applications. Found. Trends. Comput. Graph. Vis. 2, 2, 105–171. Google ScholarDigital Library
31. Shelden, D. 2002. Digital surface representation and the constructibility of Gehry’s architecture. PhD thesis, M.I.T.Google Scholar
32. Subag, J., and Elber, G. 2006. Piecewise developable surface approximation of general NURBS surfaces with global error bounds. In Proc. Geometric Modeling and Processing. 143–156. Google ScholarDigital Library
33. Wang, C., and Tang, K. 2004. Achieving developability of a polygonal surface by minimum deformation: a study of global and local optimization approaches. Vis. Computer 20, 521–539. Google ScholarDigital Library
34. Wang, C. C. L. 2008. Towards flattenable mesh surfaces. Comput. Aided Des. 40, 1, 109–122. Google ScholarDigital Library
35. Wertheim, M. 2004. Cones, Curves, Shells, Towers: He Made Paper Jump to Life. The New York Times, June 22.Google Scholar