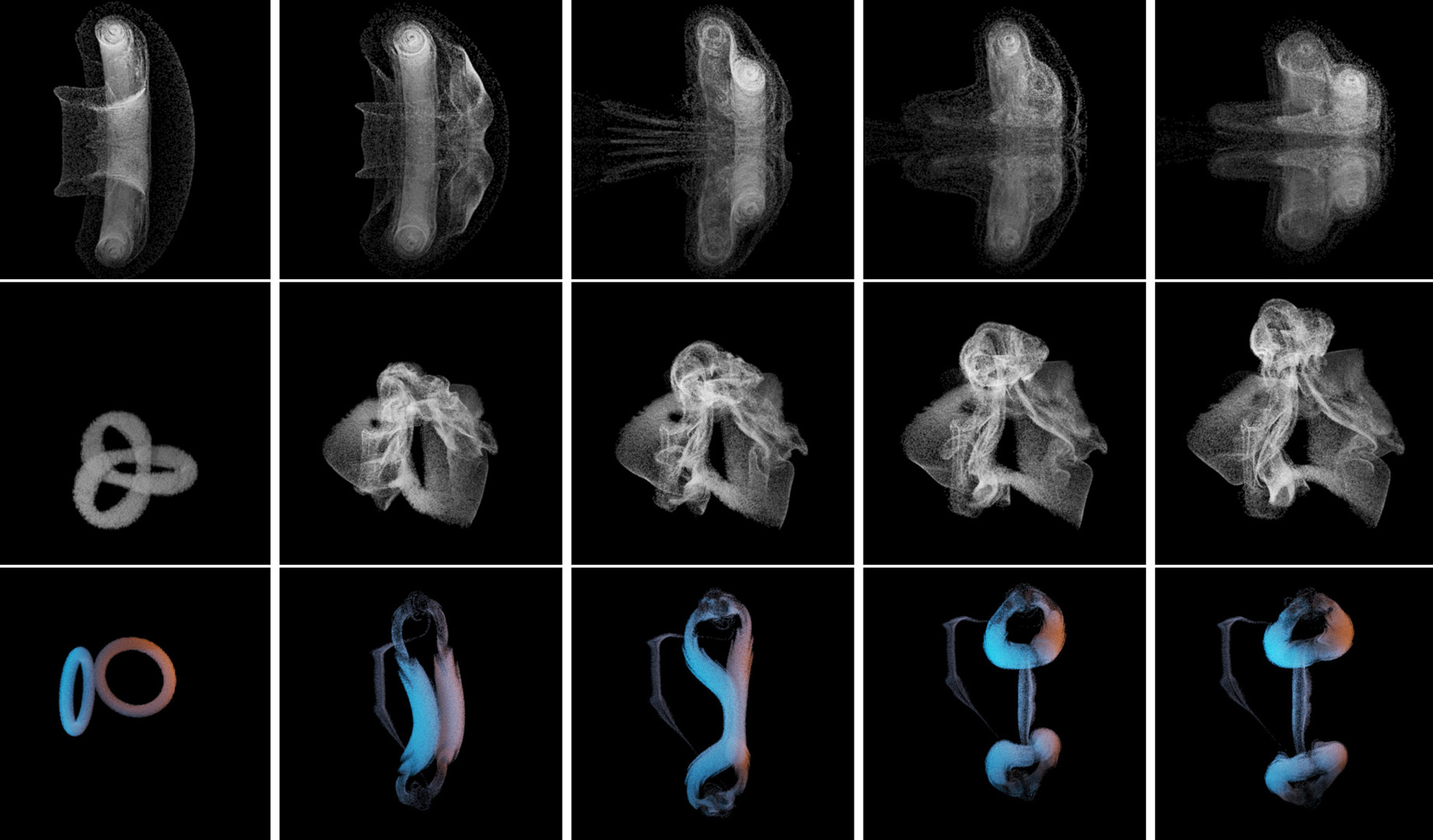
“Clebsch gauge fluid” by , Xiong, Zhang, Feng, Liu, et al. …

We propose a novel gauge fluid solver based on Clebsch wave functions to solve incompressible fluid equations. Our method combines the expressive power of Clebsch wave functions to represent coherent vortical structures and the generality of gauge methods to accommodate a broad array of fluid phenomena. By evolving a transformed wave function as the system’s gauge variable enhanced by an additional projection step to enforce pressure jumps on the free boundaries, our method can significantly improve the vorticity generation and preservation ability for a broad range of gaseous and liquid phenomena. Our approach can be easily implemented by modifying a standard grid-based fluid simulator. It can be used to solve various fluid dynamics, including complex vortex filament dynamics, fluids with different obstacles, and surface-tension flow.

1. Alexis Angelidis and Fabrice Neyret. 2005. Simulation of smoke based on vortex filament primitives. In Proceedings of the 2005 ACM SIGGRAPH/Eurographics symposium on Computer animation. 87–96.Google ScholarDigital Library
2. Landon Boyd and Robert Bridson. 2012. MultiFLIP for energetic two-phase fluid simulation. ACM Transactions on Graphics (TOG) 31, 2 (2012), 1–12.Google ScholarDigital Library
3. Jeremiah U Brackbill and Hans M Ruppel. 1986. FLIP: A method for adaptively zoned, particle-in-cell calculations of fluid flows in two dimensions. J. Comput. Phys. 65, 2 (1986), 314–343.Google ScholarDigital Library
4. Axel Brandenburg. 2010. Magnetic field evolution in simulations with euler potentials. MON. NOT. R. ASTRON. SOC. 401 (2010), 347–354.Google ScholarCross Ref
5. Robert Bridson, Jim Houriham, and Marcus Nordenstam. 2007. Curl-noise for procedural fluid flow. ACM Transactions on Graphics (ToG) 26, 3 (2007).Google ScholarDigital Library
6. Tyson Brochu, Todd Keeler, and Robert Bridson. 2012. Linear-time smoke animation with vortex sheet meshes. In Proceedings of the ACM SIGGRAPH/Eurographics Symposium on Computer Animation. Citeseer, 87–95.Google Scholar
7. Tomas F. Buttke. 1993. Velicity Methods: Lagrangian Numerical Methods which Preserve the Hamiltonian Structure of Incompressible Fluid Flow. Springer Netherlands, Dordrecht, 39–57.Google Scholar
8. Thomas F. Buttke and Alexandre J. Chorin. 1993. Turbulence calculations in magnetization variables. Applied Numerical Mathematics 12, 1 (1993), 47 — 54. SPECIAL ISSUE.Google ScholarCross Ref
9. C. Cartes, M. D. Bustamante, and M. E. Brachet. 2007. Generalized Eulerian-Lagrangian description of Navier-Stokes dynamics. Phys. Fluids 19 (2007), 077101.Google ScholarCross Ref
10. A. Chern. 2017. Fluid Dynamics with Incompressible Schrödinger Flow. Ph.D. Dissertation. California institute of technology.Google Scholar
11. A. Chern, F. Knöppel, U. Pinkall, and P. Schröder. 2017. Inside fluids: Clebsch maps for visualization and processing. ACM Trans. Graph. 36 (2017), 142.Google ScholarDigital Library
12. A. Chern, F. Knöppel, U. Pinkall, P. Schröder, and S. Weißmann. 2016. Schrödinger’s smoke. ACM Trans. Graph. 35 (2016), 77.Google ScholarDigital Library
13. A. Clebsch. 1859. Ueber die Integration der hydrodynamischen Gleichungen. J. Reine Angew. Math. 56 (1859), 1–10.Google ScholarCross Ref
14. Georges-Henri Cottet, Petros D Koumoutsakos, et al. 2000. Vortex methods: theory and practice. Vol. 8. Cambridge university press Cambridge.Google Scholar
15. Ounan Ding, Tamar Shinar, and Craig Schroeder. 2020. Affine particle in cell method for MAC grids and fluid simulation. J. Comput. Phys. 408 (2020), 109311.Google ScholarCross Ref
16. Aleksandar Donev, Andy Nonaka, Yifei Sun, Thomas Fai, Alejandro Garcia, and John Bell. 2014. Low Mach number fluctuating hydrodynamics of diffusively mixing fluids. Communications in Applied Mathematics and Computational Science 9, 1 (May 2014), 47–105.Google ScholarCross Ref
17. Weinan E and Jian-Guo Liu. 1997. Finite Difference Schemes for Incompressible Flows in the Velocity-Impulse Density Formulation. J. Comput. Phys. 130, 1 (1997), 67 — 76.Google ScholarDigital Library
18. Sharif Elcott, Yiying Tong, Eva Kanso, Peter Schröder, and Mathieu Desbrun. 2007. Stable, circulation-preserving, simplicial fluids. ACM Transactions on Graphics (TOG) 26, 1 (2007).Google ScholarDigital Library
19. Ronald Fedkiw, J. Stam, and H. Jensen. 2001. Visual simulation of smoke. Proceedings of the 28th annual conference on Computer graphics and interactive techniques (2001).Google ScholarDigital Library
20. N. Foster and Ronald Fedkiw. 2001. Practical animation of liquids. Proceedings of the 28th annual conference on Computer graphics and interactive techniques (2001).Google ScholarDigital Library
21. C. Fu, Q. Guo, Theodore F. Gast, Chenfanfu Jiang, and J. Teran. 2017. A polynomial particle-in-cell method. ACM Transactions on Graphics (TOG) 36 (2017), 1–12.Google ScholarDigital Library
22. S. Gagniere, David Hyde, A. Marquez-Razon, C. Jiang, Z. Ge, X. Han, Q. Guo, and J. Teran. 2020. A Hybrid Lagrangian/Eulerian Collocated Advection and Projection Method for Fluid Simulation. ArXiv abs/2003.12227 (2020).Google Scholar
23. C. R. Graham and F. S. Henyey. 2000. Clebsch representation near points where the vorticity vanishes. Phys. Fluids 12 (2000), 744–746.Google ScholarCross Ref
24. P. He and Y. Yang. 2016. Construction of initial vortex-surface fields and Clebsch potentials for flows with high-symmetry using first integrals. Phys. Fluids 28 (2016), 037101.Google ScholarCross Ref
25. H. Hopf. 1931. Über die Abbildungen der Dreidimensionalen Sphäre auf die Kugelfläche. Math. Ann. 104 (1931), 637–665.Google ScholarCross Ref
26. J. Jeong and F. Hussain. 1995. On the identification of a vortex. J. Fluid. Mech. 285 (1995), 69–94.Google ScholarCross Ref
27. Chenfanfu Jiang, Craig Schroeder, Andrew Selle, Joseph Teran, and Alexey Stomakhin. 2015. The affine particle-in-cell method. ACM Transactions on Graphics (TOG) 34, 4 (2015), 1–10.Google ScholarDigital Library
28. Myungjoo Kang, Ronald P Fedkiw, and Xu-Dong Liu. 2000. A boundary condition capturing method for multiphase incompressible flow. Journal of Scientific Computing 15, 3 (2000), 323–360.Google ScholarDigital Library
29. H. Kedia, D. Foster, M. R. Dennis, and W. T. M. Irvine. 2016. Weaving knotted vector fields with tunable helicity. Phys. Rev. Lett. 117 (2016), 274501.Google ScholarCross Ref
30. ByungMoon Kim, Y. Liu, I. Llamas, and J. Rossignac. 2005. FlowFixer: Using BFECC for Fluid Simulation. In NPH.Google Scholar
31. Doyub Kim, Oh-Young Song, and Hyeongseok Ko. 2009. Stretching and wiggling liquids. ACM SIGGRAPH Asia 2009 papers (2009).Google Scholar
32. Theodore Kim, Nils Thürey, Doug James, and Markus Gross. 2008. Wavelet turbulence for fluid simulation. ACM Transactions on Graphics (TOG) 27, 3 (2008), 1–6.Google ScholarDigital Library
33. Dustin Kleckner and William TM Irvine. 2013. Creation and dynamics of knotted vortices. Nat. Phys. 9, 4 (2013), 253–258.Google ScholarCross Ref
34. P. Robert Kotiuga. 1991. Clebsch potentials and the visualization of three-dimensional solenoidal vector fields. IEEE T. MAGN 27 (1991), 3986–3989.Google ScholarCross Ref
35. Petros Koumoutsakos, Georges-Henri Cottet, and Diego Rossinelli. 2008. Flow simulations using particles-Bridging Computer Graphics and CFD. In SIGGRAPH 2008-35th International Conference on Computer Graphics and Interactive Techniques. ACM, 1–73.Google Scholar
36. G. A. Kuz’min. 1983. Ideal incompressible hydrodynamics in terms of the vortex momentum density. Phys. Lett. A 96 (1983), 88–90.Google ScholarCross Ref
37. H. Lamb. 1932. Hydrodynamics (6 ed.). Cambridge University Press.Google Scholar
38. A Leonard. 1980. Vortex methods for flow simulation. J. Comput. Phys. 37, 3 (1980), 289–335.Google ScholarCross Ref
39. TT Lim. 1989. An experimental study of a vortex ring interacting with an inclined wall. Exp. Fluids 7, 7 (1989), 453–463.Google ScholarCross Ref
40. TT Lim. 1997. A note on the leapfrogging between two coaxial vortex rings at low Reynolds numbers. Phys. Fluids 9, 1 (1997), 239–241.Google ScholarCross Ref
41. Miao’er Liu, Yu-Xin Ren, and Hanxin Zhang. 2004. A class of fully second order accurate projection methods for solving the incompressible Navier-Stokes equations. J. Comput. Phys. 200, 1 (2004), 325 — 346.Google ScholarDigital Library
42. John H. Maddocks and Robert L. Pego. 1995. An unconstrained Hamiltonian formulation for incompressible fluid flow. Comm. Math. Phys. 170, 1 (1995), 207–217.Google ScholarCross Ref
43. Patrick Mullen, Keenan Crane, Dmitry Pavlov, Yiying Tong, and Mathieu Desbrun. 2009. Energy-preserving integrators for fluid animation. ACM Transactions on Graphics (TOG) 28, 3 (2009), 1–8.Google ScholarDigital Library
44. V I Oseledets. 1989. On a new way of writing the Navier-Stokes equation. The Hamiltonian formalism. Russian Mathematical Surveys 44, 3 (jun 1989), 210–211.Google ScholarCross Ref
45. S. Park and M. Kim. 2005. Vortex fluid for gaseous phenomena. In SCA ’05.Google Scholar
46. Tobias Pfaff, Nils Thuerey, and Markus Gross. 2012. Lagrangian vortex sheets for animating fluids. ACM Transactions on Graphics (TOG) 31, 4 (2012), 1–8.Google ScholarDigital Library
47. Ziyin Qu, Xinxin Zhang, Ming Gao, Chenfanfu Jiang, and Baoquan Chen. 2019. Efficient and conservative fluids using bidirectional mapping. ACM Transactions on Graphics (TOG) 38, 4 (2019), 1–12.Google ScholarDigital Library
48. R. Saye. 2016. Interfacial gauge methods for incompressible fluid dynamics. Sci. Adv. 2 (2016), e1501869.Google ScholarCross Ref
49. Robert Saye. 2017a. Implicit mesh discontinuous Galerkin methods and interfacial gauge methods for high-order accurate interface dynamics, with applications to surface tension dynamics, rigid body fluid-structure interaction, and free surface flow: Part I. J. Comput. Phys. 344 (2017), 647 — 682.Google ScholarCross Ref
50. Robert Saye. 2017b. Implicit mesh discontinuous Galerkin methods and interfacial gauge methods for high-order accurate interface dynamics, with applications to surface tension dynamics, rigid body fluid-structure interaction, and free surface flow: Part II. J. Comput. Phys. 344 (2017), 683 — 723.Google ScholarDigital Library
51. A. Selle, Ronald Fedkiw, ByungMoon Kim, Y. Liu, and J. Rossignac. 2008. An Unconditionally Stable MacCormack Method. Journal of Scientific Computing 35 (2008), 350–371.Google ScholarDigital Library
52. Andrew Selle, Nick Rasmussen, and Ronald Fedkiw. 2005. A vortex particle method for smoke, water and explosions. In ACM SIGGRAPH 2005 Papers. 910–914.Google ScholarDigital Library
53. C. B. Smiet, S. Candelaresi, and D. Bouwmeester. 2017. Ideal relaxation of the Hopf fibration. Phys. Plasmas 24 (2017), 072110.Google ScholarCross Ref
54. C. B. Smiet, S. Candelaresi, A. Thompson, J. Swearngin, J.W. Dalhuisen, and D. Bouwmeester. 2015. Self-organizing knotted magnetic structures in plasma. Phys. Rev. Lett. 115 (2015), 095001.Google ScholarCross Ref
55. J. Stam. 1999. Stable fluids. In SIGGRAPH ’99.Google ScholarDigital Library
56. Mark J Stock, Werner JA Dahm, and Grétar Tryggvason. 2008. Impact of a vortex ring on a density interface using a regularized inviscid vortex sheet method. J. Comput. Phys. 227, 21 (2008), 9021–9043.Google ScholarDigital Library
57. D.M. Summers. 2000. A Representation of Bounded Viscous Flow Based on Hodge Decomposition of Wall Impulse. J. Comput. Phys. 158, 1 (2000), 28 — 50.Google ScholarDigital Library
58. D M Summers and A J Chorin. 1996. Numerical vorticity creation based on impulse conservation. Proceedings of the National Academy of Sciences 93, 5 (1996), 1881–1885.Google ScholarCross Ref
59. S. Weißmann and U. Pinkall. 2010. Filament-based smoke with vortex shedding and variational reconnection. ACM Trans. Graph. 29 (2010), 115.Google ScholarDigital Library
60. S. Xiong and Y. Yang. 2017. The boundary-constraint method for constructing vortex-surface fields. J. Comput. Phys. 339 (2017), 31–45.Google ScholarDigital Library
61. S. Xiong and Y. Yang. 2019. Identifying the tangle of vortex tubes in homogeneous isotropic turbulence. J. Fluid Mech. 874 (2019), 952–978.Google ScholarCross Ref
62. S. Xiong and Y. Yang. 2020. Evolution and helicity analysis of linked vortex tubes in viscous flows. Sci. Sin-Phys. Mech. Astron. 50 (2020), 040005.Google ScholarCross Ref
63. Y. Yang and D. I. Pullin. 2011. Evolution of vortex-surface fields in viscous Taylor-Green and Kida-Pelz flows. J. Fluid Mech. 685 (2011), 146–164.Google ScholarCross Ref
64. V. E. Zakharov and E. A. Kuznetsov. 1997. Hamiltonian formalism for nonlinear waves. Phys.-Usp. 40 (1997), 1087–1116.Google ScholarCross Ref
65. Xinxin Zhang, Robert Bridson, and Chen Greif. 2015. Restoring the missing vorticity in advection-projection fluid solvers. ACM Transactions on Graphics (TOG) 34, 4 (2015), 1–8.Google ScholarDigital Library
66. Y. Zhao, S. Xiong, Y. Yang, and S. Chen. 2018. Sinuous distortion of vortex surfaces in the lateral growth of turbulent spots. Phys. Rev. Fluids 3 (2018), 074701.Google ScholarCross Ref
67. Y. Zhao, Y. Yang, and S. Chen. 2016. Vortex reconnection in the late transition in channel flow. J. Fluid Mech. 802 (2016), R4.Google ScholarCross Ref
68. Yongning Zhu and Robert Bridson. 2005. Animating sand as a fluid. ACM Transactions on Graphics (TOG) 24, 3 (2005), 965–972.Google ScholarDigital Library