“Beyond developable: computational design and fabrication with auxetic materials”
Conference:
Type(s):
Title:
- Beyond developable: computational design and fabrication with auxetic materials
Session/Category Title: DEFORMABLE SURFACE DESIGN
Presenter(s)/Author(s):
Moderator(s):
Abstract:
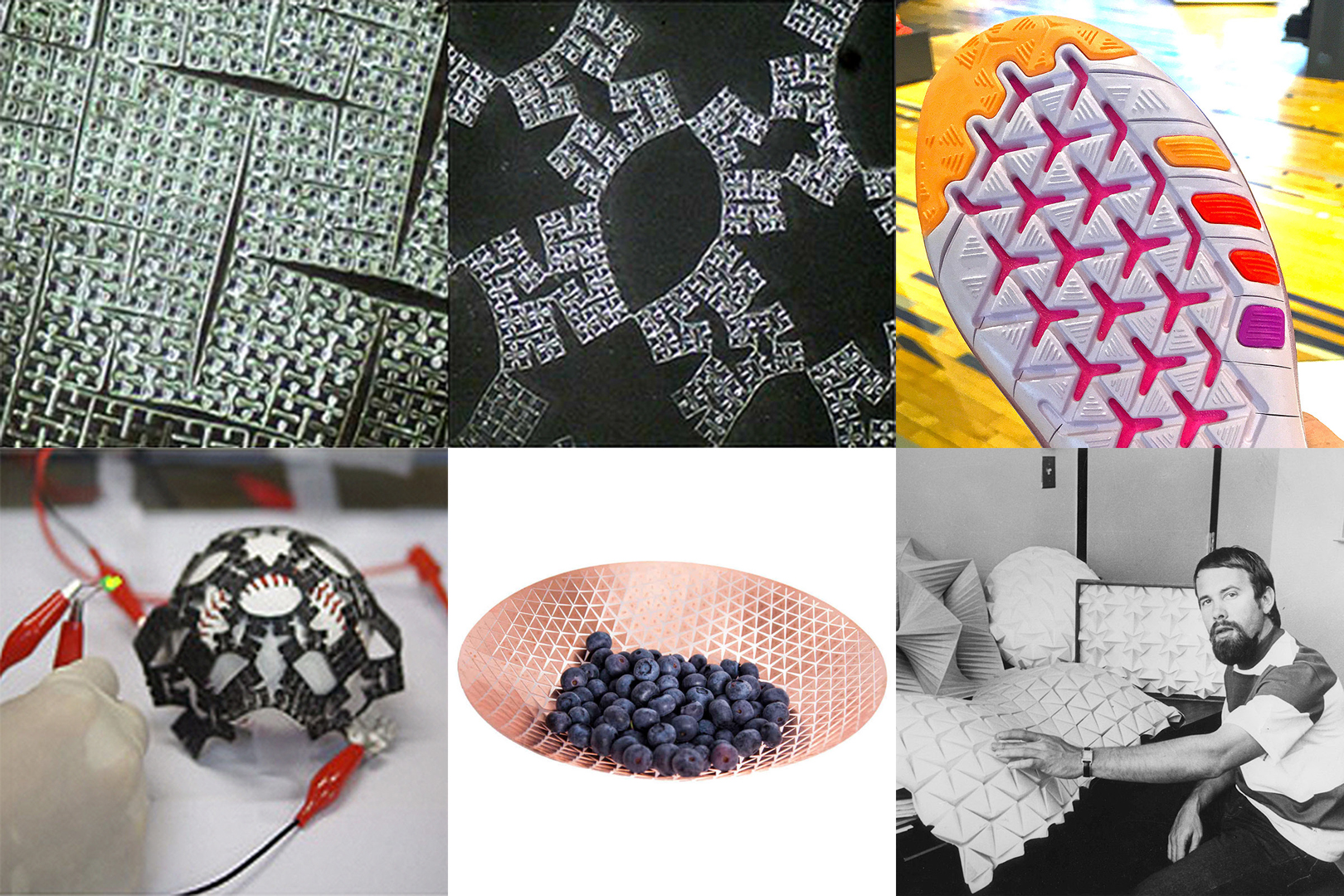
We present a computational method for interactive 3D design and rationalization of surfaces via auxetic materials, i.e., flat flexible material that can stretch uniformly up to a certain extent. A key motivation for studying such material is that one can approximate doubly-curved surfaces (such as the sphere) using only flat pieces, making it attractive for fabrication. We physically realize surfaces by introducing cuts into approximately inextensible material such as sheet metal, plastic, or leather. The cutting pattern is modeled as a regular triangular linkage that yields hexagonal openings of spatially-varying radius when stretched. In the same way that isometry is fundamental to modeling developable surfaces, we leverage conformal geometry to understand auxetic design. In particular, we compute a global conformal map with bounded scale factor to initialize an otherwise intractable non-linear optimization. We demonstrate that this global approach can handle non-trivial topology and non-local dependencies inherent in auxetic material. Design studies and physical prototypes are used to illustrate a wide range of possible applications.
References:
1. Aflalo, Y., Kimmel, R., and Zibulevsky, M. 2013. Conformal mapping with as uniform as possible conformal factor. SIAM Journal on Imaging Sciences 6, 1, 78–101.Google ScholarCross Ref
2. Bazant, M. Z., and Crowdy, D. 2005. Conformal Mapping Methods for Interfacial Dynamics.Google Scholar
3. Ben-Chen, M., and Gotsman, C. 2008. Characterizing shape using conformal factors. In Proceedings of the 1st Eurographics Conference on 3D Object Retrieval, 3DOR ’08, 1–8. Google ScholarDigital Library
4. Ben-Chen, M., Gotsman, C., and Bunin, G. 2008. Conformal flattening by curvature prescription and metric scaling. Computer Graphics Forum 27, 2, 449–458.Google ScholarCross Ref
5. Bobenko, A. I., and Suris, Y. B. 2008. Discrete Differential Geometry: Integrable Structure. AMS.Google Scholar
6. Bouaziz, S., Deuss, M., Schwartzburg, Y., Weise, T., and Pauly, M. 2012. Shape-up: Shaping discrete geometry with projections. Comput. Graph. Forum 31, 5, 1657–1667. Google ScholarDigital Library
7. Bouaziz, S., Martin, S., Liu, T., Kavan, L., and Pauly, M. 2014. Projective dynamics: Fusing constraint projections for fast simulation. ACM Trans. Graph. 33, 4 (July), 154:1–154:11. Google ScholarDigital Library
8. Caneparo, L. 2014. Digital Fabrication in Architecture, Engineering and Construction. Springer. Google ScholarDigital Library
9. Cho, Y., Shin, J.-H., Costa, A., Kim, T. A., Kunin, V., Li, J., Lee, S. Y., Yang, S., Han, H. N., Choi, I.-S., and Srolovitz, D. J. 2014. Engineering the shape and structure of materials by fractal cut. Proceedings of the National Academy of Sciences 111, 49, 17390–17395.Google ScholarCross Ref
10. Cignoni, P., Pietroni, N., Malomo, L., and Scopigno, R. 2014. Field-aligned mesh joinery. ACM Trans. Graph. 33, 1 (Feb.), 11:1–11:12. Google ScholarDigital Library
11. Crane, K., Pinkall, U., and Schröder, P. 2011. Spin transformations of discrete surfaces. ACM Trans. Graph. 30, 4 (July), 104:1–104:10. Google ScholarDigital Library
12. Crane, K., Pinkall, U., and Schröder, P. 2013. Robust fairing via conformal curvature flow. ACM Trans. Graph. 32, 4 (July), 61:1–61:10. Google ScholarDigital Library
13. Desbrun, M., Meyer, M., and Alliez, P. 2002. Intrinsic parameterizations of surface meshes. Computer Graphics Forum 21, 3, 209–218.Google ScholarCross Ref
14. Deuss, M., Deleuran, A., Bouaziz, S., Deng, B., Piker, D., and Pauly, M. 2015. ShapeOp — a robust and extensible geometric modelling paradigm. In Modelling Behaviour. Springer International Publishing, 505–515.Google Scholar
15. Evans, K. E., and Alderson, A. 2000. Auxetic materials: Functional materials and structures from lateral thinking! Advanced Materials 12, 9, 617–628.Google Scholar
16. Feeman, T. G. 2002. Portraits of the Earth: A Mathematician Looks at Maps. American Mathematical Society.Google Scholar
17. Garg, A., Sageman-Furnas, A. O., Deng, B., Yue, Y., Grinspun, E., Pauly, M., and Wardetzky, M. 2014. Wire mesh design. ACM Trans. Graph. 33, 4 (July), 66:1–66:12. Google ScholarDigital Library
18. Gatt, R., Mizzi, L., Azzopardi, J. I., Azzopardi, K. M., Attard, D., Casha, A., Briffa, J., and Grima, J. N. 2015. Hierarchical auxetic mechanical metamaterials. Scientific Reports 5.Google Scholar
19. Gibson, I., Rosen, D., and Stucker, B. 2015. Additive Manufacturing Technologies: 3D Printing, Rapid Prototyping, and Direct Digital Manufacturing, 2nd ed. Springer.Google Scholar
20. Gu, X., and Yau, S.-T. 2003. Global conformal surface parameterization. In Proc. SGP, 127–137. Google ScholarDigital Library
21. Gu, X. D., and Yau, S.-T. 2008. Computational conformal geometry. International Press of Boston, Inc.Google Scholar
22. Guo, R. 2011. Local rigidity of inversive distance circle packing. Transactions of the American Mathematical Society 363, 9, 4757–4776.Google ScholarCross Ref
23. Hildebrand, K., Bickel, B., and Alexa, M. 2012. crdbrd: Shape fabrication by sliding planar slices. Computer Graphics Forum 31, 2, 583–592. Google ScholarDigital Library
24. Iarussi, E., Li, W., and Bousseau, A. 2015. Wrapit: Computer-assisted crafting of wire wrapped jewelry. ACM Trans. Graph. 34, 6 (Oct.), 221:1–221:8. Google ScholarDigital Library
25. Igarashi, Y., Igarashi, T., and Mitani, J. 2012. Beady: Interactive beadwork design and construction. ACM Trans. Graph. 31, 4 (July), 49:1–49:9. Google ScholarDigital Library
26. Kharevych, L., Springborn, B., and Schröder, P. 2006. Discrete conformal mappings via circle patterns. ACM Trans. Graph. 25, 2 (Apr.), 412–438. Google ScholarDigital Library
27. Kilian, M., Flöry, S., Chen, Z., Mitra, N. J., Sheffer, A., and Pottmann, H. 2008. Curved folding. ACM Trans. Graph. 27, 3 (Aug.), 75:1–75:9. Google ScholarDigital Library
28. Kim, J., Hanna, J. A., Byun, M., Santangelo, C. D., and Hayward, R. C. 2012. Designing responsive buckled surfaces by halftone gel lithography. Science 335, 6073 (Mar.), 1201–1205.Google ScholarCross Ref
29. Lévy, B., Petitjean, S., Ray, N., and Maillot, J. 2002. Least squares conformal maps for automatic texture atlas generation. ACM Trans. Graph. 21, 3 (July), 362–371. Google ScholarDigital Library
30. Li, H., Adams, B., Guibas, L. J., and Pauly, M. 2009. Robust single-view geometry and motion reconstruction. ACM Trans. Graph. 28, 5 (Dec.), 175:1–175:10. Google ScholarDigital Library
31. Li, S., Zeng, W., Zhou, D., Gu, X. D., and Gao, J. 2013. Compact conformal map for greedy routing in wireless mobile sensor networks. In INFOCOM, IEEE, 2409–2417.Google Scholar
32. Lipman, Y., and Funkhouser, T. 2009. Möbius voting for surface correspondence. ACM Trans. Graph. 28, 3 (July), 72:1–72:12. Google ScholarDigital Library
33. Lipman, Y. 2012. Bounded distortion mapping spaces for triangular meshes. ACM Trans. Graph. 31, 4 (July), 108:1–108:13. Google ScholarDigital Library
34. Liu, Y., Pottmann, H., Wallner, J., Yang, Y.-L., and Wang, W. 2006. Geometric modeling with conical meshes and developable surfaces. ACM Trans. Graph. 25, 3 (July), 681–689. Google ScholarDigital Library
35. Luo, S.-J., Yue, Y., Huang, C.-K., Chung, Y.-H., Imai, S., Nishita, T., and Chen, B.-Y. 2015. Legolization: Optimizing lego designs. ACM Trans. Graph. 34, 6 (Oct.), 222:1–222:12. Google ScholarDigital Library
36. Mullen, P., Tong, Y., Alliez, P., and Desbrun, M. 2008. Spectral conformal parameterization. Computer Graphics Forum 27, 5, 1487–1494. Google ScholarDigital Library
37. Pérez, J., Thomaszewski, B., Coros, S., Bickel, B., Canabal, J. A., Sumner, R., and Otaduy, M. A. 2015. Design and fabrication of flexible rod meshes. ACM Trans. Graph. 34, 4 (July), 138:1–138:12. Google ScholarDigital Library
38. Pottmann, H., Eigensatz, M., Vaxman, A., and Wallner, J. 2015. Architectural geometry. Computers & Graphics 47, 145–164. Google ScholarDigital Library
39. Rafsanjani, A., and Pasini, D. 2016. Multistable compliant auxetic metamaterials inspired by geometric patterns in Islamic arts. APS March Meeting.Google Scholar
40. Resch, R. D. 1973. The topological design of sculptural and architectural systems. In Proceedings of the June 4-8, 1973, National Computer Conference and Exposition, ACM, New York, NY, USA, AFIPS ’73, 643–650. Google ScholarDigital Library
41. Röhrig, T., Sechelmann, S., Kycia, A., and Fleischmann, M. 2014. Surface panelization using periodic conformal maps. In Advances in Architectural Geometry 2014. Springer International Publishing, 199–214.Google Scholar
42. Schiftner, A., Höbinger, M., Wallner, J., and Pottmann, H. 2009. Packing circles and spheres on surfaces. ACM Trans. Graph. 28, 5 (Dec.), 139:1–139:8. Google ScholarDigital Library
43. Schwartzburg, Y., and Pauly, M. 2013. Fabrication-aware design with intersecting planar pieces. Computer Graphics Forum 32, 2, 317–326.Google ScholarCross Ref
44. Skouras, M., Thomaszewski, B., Kaufmann, P., Garg, A., Bickel, B., Grinspun, E., and Gross, M. 2014. Designing inflatable structures. ACM Trans. Graph. 33, 4 (July), 63:1–63:10. Google ScholarDigital Library
45. Song, P., Fu, C.-W., Goswami, P., Zheng, J., Mitra, N. J., and Cohen-Or, D. 2013. Reciprocal frame structures made easy. ACM Trans. Graph. 32, 4 (July), 94:1–94:13. Google ScholarDigital Library
46. Springborn, B., Schröder, P., and Pinkall, U. 2008. Conformal equivalence of triangle meshes. ACM Trans. Graph. 27, 3 (Aug.), 77:1–77:11. Google ScholarDigital Library
47. Stephenson, K. 2003. Circle packing: a mathematical tale. Notices of the AMS 50, 11, 1376–1388.Google Scholar
48. Sullivan, J. M. 2011. Conformal tiling on a torus. In Proceedings of Bridges 2011: Mathematics, Music, Art, Architecture, Culture, Tessellations Publishing, R. Sarhangi and C. H. Séquin, Eds., 593–596.Google Scholar
49. Tachi, T. 2010. Origamizing polyhedral surfaces. IEEE Trans. Vis. Comput. Graph. 16, 2, 298–311. Google ScholarDigital Library
50. Tachi, T. 2013. Freeform origami tessellations by generalizing Resch’s patterns. Journal of Mechanical Design 135, 11.Google ScholarCross Ref
51. Tang, C., Bo, P., Wallner, J., and Pottmann, H. 2016. Interactive design of developable surfaces. ACM Trans. Graph. 35, 2 (Jan.), 12:1–12:12. Google ScholarDigital Library
52. Testuz, R., Schwartzburg, Y., and Pauly, M. 2013. Automatic generation of constructable brick sculptures. In Proc. Eurographics.Google Scholar
53. Vaxman, A., Müller, C., and Weber, O. 2015. Conformal mesh deformations with Möbius transformations. ACM Trans. Graph. 34, 4 (July), 55:1–55:11. Google ScholarDigital Library
54. Weber, O., Myles, A., and Zorin, D. 2012. Computing extremal quasiconformal maps. Computer Graphics Forum 31, 5, 1679–1689. Google ScholarDigital Library
55. Zimmer, H., and Kobbelt, L. 2014. Zometool rationalization of freeform surfaces. Visualization and Computer Graphics, IEEE Transactions on 20, 10 (Oct), 1461–1473.Google Scholar