“An inversion algorithm for geometric models” by Mantyla
Conference:
Type(s):
Title:
- An inversion algorithm for geometric models
Presenter(s)/Author(s):
Abstract:
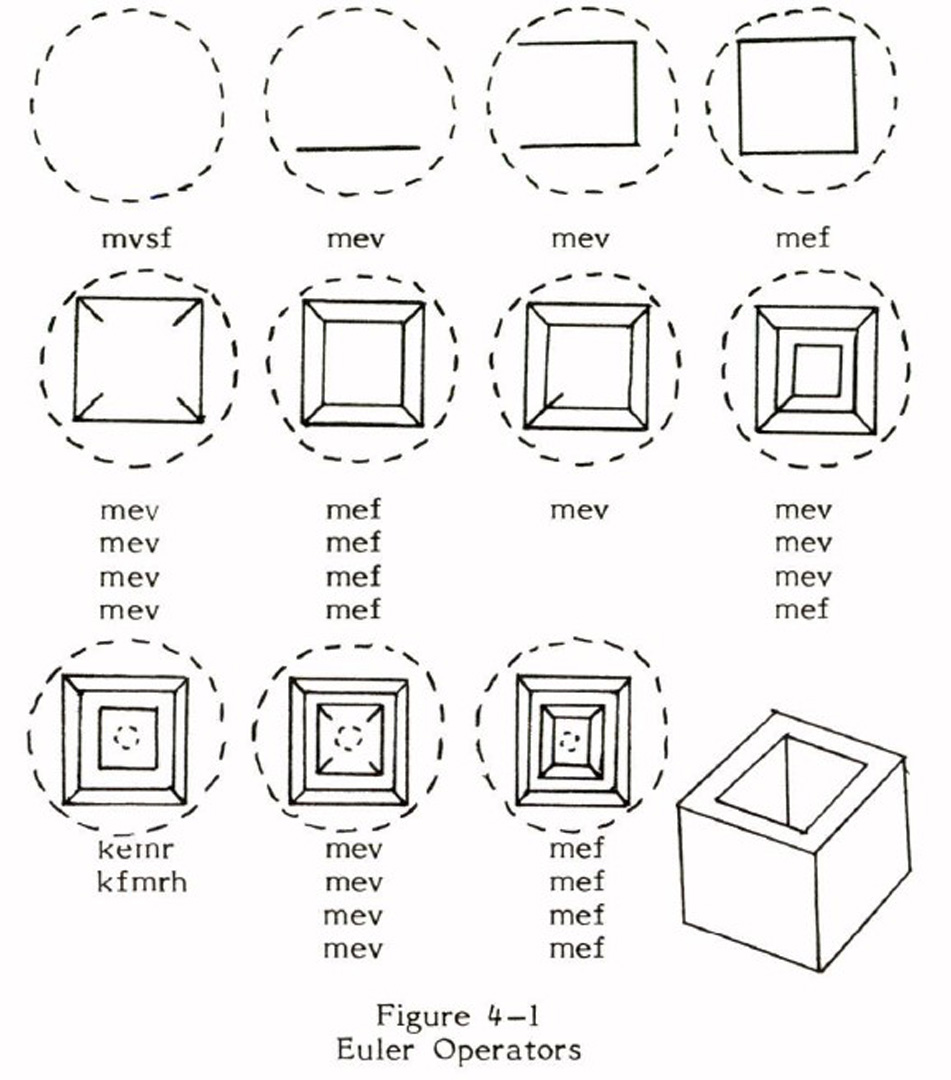
Instead of storing boundary models of solids directly into a data base, it would be advantageous to map them first into a simpler form. This approach calls for a procedure called in this paper the inversion algorithm of a geometric model. We present and analyze an inversion algorithm which constructs a sequence of Euler Operators capable of creating a given boundary representation. The algorithm is completely based on the use of Euler Operators enabling us to keep the algorithm simple and to hide implementation and data structure details.
References:
1. Baer, A., C.M.Eastman and M.Henrion: “Geometric Modeling: A Survey”, Computer Aided Design, Vol. 11, No. 5 (1979), pp. 253-272
2. Baumgart, B.G.: “Geometric modeling for computer vision”, Ph.D. Thesis, Rep. No. CS-463, Computer Science Dept., Stanford Univ. (1974)
3. Baumgart, B.G.: “A polyhedron representation for computer vision”, AFIPS Conf. Proc. Vol. 44, National Computer Conf. (1975), pp. 589-596
4. Braid, I.C., R.C.Hillyard and I.A.Stroud: “Stepwise Construction of Polyhedra in Geometric Modeling”, in: Brodlie, K.W.: “Mathematical Methods in Computer Graphics and Design”, Academic Press, New York (1980), pp. 123-141
5. Eastman, C.M. and K.Weiler: “Geometric Modeling Using the Euler Operators”, Res. Report No. 78, Institute of Physical Planning, Carnegie-Mellon Univ. (1979)
6. Mäntylä, M.: “Methodological Background of the Geometric Workbench”, Report No. HTKK-TKO-B30, Laboratory of Inf. Proc. Science, Helsinki University of Technology (1981)
7. Mäntylä, M. and R.Sulonen: “GWB – A Solid Modeller With Euler Operators”, to appear in IEEE Computer Graphics & Applications
8. Mäntylä, M. and T.Takala: “The Geometric Workbench (GWB) – an Experimental Geometric Modeling System”, in: Encarnacao, J. (ed.): “EUROGRAPHICS ’81”, North-Holland, Amsterdam (1981), pp. 205-215
9. Requicha, A.A.G.: “Representations of Rigid Solids – Theory, Methods, and Systems”, ACM Computing Surveys, Vol. 12 No. 4 (1980), pp. 437-464
10. Requicha, A.A.G. and H.B.Voelcker: “Constructive Solid Geometry”, Production Automation Project, Tech. Memo. No. 25, Univ. of Rochester (1977)