“A simple manifold-based construction of surfaces of arbitrary smoothness” by Ying and Zorin
Conference:
Type(s):
Title:
- A simple manifold-based construction of surfaces of arbitrary smoothness
Presenter(s)/Author(s):
Abstract:
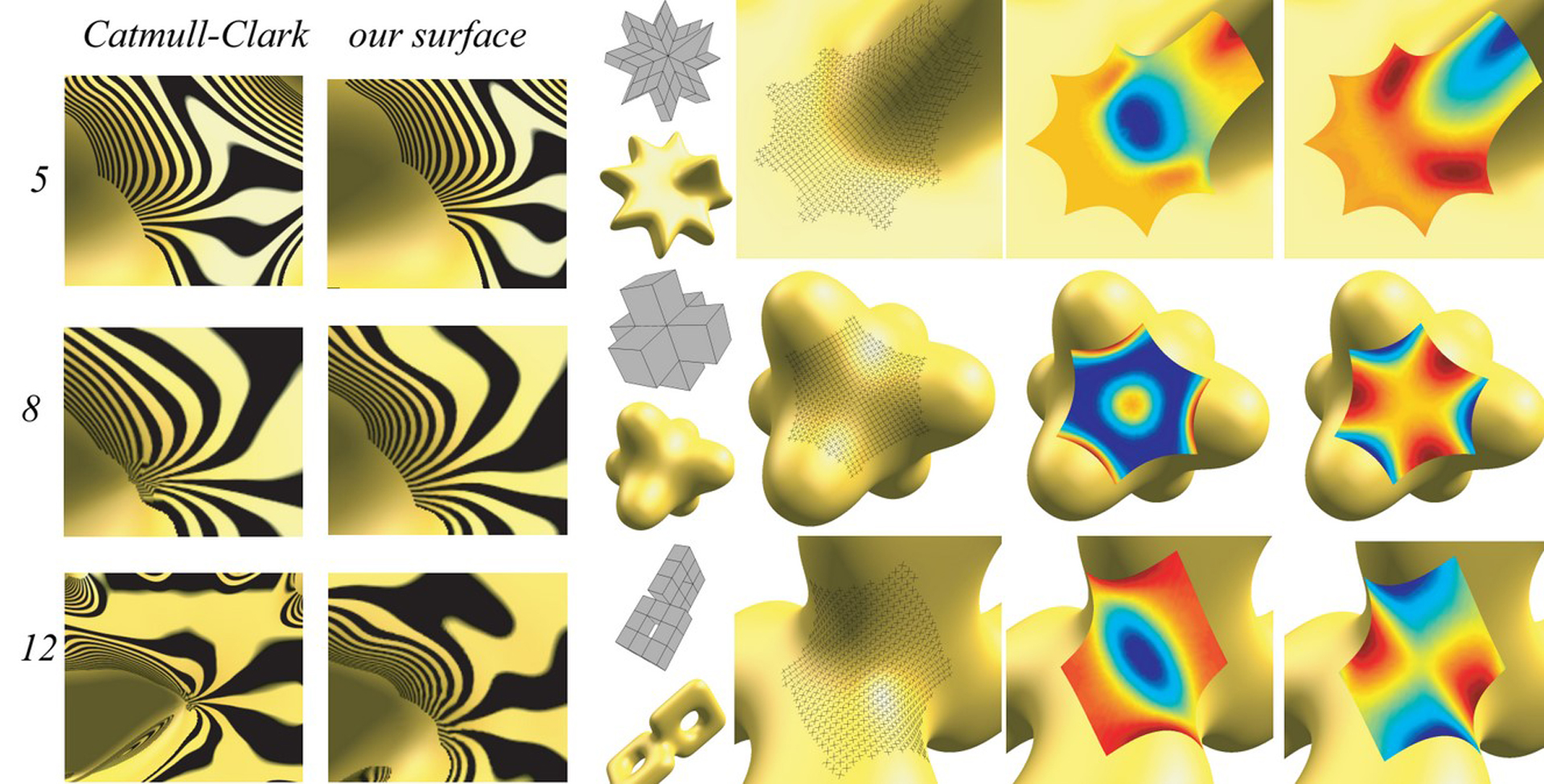
We present a smooth surface construction based on the manifold approach of Grimm and Hughes. We demonstrate how this approach can relatively easily produce a number of desirable properties which are hard to achieve simultaneously with polynomial patches, subdivision or variational surfaces. Our surfaces are C∞-continuous with explicit nonsingular C∞ parameterizations, high-order flexible at control vertices, depend linearly on control points, have fixed-size local support for basis functions, and have good visual quality.
References:
1. BOHL, H., AND REIF, U. 1997. Degenerate Bézier patches with continuous curvature. Comput. Aided Geom. Design 14, 8, 749–761. Google ScholarDigital Library
2. BRUNO, O. P., AND KUNYANSKY, L. A. 2001. A fast, high-order algorithm for the solution of surface scattering problems: basic implementation, tests, and applications. J. Comput. Phys. 169, 1, 80–110. Google ScholarDigital Library
3. DUCHAMP, T., CERTAIN, A., DEROSE, A., AND STUETZLE, W. 1997. Hierarchical computation of pl harmonic embeddings. Tech. rep., University of Washington.Google Scholar
4. GREGORY, J. A., AND HAHN, J. M. 1989. A Cspan2 polygonal surface patch. Comput. Aided Geom. Design 6, 1, 69–75. Google ScholarDigital Library
5. GRIMM, C. M., AND HUGHES, J. F. 1995. Modeling surfaces of arbitrary topology using manifolds. In Proceedings of SIGGRAPH 95, Computer Graphics Proceedings, Annual Conference Series, 359–368. Google ScholarDigital Library
6. GRIMM, C. M., AND HUGHES, J. F. 2003. Parameterizating n-holed tori. In The Mathematics of Surfaces IX.Google Scholar
7. GRIMM, C. M. 2002. Simple manifolds for surface modeling and parameterization. In Shape Modeling International. Google ScholarDigital Library
8. GU, X., AND YAU, S.-T. 2003. Global conformal surface parameterization. In Proceedings of the Eurographics/ACM SIGGRAPH symposium on Geometry processing, Eurographics Association, 127–137. Google ScholarDigital Library
9. HERMANN, T. 1996. G2 interpolation of free form curve networks by biquintic Gregory patches. Comput. Aided Geom. Design 13, 9, 873–893. In memory of John Gregory. Google ScholarDigital Library
10. JAMES, D. L., AND PAI, D. K. 1999. Artdefo: accurate real time deformable objects. In Proceedings of the 26th annual conference on Computer graphics and interactive techniques, ACM Press/Addison-Wesley Publishing Co., 65–72. Google ScholarDigital Library
11. LOOP, C. T., AND DEROSE, T. D. 1989. A multisided generalization of bézier surfaces. ACM Trans. Graph. 8, 3, 204–234. Google ScholarDigital Library
12. NAVAU, J. C., AND GARCIA, N. P. 2000. Modeling surfaces from meshes of arbitrary topology. Comput. Aided Geom. Design 17, 7, 643–671. Google ScholarDigital Library
13. PETERS, J. 1996. Curvature continuous spline surfaces over irregular meshes. Comput. Aided Geom. Design 13, 2, 101–131. Google ScholarDigital Library
14. PETERS, J. 2000. Patching Catmull-Clark meshes. In Proceedings of ACM SIGGRAPH 2000, Computer Graphics Proceedings, Annual Conference Series, 255–258. Google ScholarDigital Library
15. PETERS, J. 2002. C2 free-form surfaces of degree (3, 5). Comput. Aided Geom. Design 19, 2, 113–126. Google ScholarDigital Library
16. PRAUTZSCH, H. 1997. Freeform splines. Comput. Aided Geom. Design 14, 3, 201–206. Google ScholarDigital Library
17. REIF, U. 1998. TURBS—topologically unrestricted rational B-splines. Constr. Approx. 14, 1, 57–77.Google ScholarCross Ref
18. SEIDEL, H.-P. 1994. Polar forms and triangular B-Spline surfaces. In Euclidean Geometry and Computers, 2nd Edition, D.-Z. Du and F. Hwang, Eds. World Scientific Publishing Co., 235–286.Google Scholar
19. STAM, J. 1998. Exact evaluation of Catmull-Clark subdivision surfaces at arbitrary parameter values. In Proceedings of SIGGRAPH 98, ACM SIGGRAPH / Addison Wesley, Orlando, Florida, Computer Graphics Proceedings, Annual Conference Series, 395–404. ISBN 0-89791-999-8. Google ScholarDigital Library
20. STAM, J. 2003. Flows on surfaces of arbitrary topology. ACM Trans. Graph. 22, 3, 724–731. Google ScholarDigital Library
21. WANG, T. J. 1992. A C2-quintic spline interpolation scheme on triangulation. Comput. Aided Geom. Design 9, 5, 379–386. Google ScholarDigital Library
22. WARREN, J., AND WEIMER, H. 2001. Subdivision Methods for Geometric Design. Morgan Kaufmann. Google ScholarDigital Library
23. ZORIN, D., SCHRÖDER, P., DEROSE, A., KOBBELT, L., LEVIN, A., AND SWELDENS., W., 2001. Subdivision for modeling and animation. SIGGRAPH 2001 Course Notes.Google Scholar