“Worst-case stress relief for microstructures” by Panetta, Rahimian and Zorin
Conference:
Type(s):
Title:
- Worst-case stress relief for microstructures
Session/Category Title: Fabricating Look & Feel
Presenter(s)/Author(s):
Moderator(s):
Abstract:
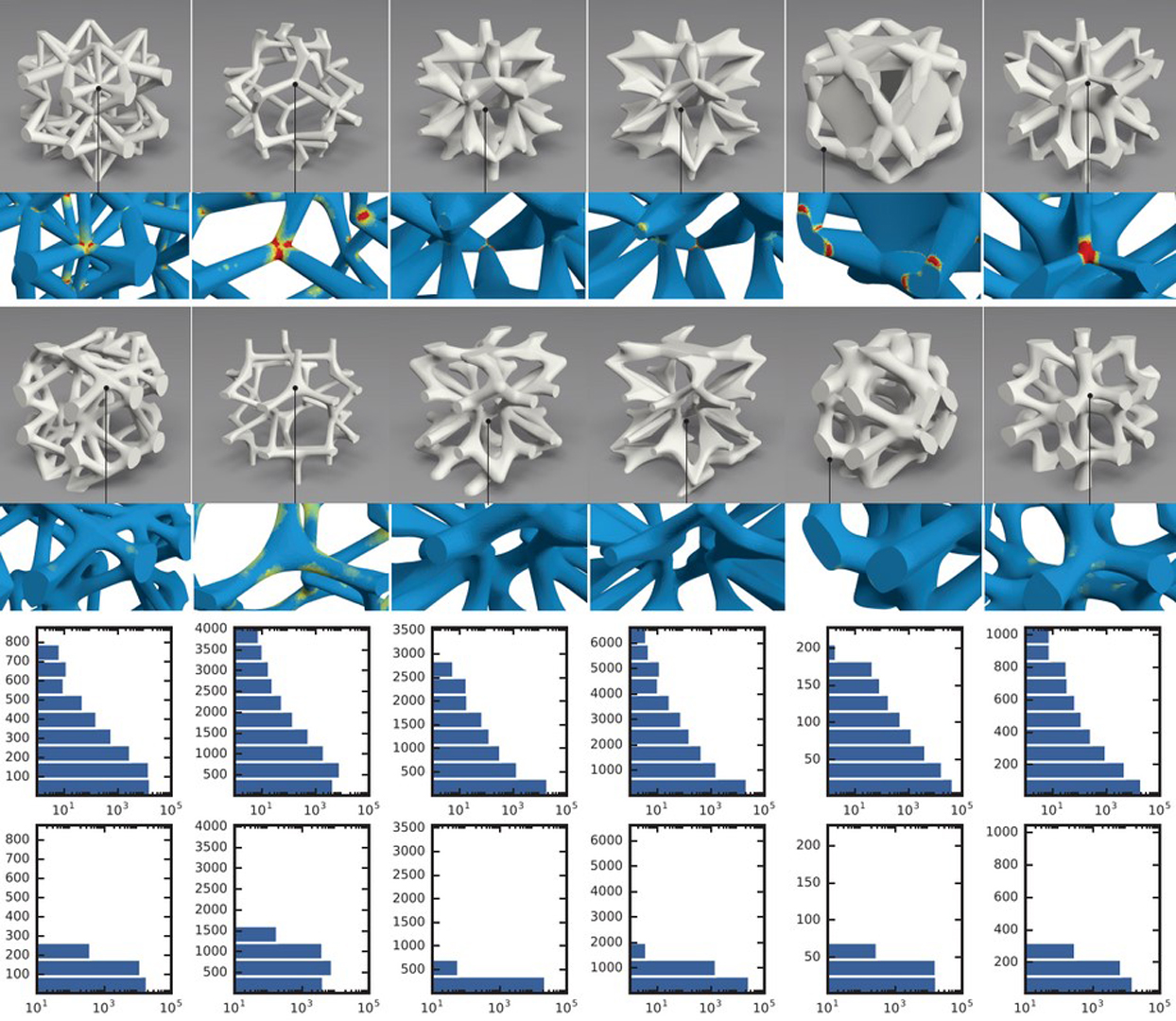
Additive fabrication technologies are limited by the types of material they can print: while the technologies are continuously improving, still only a relatively small discrete set of materials can be used in each printed object. At the same time, the low cost of introducing geometric complexity suggests the alternative of controlling the elastic material properties by producing microstructures, which can achieve behaviors significantly differing from the solid printing material. While promising results have been obtained in this direction, fragility is a significant problem blocking practical applications, especially for achieving soft material properties: due to stress concentrations at thin joints, deformations and repeated loadings are likely to cause fracture.We present a set of methods to minimize stress concentrations in microstructures by evolving their shapes. First, we demonstrate that the worst-case stress analysis problem (maximizing a stress measure over all possible unit loads) has an exact solution for periodic microstructures. We develop a new, accurate discretization of the shape derivative for stress objectives and introduce a low-dimensional parametric shape model for microstructures. This model supports robust minimization of maximal stress (approximated by an Lp norm with high p) and an efficient implementation of printability constraints. In addition to significantly reducing stresses (by a typical factor of 5X), the new method substantially expands the range of effective material properties covered by the collection of structures.
References:
1. G. Allaire. 2002. Shape optimization by the homogenization method. Vol. 146. Springer. Google ScholarCross Ref
2. G. Allaire and F. Jouve. 2008. Minimum stress optimal design with the level set method. Engineering Analysis with Boundary Elements 32, 11 (2008), 909 — 918. Shape and Topological Sensitivity Analysis: Theory and Applications. Google ScholarCross Ref
3. G. Allaire, F. Jouve, and H. Maillot. 2004. Topology optimization for minimum stress design with the homogenization method. Structural and Multidisciplinary Optimization 28, 2–3 (2004), 87–98.Google ScholarCross Ref
4. P. Alliez, C. Jamin, L. Rineau, S. Tayeb, J. Tournois, and M. Yvinec. 2016. 3D Mesh Generation. In CGAL User and Reference Manual (4.9 ed.). CGAL Editorial Board. http://doc.cgal.org/4.9/Manual/packages.html#PkgMesh_3SummaryGoogle Scholar
5. E. Andreassen, B. S. Lazarov, and O. Sigmund. 2014. Design of manufacturable 3D extremal elastic microstructure. Mechanics of Materials 69, 1 (2014), 1–10. Google ScholarCross Ref
6. L. Barthe, B. Wyvill, and E. De Groot. 2004. Controllable Binary CSG operators for “soft objects”. International Journal of Shape Modeling 10, 02 (2004), 135–154. Google ScholarCross Ref
7. M. P. Bendsøe. 1989. Optimal shape design as a material distribution problem. Structural optimization 1, 4 (1989), 193–202. Google ScholarCross Ref
8. M. P. Bendsøe and O. Sigmund. 2003. Topology optimization: theory, methods and applications. Springer.Google Scholar
9. A. Bernhardt, L. Barthe, M.-P. Cani, and B. Wyvill. 2010. Implicit blending revisited. In Computer Graphics Forum, Vol. 29. Wiley Online Library, 367–375. Google ScholarCross Ref
10. B. Bickel, M. Bächer, M. A. Otaduy, H. R. Lee, H. Pfister, M. Gross, and W. Matusik. 2010. Design and Fabrication of Materials with Desired Deformation Behavior. ACM Trans. Graph. 29, 4, Article 63 (July 2010), 10 pages. Google ScholarDigital Library
11. J. F. Blinn. 1982. A generalization of algebraic surface drawing. ACM transactions on graphics (TOG) 1, 3 (1982), 235–256.Google Scholar
12. J. Bloomenthal. 1997. Bulge elimination in convolution surfaces. In Computer Graphics Forum, Vol. 16. Wiley Online Library, 31–41. Google ScholarCross Ref
13. T. Bückmann, N. Stenger, M. Kadic, J. Kaschke, A. Frölich, T. Kennerknecht, C. Eberl, M. Thiel, and M. Wegener. 2012. Tailored 3D Mechanical Metamaterials Made by Dip-in Direct-Laser-Writing Optical Lithography. Advanced Materials 24, 20 (2012), 2710–2714. Google ScholarCross Ref
14. A. Cherkaev. 2000. Variational methods for structural optimization. Vol. 140. Springer. Google ScholarCross Ref
15. D. Cioranescu and P. Donato. 1999. An introduction to homogenization. Oxford University Press.Google Scholar
16. A. T. Gaynor and J. K. Guest. 2016. Topology optimization considering overhang constraints: Eliminating sacrificial support material in additive manufacturing through design. Structural and Multidisciplinary Optimization 54, 5 (2016), 1157–1172. Google ScholarDigital Library
17. O. Gourmel, L. Barthe, M.-P. Cani, B. Wyvill, A. Bernhardt, M. Paulin, and H. Grasberger. 2013. A gradient-based implicit blend. ACM Transactions on Graphics (TOG) 32, 2 (2013), 12.Google ScholarDigital Library
18. G. N. Greaves, A. L. Greer, R. S. Lakes, and T. Rouxel. 2011. Poisson’s ratio and modern materials. Nature Materials 10, 11 (2011), 823–837. Google ScholarCross Ref
19. Z. Hashin and S. Shtrikman. 1963. A variational approach to the theory of the elastic behaviour of multiphase materials. Journal of the Mechanics and Physics of Solids 11, 2 (1963), 127–140. Google ScholarCross Ref
20. J. Hiller and H. Lipson. 2009. Design and analysis of digital materials for physical 3D voxel printing. Rapid Prototyping Journal 15, 2 (2009), 137–149. Google ScholarCross Ref
21. S. J. Hollister. 2005. Porous scaffold design for tissue engineering. Nature Materials 4, 7 (2005), 518–524. Google ScholarCross Ref
22. S. G. Johnson. 2016. The NLopt nonlinear-optimization package. (2016). http://ab-initio.mit.edu/nloptGoogle Scholar
23. H. S. Kang. 2010. Hierarchical design and simulation of tissue engineering scaffold mechanical, mass transport, and degradation properties. Ph.D. Dissertation. The University of Michigan.Google Scholar
24. L. Kharevych, P. Mullen, H. Owhadi, and M. Desbrun. 2009. Numerical Coarsening of Inhomogeneous Elastic Materials. ACM Trans. Graph. 28, 3, Article 51 (July 2009), 8 pages. Google ScholarDigital Library
25. D. Kraft. 1994. Algorithm 733: TOMP-Fortran modules for optimal control calculations. ACM Transactions on Mathematical Software (TOMS) 20, 3 (1994), 262–281. Google ScholarDigital Library
26. G. Kreisselmeier and R. Steinhauser. 1983. Application of vector performance optimization to a robust control loop design for a fighter aircraft. Internat. J. Control 37, 2 (1983), 251–284. Google ScholarCross Ref
27. M. Langelaar. 2016. An additive manufacturing filter for topology optimization of print-ready designs. Structural and Multidisciplinary Optimization (2016), 1–13.Google Scholar
28. T. Langlois, A. Shamir, D. Dror, W. Matusik, and D. I. Levin. 2016. Stochastic structural analysis for context-aware design and fabrication. ACM Transactions on Graphics (TOG) 35, 6 (2016), 226.Google ScholarDigital Library
29. H. Lian, A. N. Christiansen, D. A. Tortorelli, O. Sigmund, and N. Aage. 2017. Combined shape and topology optimization for minimization of maximal von Mises stress. Structural and Multidisciplinary Optimization 55, 5 (2017), 1541–1557. Google ScholarDigital Library
30. C.-Y. Lin, C.-C. Hsiao, P.-Q. Chen, and S. J. Hollister. 2004a. Interbody fusion cage design using integrated global layout and local microstructure topology optimization. Spine 29, 16 (2004), 1747–1754. PMID: 15303018. Google ScholarCross Ref
31. C. Y. Lin, N. Kikuchi, and S. J. Hollister. 2004b. A novel method for biomaterial scaffold internal architecture design to match bone elastic properties with desired porosity. Journal of Biomechanics 37, 5 (2004), 623–636. Google ScholarCross Ref
32. H. Lin, Y. Xiong, and H. Liao. 2014. Semi-structured B-spline for blending two B-spline surfaces. Computers & Mathematics with Applications 68, 7 (2014), 706–718. Google ScholarDigital Library
33. J. Martínez, J. Dumas, and S. Lefebvre. 2016. Procedural Voronoi foams for additive manufacturing. ACM Transactions on Graphics (TOG) 35, 4 (2016), 44.Google ScholarDigital Library
34. G. W. Milton. 2002. The theory of composites. Cambridge University Press. Google ScholarCross Ref
35. P. Nakasone and E. Silva. 2010. Dynamic design of piezoelectric laminated sensors and actuators using topology optimization. Journal of Intelligent Material Systems and Structures 21, 16 (2010), 1627–1652. Google ScholarCross Ref
36. J. Panetta, Q. Zhou, L. Malomo, N. Pietroni, P. Cignoni, and D. Zorin. 2015. Elastic textures for additive fabrication. ACM Transactions on Graphics (TOG) 34, 4 (2015), 135.Google ScholarDigital Library
37. A. Pasko and V. Adzhiev. 2002. Function-based shape modeling: mathematical framework and specialized language. In International Workshop on Automated Deduction in Geometry. Springer, 132–160.Google Scholar
38. G. I. Pasko, A. A. Pasko, and T. L. Kunii. 2005. Bounded blending for function-based shape modeling. IEEE Computer Graphics and Applications 25, 2 (2005), 36–45. Google ScholarDigital Library
39. M. Polajnar, F. Kosel, and R. Drazumeric. 2017. Structural optimization using global stress-deviation objective function via the level-set method. Structural and Multidisciplinary Optimization 55, 1 (2017), 91–104. Google ScholarDigital Library
40. X. Qian. 2016. Undercut and overhang angle control in topology optimization: a density gradient based integral approach. Internat. J. Numer. Methods Engrg. (2016).Google Scholar
41. I. Quilez. 2013. Smooth minimum. (2013). http://www.iquilezles.org/www/articles/smin/smin.htmGoogle Scholar
42. A. P. Rockwood. 1989. The displacement method for implicit blending surfaces in solid models. ACM Transactions on Graphics (TOG) 8, 4 (1989), 279–297. Google ScholarDigital Library
43. M. Sabin. 1968. The use of potential surfaces for numerical geometry. British Aircraft Corporation, Weybridge, UK, Technical Report No. VTO/MS/153 (1968).Google Scholar
44. C. Schumacher, B. Bickel, J. Rys, S. Marschner, C. Daraio, and M. Gross. 2015. Microstructures to control elasticity in 3D printing. ACM Transactions on Graphics (TOG) 34, 4 (2015), 136.Google ScholarDigital Library
45. J. Schwerdtfeger, F. Wein, G. Leugering, R. F. Singer, C. Körner, M. Stingl, and F. Schury. 2011. Design of Auxetic Structures via Mathematical Optimization. Advanced Materials 23, 22 (2011), 2650–2654. Google ScholarCross Ref
46. M. Skouras, B. Thomaszewski, S. Coros, B. Bickel, and M. Gross. 2013. Computational design of actuated deformable characters. ACM Transactions on Graphics (TOG) 32, 4 (2013), 82.Google ScholarDigital Library
47. F. Sonmez. 2009. Optimal shape design of shoulder fillets for flat and round bars under various loadings. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science 223, 8 (2009), 1741–1754. Google ScholarCross Ref
48. O. Stava, J. Vanek, B. Benes, N. Carr, and R. Mvech. 2012. Stress Relief: Improving Structural Strength of 3D Printable Objects. ACM Trans. Graph. 31, 4, Article 48 (July 2012), 11 pages.Google ScholarDigital Library
49. S. Torquato. 2002. Random heterogeneous materials: microstructure and macroscopic properties. Vol. 16. Springer. Google ScholarCross Ref
50. L. Van Miegroet and P. Duysinx. 2007. Stress concentration minimization of 2D filets using X-FEM and level set description. Structural and Multidisciplinary Optimization 33, 4–5 (2007), 425–438.Google ScholarCross Ref
51. J. Vida, R. R. Martin, and T. Varady. 1994. A survey of blending methods that use parametric surfaces. Computer-Aided Design 26, 5 (1994), 341–365. Google ScholarCross Ref
52. Q. Xia, T. Shi, S. Liu, and M. Y. Wang. 2012. A level set solution to the stress-based structural shape and topology optimization. Computers & Structures 90 (2012), 55–64. Google ScholarDigital Library
53. C. Zanni, A. Bernhardt, M. Quiblier, and M.-P. Cani. 2013. SCALe-invariant Integral Surfaces. In Computer Graphics Forum, Vol. 32. Wiley Online Library, 219–232. Google ScholarCross Ref
54. C. Zanni, M. Gleicher, and M.-P. Cani. 2015. N-ary implicit blends with topology control. Computers & Graphics 46 (2015), 1–13. Google ScholarDigital Library
55. H. Zhao, W. Xu, K. Zhou, Y. Yang, X. Jin, and H. Wu. 2016. Stress-Constrained Thickness Optimization for Shell Object Fabrication. In Computer Graphics Forum. Wiley Online Library. Google ScholarDigital Library
56. Q. Zhou, J. Panetta, and D. Zorin. 2013. Worst-case structural analysis. ACM Trans. Graph. 32, 4, Article 137 (July 2013), 12 pages.Google ScholarDigital Library
57. Y. Zhou, E. Kalogerakis, R. Wang, and I. R. Grosse. 2016. Direct shape optimization for strengthening 3D printable objects. In Computer Graphics Forum, Vol. 35. Wiley Online Library, 333–342.Google Scholar