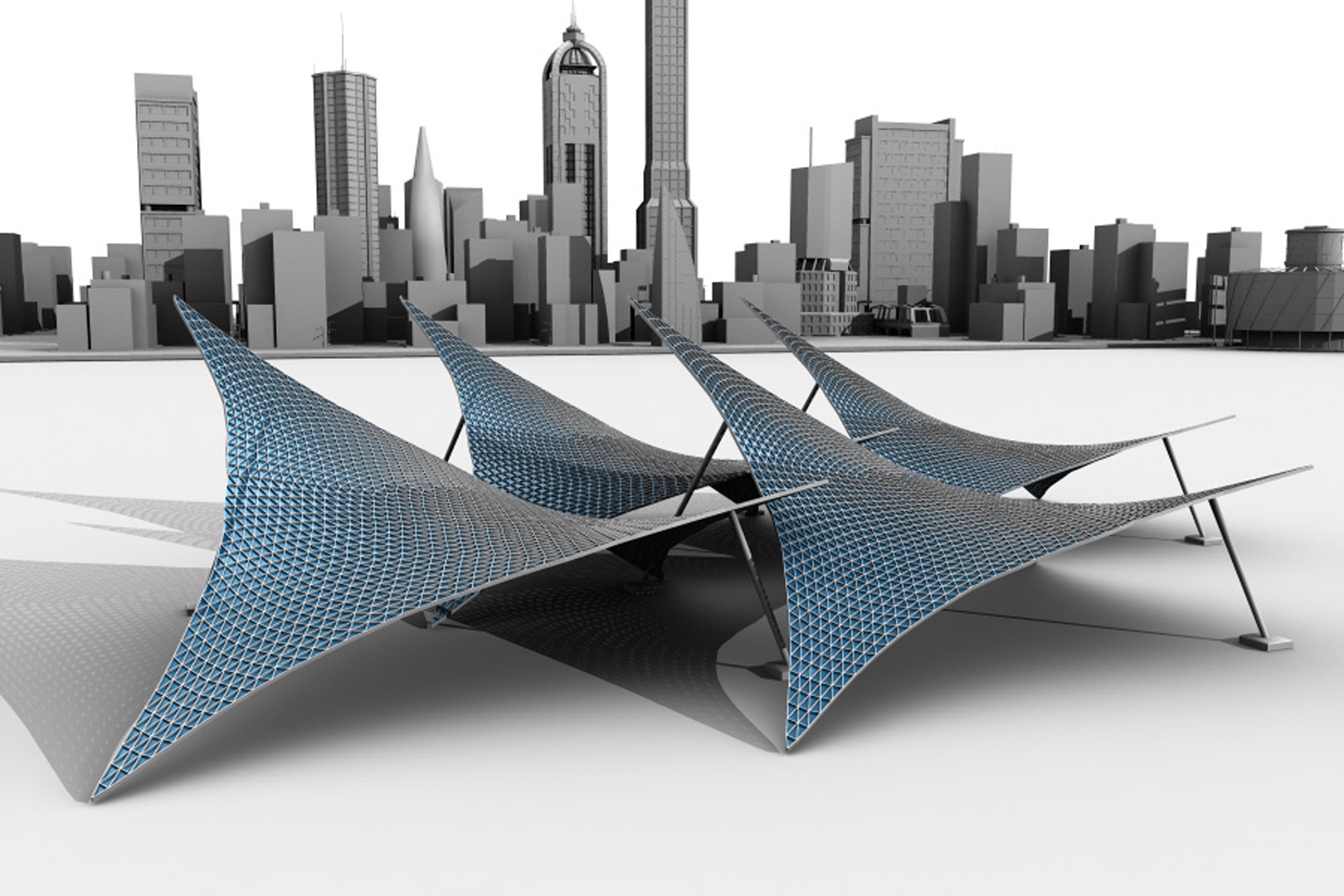
“Triangle surfaces with discrete equivalence classes” by Singh and Schaefer

We propose a technique that takes a triangulated surface as input and outputs a surface with the same topology but altered geometry such that each polygon falls into a set of discrete equivalence classes. We begin by describing an error function that measures how close the polygons are to satisfying this criteria. To optimize this error function, we first cluster triangles into discrete sets such that the assignment of sets minimizes our error. We then find canonical polygons for each set using nonlinear optimization. Next, we solve a Poisson equation to find positions of vertices such that the surface polygons match the canonical polygons as close as possible. We also describe how to incorporate a fairness criteria into the optimization to avoid oscillations of the surface. We iterate this entire process until we reach a user specified tolerance, possibly adding clusters during iteration to guarantee convergence. We have been able to successfully reduce the number of unique triangles to lie within a small percentage of the total number of triangles in the surface and demonstrate our technique on various examples.

1. Arthur, D., and Vassilvitskii, S. 2007. k-means++: the advantages of careful seeding. In Proceedings of the 18th annual ACM-SIAM symposium on Discrete algorithms, 1027–1035. Google ScholarDigital Library
2. Arun, K. S., Huang, T. S., and Blostein, S. D. 1987. Least-squares fitting of two 3-d point sets. IEEE Trans. Pattern Anal. Mach. Intell. 9, 5, 698–700. Google ScholarDigital Library
3. Cutler, B., and Whiting, E. 2007. Constrained planar remeshing for architecture. In Proceedings GI ’07, ACM, New York, NY, USA, 11–18. Google ScholarDigital Library
4. Dos Passos, V. A., and Walter, M. 2008. 3d mosaics with variable-sized tiles. Vis. Comput. 24, 7, 617–623. Google ScholarDigital Library
5. Drew, P. 2008. New Tent Architecture. Thames & Hudson, June.Google Scholar
6. Eigensatz, M., Kilian, M., Schiftner, A., Mitra, N., Pottmann, H., and Pauly, M., 2010. Paneling architectural freeform surfaces. To appear in ACM SIGGRAPH. Google ScholarDigital Library
7. Elber, G., and Wolberg, G. 2003. Rendering traditional mosaics. The Visual Computer 19, 67–78.Google ScholarCross Ref
8. Frandsen, P. E., Jonasson, K., Nielsen, H. B., and Tingleff, O., 2004. Unconstrained optimization, 3rd edition.Google Scholar
9. Fu, C.-W., Lai, C.-F., He, Y., and Cohen-Or, D., 2010. K-set tilable surfaces. To appear in ACM SIGGRAPH. Google ScholarDigital Library
10. Grünbaum, B., and Shephard, G. C. 1986. Tilings and patterns. W. H. Freeman & Co., New York, NY, USA. Google ScholarDigital Library
11. Kim, J., and Pellacini, F. 2002. Jigsaw image mosaics. In ACM SIGGRAPH ’02, ACM, New York, NY, USA, 657–664. Google ScholarDigital Library
12. Lai, Y.-K., Hu, S.-M., and Martin, R. R. 2006. Surface mosaics. Vis. Comput. 22, 9, 604–611. Google ScholarDigital Library
13. Liu, Y., Pottmann, H., Wallner, J., Yang, Y.-L., and Wang, W. 2006. Geometric modeling with conical meshes and developable surfaces. In ACM SIGGRAPH ’06, ACM, New York, NY, USA, 681–689. Google ScholarDigital Library
14. Pavic, D., Ceumern, U., and Kobbelt, L. 2009. Gizmos: Genuine image mosaics with adaptive tiling. Comput. Graph. Forum 28, 8, 2244–2254.Google ScholarCross Ref
15. Pottmann, H., Liu, Y., Wallner, J., Bobenko, A., and Wang, W. 2007. Geometry of multi-layer freeform structures for architecture. In ACM SIGGRAPH ’07, ACM, New York, NY, USA, 65. Google ScholarDigital Library
16. Pottmann, H., Schiftner, A., Bo, P., Schmiedhofer, H., Wang, W., Baldassini, N., and Wallner, J. 2008. Freeform surfaces from single curved panels. In ACM SIGGRAPH ’08, ACM, New York, NY, USA, 1–10. Google ScholarDigital Library
17. Schiftner, A., Höbinger, M., Wallner, J., and Pottmann, H. 2009. Packing circles and spheres on surfaces. In ACM SIGGRAPH Asia ’09, ACM, New York, NY, USA, 1–8. Google ScholarDigital Library
18. Wang, L., Yu, Y., Zhou, K., and Guo, B. 2009. Example-based hair geometry synthesis. In ACM SIGGRAPH ’09, ACM, New York, NY, USA, 1–9. Google ScholarDigital Library
19. Yu, Y., Zhou, K., Xu, D., Shi, X., Bao, H., Guo, B., and Shum, H.-Y. 2004. Mesh editing with poisson-based gradient field manipulation. In ACM SIGGRAPH ’04, ACM, New York, NY, USA, 644–651. Google ScholarDigital Library