“The human touch: measuring contact with real human soft tissues” by Pai, Rothwell, Wyder-Hodge, Wick, Fan, et al. …
Conference:
Type(s):
Entry Number: 58
Title:
- The human touch: measuring contact with real human soft tissues
Session/Category Title: Virtually Human
Presenter(s)/Author(s):
- Dinesh K. Pai
- Austin Rothwell
- Pearson Wyder-Hodge
- Alistair Wick
- Ye Fan
- Darcy Harrison
- Debanga R. Neog
- Cole Shing
Moderator(s):
Abstract:
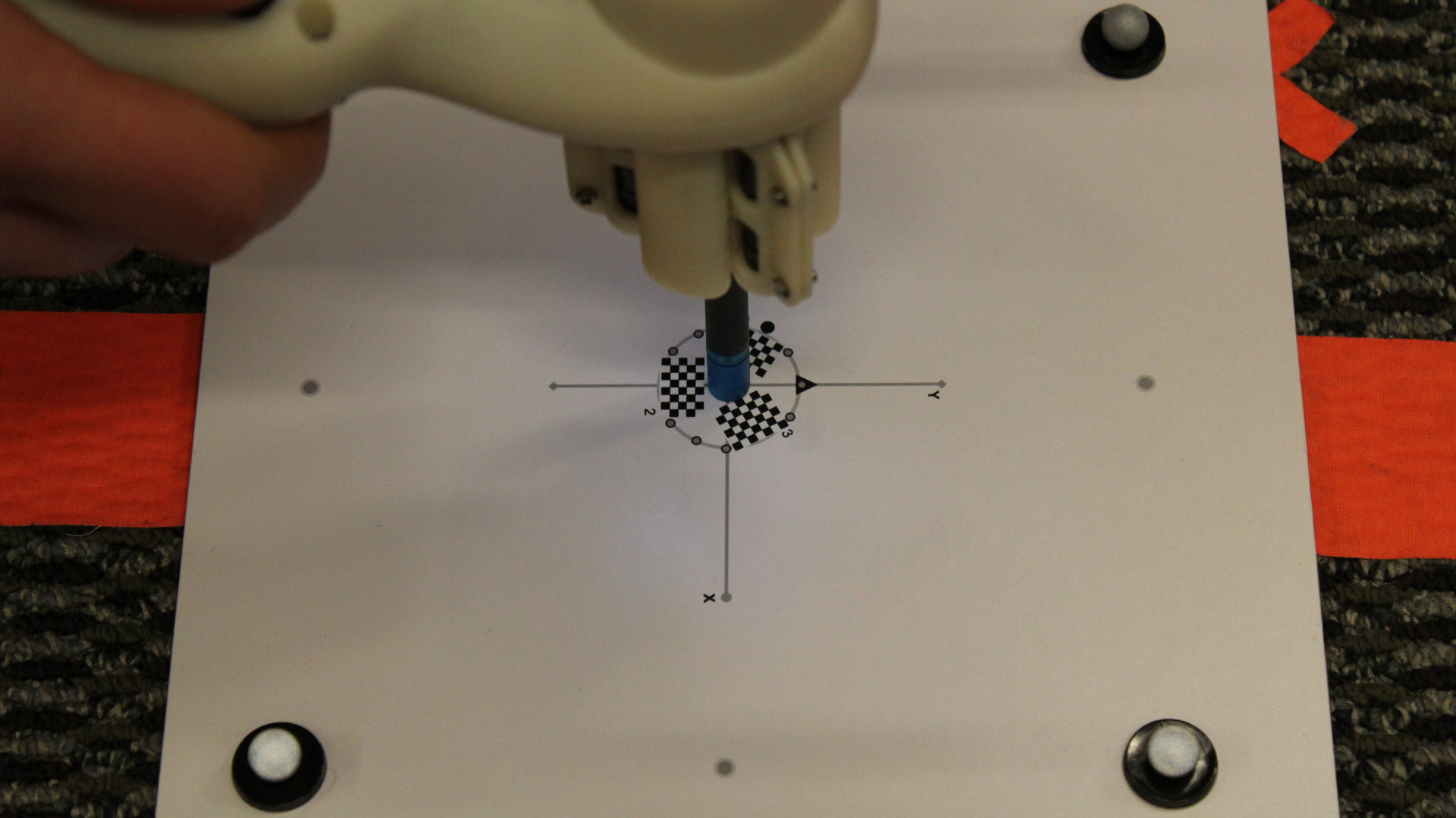
Simulating how the human body deforms in contact with external objects, tight clothing, or other humans is of central importance to many fields. Despite great advances in numerical methods, the material properties required to accurately simulate the body of a real human have been sorely lacking. Here we show that mechanical properties of the human body can be directly measured using a novel hand-held device. We describe a complete pipeline for measurement, modeling, parameter estimation, and simulation using the finite element method. We introduce a phenomenological model (the sliding thick skin model) that is effective for both simulation and parameter estimation. Our data also provide new insights into how the human body actually behaves. The methods described here can be used to create personalized models of an individual human or of a population. Consequently, our methods have many potential applications in computer animation, product design, e-commerce, and medicine.
References:
1. D. Ali-Hamadi, T. Liu, B. Gilles, L. Kavan, F. Faure, O. Palombi, and M.-P. Cani. 2013. Anatomy transfer. ACM Transactions on Graphics (TOG) 32, 6 (2013), 188. Google ScholarDigital Library
2. N. Badler. 1997. Virtual humans for animation, ergonomics, and simulation. In Nonrigid and Articulated Motion Workshop, 1997. Proceedings., IEEE. IEEE, 28–36. Google ScholarDigital Library
3. B. Bickel, M. Bächer, M. A. Otaduy, W. Matusik, H. Pfister, and M. Gross. 2009. Capture and modeling of non-linear heterogeneous soft tissue. ACM Transactions on Graphics (TOG) 28, 3 (2009), 89. Google ScholarDigital Library
4. G. Bradski. 2000. The OpenCV Library: Dense Inverse Flow. https://docs.opencv.org/3.3.1/da/d06/classcv_1_1optflow_1_1DISOpticalFlow.html. Dr. Dobb’s Journal of Software Tools (2000). Accessed: 2017-12-25.Google Scholar
5. H. Dreyfuss et al. 1967. Measure of Man. (1967).Google Scholar
6. Y. Fan, J. Litven, and D. K. Pai. 2014. Active volumetric musculoskeletal systems. ACM Transactions on Graphics (TOG) 33, 4 (2014), 152. Google ScholarDigital Library
7. C. Flynn. 2014. Fiber Matrix Models of the Dermis. In Computational Biophysics of the Skin. CRC Press, 133–160.Google Scholar
8. T. F. Gast and C. Schroeder. 2014. Optimization Integrator for Large Time Steps. In Proceedings of the ACM SIGGRAPH/Eurographics Symposium on Computer Animation (SCA ’14). Eurographics Association, Aire-la-Ville, Switzerland, Switzerland, 31–40. http://dl.acm.org/citation.cfm?id=2849517.2849523 Google ScholarDigital Library
9. P. Kadleček, A.-E. Ichim, T. Liu, J. Křivánek, and L. Kavan. 2016. Reconstructing personalized anatomical models for physics-based body animation. ACM Transactions on Graphics (TOG) 35, 6 (2016), 213. Google ScholarDigital Library
10. M. Kim, G. Pons-Moll, S. Pujades, S. Bang, J. Kim, M. Black, and S.-H. Lee. 2017. Data-Driven Physics for Human Soft Tissue Animation. ACM Transactions on Graphics, (Proc. SIGGRAPH) 36, 4 (2017). Google ScholarDigital Library
11. M. Klaudiny, S. McDonagh, D. Bradley, T. Beeler, and K. Mitchell. 2017. Real-Time Multi-View Facial Capture with Synthetic Training. Computer Graphics Forum 36, 2 (2017), 325–336. Google ScholarDigital Library
12. R. M. Koch, M. H. Gross, F. R. Carls, D. F. von Büren, G. Fankhauser, and Y. I. Parish. 1996. Simulating facial surgery using finite element models. In Proceedings of the 23rd annual conference on Computer graphics and interactive techniques. ACM, 421–428. Google ScholarDigital Library
13. T. Kroeger, R. Timofte, D. Dai, and L. Van Gool. 2016. Fast optical flow using dense inverse search. European Conference on Computer Vision (2016), 471–488.Google Scholar
14. P. G. Kry and D. K. Pai. 2006. Interaction capture and synthesis. ACM Transactions on Graphics 25, 3 (July 2006), 872–880. Google ScholarDigital Library
15. Y. Lanir and Y. Fung. 1974. Two-dimensional mechanical properties of rabbit skin-II. Experimental results. Journal of biomechanics 7, 2 (1974), 171IN9175–174182.Google Scholar
16. D. Lee, M. Glueck, A. Khan, E. Fiume, and K. Jackson. 2010. A survey of modeling and simulation of skeletal muscle. ACM Transactions on Graphics 28, 4 (2010),1–13.Google ScholarDigital Library
17. S.-H. Lee, E. Sifakis, and D. Terzopoulos. 2009. Comprehensive biomechanical modeling and simulation of the upper body. ACM Transactions on Graphics (TOG) 28, 4 (2009), 99. Google ScholarDigital Library
18. D. Li, S. Sueda, D. R. Neog, and D. K. Pai. 2013. Thin Skin Elastodynamics. ACM Trans. Graph. (Proc. SIGGRAPH) 32, 4 (July 2013), 49:1–49:9. Google ScholarDigital Library
19. G. Limbert. 2017. Mathematical and computational modelling of skin biophysics: a review. In Proc. R. Soc. A, Vol. 473. The Royal Society, 20170257.Google ScholarCross Ref
20. I. Macêdo, J. P. Gois, and L. Velho. 2009. Hermite Interpolation of Implicit Surfaces with Radial Basis Functions. In 2009 XXII Brazilian Symposium on Computer Graphics and Image Processing. 1–8. Google ScholarDigital Library
21. W. Maurel, Y. Wu, N. M. Thalmann, and D. Thalmann. 1998. Biomechanical models for soft tissue simulation. Springer. Google ScholarDigital Library
22. A. McAdams, Y. Zhu, A. Selle, M. Empey, R. Tamstorf, J. Teran, and E. Sifakis. 2011. Efficient Elasticity for Character Skinning with Contact and Collisions. In ACM SIGGRAPH 2011 Papers (SIGGRAPH ’11). ACM, New York, NY, USA, Article 37, 12 pages. Google ScholarDigital Library
23. E. Miguel, D. Miraut, and M. A. Otaduy. 2016. Modeling and Estimation of Energy-Based Hyperelastic Objects. In Computer Graphics Forum, Vol. 35. Wiley Online Library, 385–396.Google Scholar
24. N. M. Mitchell, T. W. King, A. Oliker, E. D. Sifakis, et al. 2016. A Real-Time Local Flaps Surgical Simulator Based on Advances in Computational Algorithms for Finite Element Models. Plastic and reconstructive surgery 137, 2 (2016), 445e–452e.Google Scholar
25. R. Murray, Z. Li, and S. S. Sastry. 1994. A mathematical introduction to robotic manipulation. CRC Press. Google ScholarDigital Library
26. R. Ogden. 1972. Large deformation isotropic elasticity-on the correlation of theory and experiment for incompressible rubberlike solids. In Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences, Vol. 326. The Royal Society, 565–584.Google ScholarCross Ref
27. R. Ogden, G. Saccomandi, and I. Sgura. 2004. Fitting hyperelastic models to experimental data. Computational Mechanics 34, 6 (2004), 484–502.Google ScholarCross Ref
28. D. K. Pai. 2000. Robotics in Reality-based Modeling. In Robotics Research: the Ninth International Symposium. Springer-Verlag, 353–358.Google Scholar
29. D. K. Pai, K. v. d. Doel, D. L. James, J. Lang, J. E. Lloyd, J. L. Richmond, and S. H. Yau. 2001. Scanning Physical Interaction Behavior of 3D Objects. In Proceedings of the 28th Annual Conference on Computer Graphics and Interactive Techniques (SIGGRAPH ’01). ACM, New York, NY, USA, 87–96. Google ScholarDigital Library
30. G. Pons-Moll, J. Romero, N. Mahmood, and M. J. Black. 2015. Dyna: A model of dynamic human shape in motion. ACM Transactions on Graphics (TOG) 34, 4 (2015), 120. Google ScholarDigital Library
31. R. S. Rivlin and D. Saunders. 1951. Large elastic deformations of isotropic materials. VII. Experiments on the deformation of rubber. Philosophical Transactions of the Royal Society of London A: Mathematical, Physical and Engineering Sciences 243, 865 (1951), 251–288.Google ScholarCross Ref
32. W. Si, S.-H. Lee, E. Sifakis, and D. Terzopoulos. 2014. Realistic biomechanical simulation and control of human swimming. ACM Transactions on Graphics (TOG) 34, 1 (2014), 10. Google ScholarDigital Library
33. E. Sifakis and J. Barbic. 2012. FEM Simulation of 3D Deformable Solids: A Practitioner’s Guide to Theory, Discretization and Model Reduction. In ACM SIGGRAPH 2012 Courses (SIGGRAPH ’12). ACM, New York, NY, USA, Article 20, 50 pages. Google ScholarDigital Library
34. E. Sifakis and J. Barbič. 2015. Finite Element Method Simulation of 3D Deformable Solids. Synthesis Lectures on Visual Computing: Computer Graphics, Animation, Computational Photography, and Imaging 1, 1 (2015), 1–69. Google ScholarDigital Library
35. E. Sifakis, I. Neverov, and R. Fedkiw. 2005. Automatic determination of facial muscle activations from sparse motion capture marker data. In Acm transactions on graphics (tog), Vol. 24. ACM, 417–425. Google ScholarDigital Library
36. B. Smith, F. de Goes, and T. Kim. 2017. Stable Neo-Hookean Flesh Simulation. ACM Trans. Graph. (Dec. 2017). Google ScholarDigital Library
37. S. Sueda, A. Kaufman, and D. K. Pai. 2008. Musculotendon Simulation for Hand Animation. ACM Trans. Graph. (Proc. SIGGRAPH) 27, 3 (2008), 83:1–83:8. Google ScholarDigital Library
38. J. Teran, E. Sifakis, S. S. Blemker, V. Ng-Thow-Hing, C. Lau, and R. Fedkiw. 2005a. Creating and simulating skeletal muscle from the visible human data set. IEEE Transactions on Visualization and Computer Graphics 11, 3 (2005), 317–328. Google ScholarDigital Library
39. J. Teran, E. Sifakis, G. Irving, and R. Fedkiw. 2005b. Robust Quasistatic Finite Elements and Flesh Simulation. In Proceedings of the 2005 ACM SIGGRAPH/Eurographics Symposium on Computer Animation (SCA ’05). ACM, New York, NY, USA, 181–190. Google ScholarDigital Library
40. R. Vaillant, L. Barthe, G. Guennebaud, M.-P. Cani, D. Rohmer, B. Wyvill, O. Gourmel, and M. Paulin. 2013. Implicit skinning: real-time skin deformation with contact modeling. ACM Transactions on Graphics (TOG) 32, 4 (2013), 125. Google ScholarDigital Library
41. B. Wang, L. Wu, K. Yin, U. M. Ascher, L. Liu, and H. Huang. 2015. Deformation capture and modeling of soft objects. ACM Trans. Graph. 34, 4 (2015), 94–1. Google ScholarDigital Library
42. H. Wendland. 2005. Scattered Data Approximation: Cambridge Monographs on Applied and Computational Mathematics No. 17. Cambridge University Press, GB, Chapter 16.2 – Hermite-Birkhoff interpolation.Google Scholar
43. C. Wu, D. Bradley, M. Gross, and T. Beeler. 2016. An Anatomically-constrained Local Deformation Model for Monocular Face Capture. ACM Trans. Graph. 35, 4, Article 115 (July 2016), 12 pages. Google ScholarDigital Library