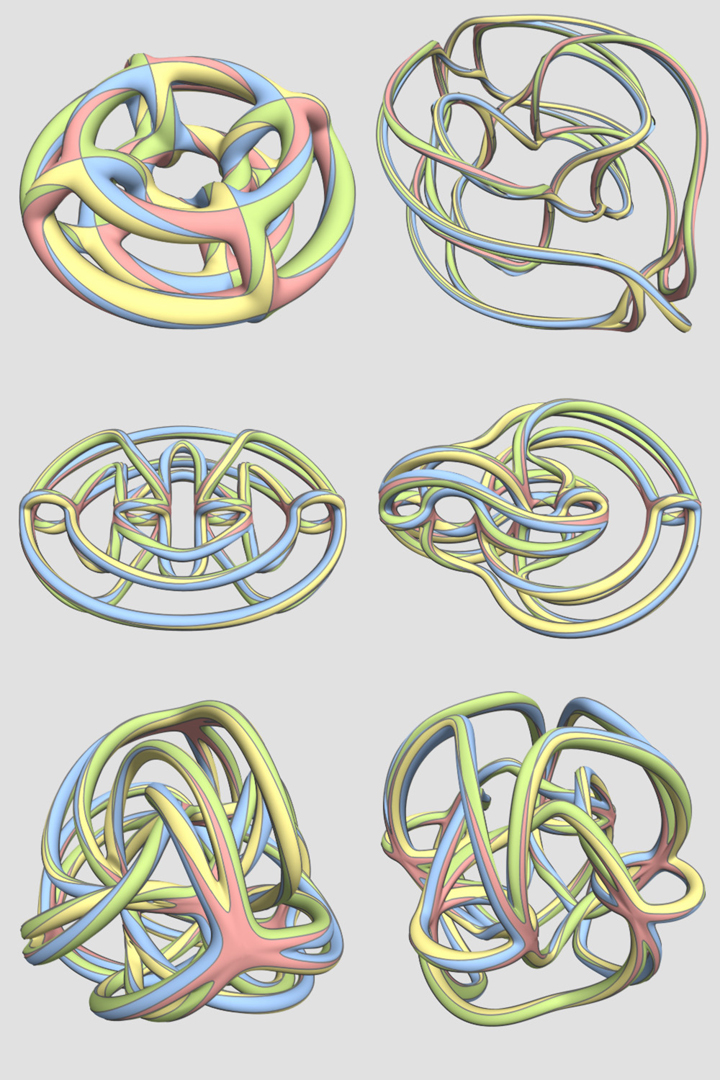
“Symmetric tiling of closed surfaces: visualization of regular maps” by van Wijk

A regular map is a tiling of a closed surface into faces, bounded by edges that join pairs of vertices, such that these elements exhibit a maximal symmetry. For genus 0 and 1 (spheres and tori) it is well known how to generate and present regular maps, the Platonic solids are a familiar example. We present a method for the generation of space models of regular maps for genus 2 and higher. The method is based on a generalization of the method for tori. Shapes with the proper genus are derived from regular maps by tubification: edges are replaced by tubes. Tessellations are produced using group theory and hyperbolic geometry. The main results are a generic procedure to produce such tilings, and a collection of intriguing shapes and images. Furthermore, we show how to produce shapes of genus 2 and higher with a highly regular structure.

1. Akleman, E., and Chen, J. 2006. Regular meshes construction algorithms using regular handles. In Proceedings of Shape Modeling International 2006, Matsushima, Japan. Google ScholarDigital Library
2. Anderson, J. W. 2007. Hyperbolic Geometry, 2nd edition. Springer.Google Scholar
3. Beardon, A. F. 1983. The Geometry of Discrete Groups. Springer.Google Scholar
4. Catmull, E., and Clark, J. 1978. Recursively generated B-spline surfaces on arbitrary topological surfaces. Computer-Aided Design 10, 6, 350–355.Google ScholarCross Ref
5. Conder, M., and Dobcsányi, P. 2001. Determination of all regular maps of small genus. Journal of Combinatorial Theory, Series B 81, 224–242. Google ScholarDigital Library
6. Conder, M., 2006. Orientable regular maps of genus 2 to 101. http://www.math.auckland.ac.nz/~conder.Google Scholar
7. Conway, J. H., Burgiel, H., and Goodman-Strauss, C. 2008. The Symmetries of Things. A. K. Peters.Google Scholar
8. Coxeter, H., and Moser, W. 1984. Generators and Relations for Discrete Groups. Springer-Verlag, Berlin. Reprint of the fourth edition.Google Scholar
9. Coxeter, H. 1989. Introduction to Geometry, Second Edition. Wiley.Google Scholar
10. Eades, P. 1984. A heuristic for graph drawing. Congressus Numerantium 42, 149–160.Google Scholar
11. Firby, P. A., and Gardiner, C. F. 2001. Surface topology, 3rd edition. Horwood publishing.Google Scholar
12. Francis, G. K. 1987. A Topological Picturebook. Springer.Google Scholar
13. Hanson, A. 1994. A construction for computer visualization of certain complex curves. Notices of the Amer. Math. Soc. 41, 9 (November/December), 1156–1163.Google Scholar
14. Havas, G., and Ramsay, C., 2002. ACE — Advanced Coset Enumerator. http://www.itee.uq.edu.au/~cram/ce.html.Google Scholar
15. Hong, S.-H. 2002. Drawing graphs symmetrically in three dimensions. In Graph Drawing, 9th International Symposium, GD 2001, Vienna, Springer, vol. 2265 of Lecture Notes in Computer Science, 189–204. Google ScholarDigital Library
16. Klein, F. 1879. Über die Transformation siebenter Ordnung der elliptischen Functionen. Math. Ann. 14, 428–471.Google ScholarCross Ref
17. Levy, S. 1999. The Eightfold Way: The Beauty of Klein’s Quartic Curve. Cambridge University Press.Google Scholar
18. Lozada, L. A. P., de Mendonĉa Neto, C. F. X., Rosi, R. M., and Stolfi, J. 1997. Automatic visualization of twodimensional cellular complexes. In Graph Drawing, Symposium on Graph Drawing, GD ’96, Berkeley, Springer, vol. 1190 of Lecture Notes in Computer Science, 303–317. Google ScholarDigital Library
19. Nedela, R. 2001. Regular maps — combinatorial objects relating different fields of mathematics. Journal of the Korean Mathematical Society 38, 5, 1069–1105.Google Scholar
20. Séquin, C. H. 2006. Patterns on the genus-3 Klein quartic. In Proc. BRIDGES 2006 Conference, London, 245–254.Google Scholar
21. Séquin, C. H. 2007. Symmetric embedding of locally regular hyperbolic tilings. In Proc. BRIDGES 2007 Conference, San Sebastian, 379–388.Google Scholar
22. Stewart, I. 2008. Why Beauty is Truth: The history of Symmetry. Basic Books.Google Scholar
23. van Wijk, J. J., and Cohen, A. M. 2006. Visualization of Seifert surfaces. IEEE Trans. on Visualization and Computer Graphics 12, 4, 485–496. Google ScholarDigital Library