“Somigliana Coordinates: an elasticity-derived approach for cage deformation” by Chen, Goes and Desbrun
Conference:
Type(s):
Title:
- Somigliana Coordinates: an elasticity-derived approach for cage deformation
Session/Category Title: All About Meshes
Presenter(s)/Author(s):
Moderator(s):
Abstract:
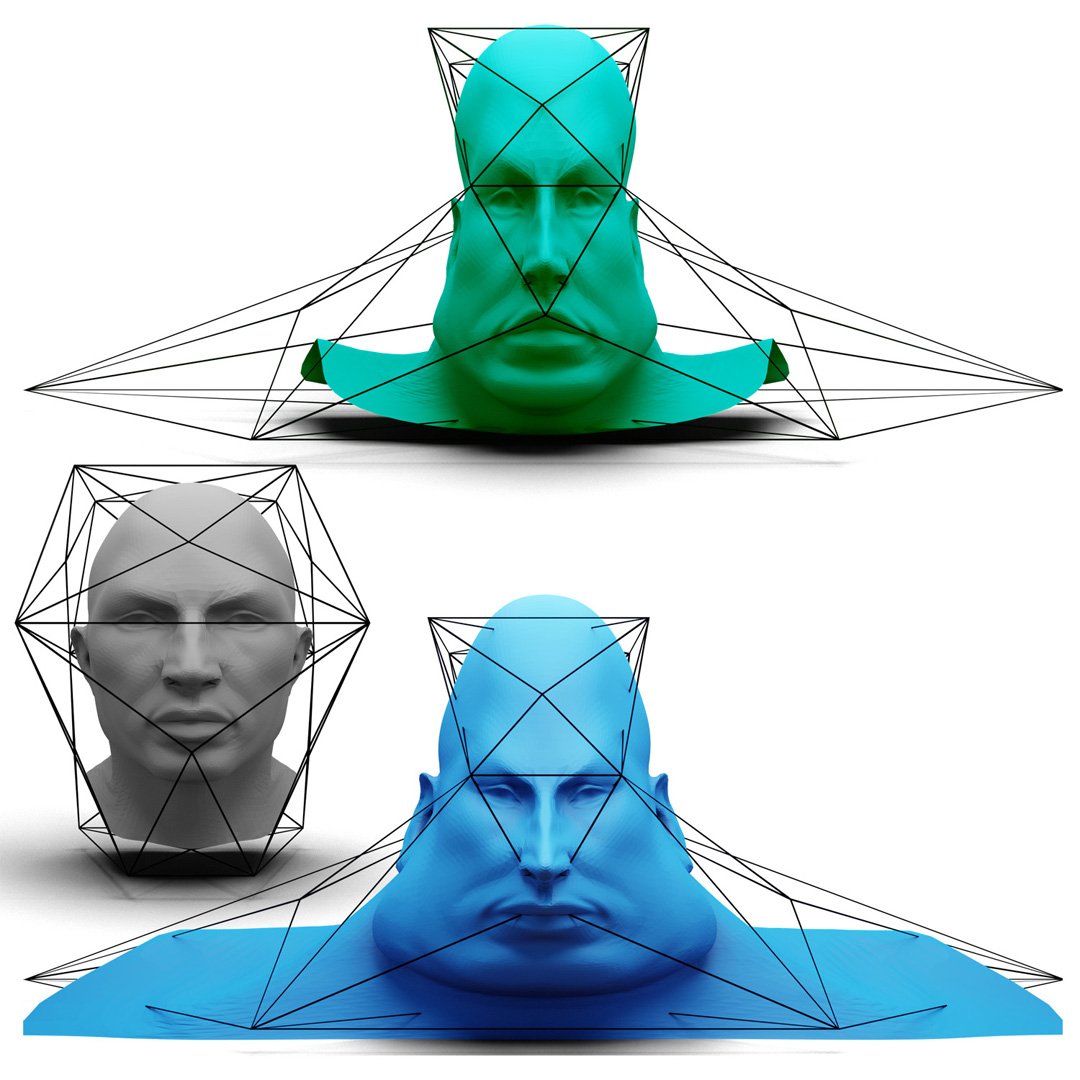
In this paper, we present a novel cage deformer based on elasticity-derived matrix-valued coordinates. In order to bypass the typical shearing artifacts and lack of volume control of existing cage deformers, we promote a more elastic behavior of the cage deformation by deriving our coordinates from the Somigliana identity, a boundary integral formulation based on the fundamental solution of linear elasticity. Given an initial cage and its deformed pose, the deformation of the cage interior is deduced from these Somigliana coordinates via a corotational scheme, resulting in a matrix-weighted combination of both vertex positions and face normals of the cage. Our deformer thus generalizes Green coordinates, while producing physically-plausible spatial deformations that are invariant under similarity transformations and with interactive bulging control. We demonstrate the efficiency and versatility of our method through a series of examples in 2D and 3D.
References:
1. Adobe. 2022. Substance 3D Modeler. http://www.adobe.com/products/substance3d-modeler.html
2. Jernej Barbič and Doug L. James. 2005. Real-Time Subspace Integration for St. Venant-Kirchhoff Deformable Models. ACM Trans. Graph. 24, 3 (2005), 982–990.
3. Jernej Barbič and Yili Zhao. 2011. Real-time Large-deformation Substructuring. ACM Trans. Graph. 30, 4, Article 91 (2011).
4. Mirela Ben-Chen, Ofir Weber, and Craig Gotsman. 2009. Variational Harmonic Maps for Space Deformation. ACM Trans. Graph. 28, 3, Article 34 (2009).
5. Blender. 2022. A 3D modelling and rendering package. Blender Online Community. http://www.blender.org
6. Max Budninskiy, Beibei Liu, Yiying Tong, and Mathieu Desbrun. 2016. Power Coordinates: A Geometric Construction of Barycentric Coordinates on Convex Polytopes. ACM Trans. Graph. 35, 6, Article 241 (2016).
7. Jiong Chen and Mathieu Desbrun. 2022. Go Green: General Regularized Green’s Functions for Elasticity. In ACM SIGGRAPH Proceedings. Article 6.
8. Thommas A. Cruse and Wan Suwito. 1993. On the Somigliana Stress Identity in Elasticity. Computational Mechanics 11 (1993), 1–10.
9. Fernando de Goes and Doug L. James. 2017. Regularized Kelvinlets: Sculpting Brushes Based on Fundamental Solutions of Elasticity. ACM Trans. Graph. 36, 4, Article 40 (2017).
10. Fernando de Goes and Doug L. James. 2018. Dynamic Kelvinlets: Secondary Motions Based on Fundamental Solutions of Elastodynamics. ACM Trans. Graph. 37, 4, Article 81 (2018).
11. Fernando de Goes and Doug L. James. 2019. Sharp Kelvinlets: Elastic Deformations with Cusps and Localized Falloffs. In Proceedings of the Digital Production Symposium. Article 2.
12. Michael S. Floater. 2003. Mean Value Coordinates. Comp. Aided Geom. Design 20, 1 (2003), 19–27.
13. Michael S. Floater. 2015. Generalized barycentric coordinates and applications. Acta Numerica 24 (2015), 161–214.
14. Kai Hormann and N. Sukumar. 2008. Maximum Entropy Coordinates for Arbitrary Polytopes. In Symp. Geo. Proc.1513–1520.
15. Kai Hormann and N. Sukumar. 2017. Generalized barycentric coordinates in computer graphics and computational mechanics. CRC Press.
16. Doug L. James. 2020. Phong deformation: a better C0 interpolant for embedded deformation. ACM Trans. Graph. 39, 4 (2020), 56–1.
17. Doug L. James and Dinesh K. Pai. 1999. ArtDefo: Accurate Real Time Deformable Objects. In Proceedings of the 26th Annual Conference on Computer Graphics and Interactive Techniques. 65–72.
18. Doug L. James and Dinesh K. Pai. 2003. Multiresolution Green’s Function Methods for Interactive Simulation of Large-Scale Elastostatic Objects. ACM Trans. Graph. 22, 1 (2003), 47–82.
19. Pushkar Joshi, Mark Meyer, Tony DeRose, Brian Green, and Tom Sanocki. 2007. Harmonic Coordinates for Character Articulation. ACM Trans. Graph. 26, 3, Article 71 (2007).
20. Tao Ju, Scott Schaefer, and Joe Warren. 2005. Mean Value Coordinates for Closed Triangular Meshes. ACM Trans. Graph. 24, 3 (2005), 561–566.
21. Lord Kelvin. 1848. Note on the Integration of the Equations of Equilibrium of an Elastic Solid. Cambridge and Dublin Mathematical Journal 3 (1848), 87–89.
22. Binh Huy Le and Zhigang Deng. 2017. Interactive Cage Generation for Mesh Deformation. In Symposium on Interactive 3D Graphics and Games. Article 3.
23. Yaron Lipman, Johannes Kopf, Daniel Cohen-Or, and David Levin. 2007. GPU-assisted Positive Mean Value Coordinates for Mesh Deformations. In Symposium on Geometry Processing.
24. Yaron Lipman, David Levin, and Daniel Cohen-Or. 2008. Green Coordinates. ACM Trans. Graph. 27, 3 (2008), 1–10.
25. Sebastian Martin, Christopher O. Huber, Peter Kaufmann, and Markus H. Gross. 2009. Shape-Preserving Animation of Deformable Objects. In International Workshop on Vision, Modeling, and Visualization. 65–72.
26. Aleka McAdams, Yongning Zhu, Andrew Selle, Mark Empey, Rasmus Tamstorf, Joseph Teran, and Eftychios Sifakis. 2011. Efficient Elasticity for Character Skinning with Contact and Collisions. ACM Trans. Graph. 30, 4, Article 37 (2011).
27. Mark Meyer, Alan Barr, Haeyoung Lee, and Mathieu Desbrun. 2002. Generalized Barycentric Coordinates on Irregular Polygons. J. Graph. Tools 7, 1 (2002), 13–22.
28. Constantine Pozrikidis. 2002. Practical Guide to Boundary Element Methods with the Software Library Bemlib.
29. Eftychios Sifakis and Jernej Barbic. 2012. FEM Simulation of 3D Deformable Solids. In ACM SIGGRAPH courses.
30. William S Slaughter. 2012. The Linearized Theory of Elasticity. Springer.
31. Breannan Smith, Fernando de Goes, and Theodore Kim. 2018. Stable Neo-Hookean Flesh Simulation. ACM Trans. Graph. 37, 2, Article 12 (2018), 15 pages.
32. Carlo Somigliana. 1885. Sopra l’Equilibrio di un Corpo Elastico Isotropo. Nuovo Cimento 3 (1885), 140–148.
33. Jean-Marc Thiery and Tamy Boubekeur. 2022. Green Coordinates for Triquad Cages in 3D. In SIGGRAPH Asia 2022 Conference Papers. Article 38.
34. Jean-Marc Thiery, Pooran Memari, and Tamy Boubekeur. 2018. Mean Value Coordinates for Quad Cages in 3D. ACM Trans. Graph. 37, 6, Article 229 (2018).
35. Shinyi Umeyama. 1991. Least-squares Estimation of Transformation Parameters Between Two Point Patterns. IEEE Trans. PAMI 13, 4 (1991), 376–380.
36. Joe Warren, Scott Schaefer, Anil Hirani, and Mathieu Desbrun. 2007. Barycentric Coordinates for Convex Sets. Adv. Comput. Math. 27, 3 (2007), 319–338.
37. Ofir Weber. 2017. Planar Shape Deformation. In Generalized Barycentric Coordinates in Computer Graphics and Computational Mechanics, Kai Hormann and N. Sukumar (Eds.). CRC Press, Chapter 7, 109–133.
38. Ofir Weber, Mirela Ben-Chen, and Craig Gotsman. 2009. Complex Barycentric Coordinates with Applications to Planar Shape Deformation. Comp. Graph. Forum 28, 2 (2009).
39. Chuhua Xian, Hongwei Lin, and Shuming Gao. 2012. Automatic Cage Generation by Improved OBBs for Mesh Deformation. Vis. Comp. 28, 1 (2012), 21–33.