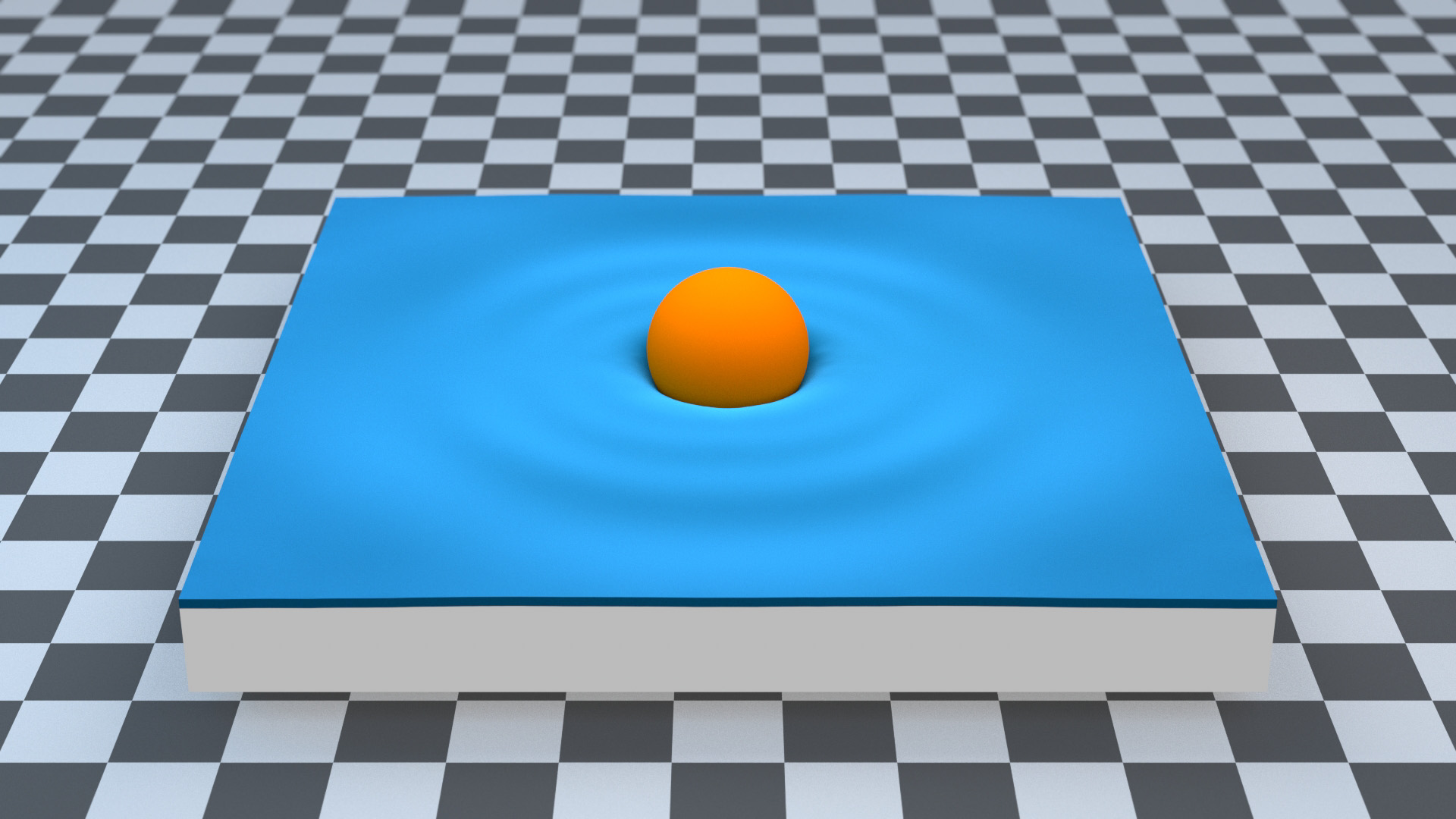
“Solid-fluid interaction with surface-tension-dominant contact” by Ruan, Liu, Zhu, Sueda, Wang, et al. …

We propose a novel three-way coupling method to model the contact interaction between solid and fluid driven by strong surface tension. At the heart of our physical model is a thin liquid membrane that simultaneously couples to both the liquid volume and the rigid objects, facilitating accurate momentum transfer, collision processing, and surface tension calculation. This model is implemented numerically under a hybrid Eulerian-Lagrangian framework where the membrane is modelled as a simplicial mesh and the liquid volume is simulated on a background Cartesian grid. We devise a monolithic solver to solve the interactions among the three systems of liquid, solid, and membrane. We demonstrate the efficacy of our method through an array of rigid-fluid contact simulations dominated by strong surface tension, which enables the faithful modeling of a host of new surface-tension-dominant phenomena including: objects with higher density than water that remains afloat; ‘Cheerios effect’ where floating objects attract one another; and surface tension weakening effect caused by surface-active constituents.

1. Mridul Aanjaneya. 2018. An efficient solver for two-way coupling rigid bodies with incompressible flow. Computer Graphics Forum 37, 8 (2018), 59–68.Google ScholarCross Ref
2. Christopher Batty, Florence Bertails, and Robert Bridson. 2007. A fast variational framework for accurate solid-fluid coupling. ACM Trans. on Graphics 26, 3 (2007), 100–es.Google ScholarDigital Library
3. Christopher Batty, Andres Uribe, Basile Audoly, and Eitan Grinspun. 2012. Discrete viscous sheets. ACM Trans. on Graphics 31, 4 (2012), 1–7.Google ScholarDigital Library
4. Mikhail Belkin and Partha Niyogi. 2008. Towards a theoretical foundation for Laplacian-based manifold methods. J. of Computer and System Sciences 74, 8 (2008), 1289–1308.Google ScholarDigital Library
5. Mikhail Belkin, Jian Sun, and Yusu Wang. 2009. Constructing Laplace Operator from Point Clouds in Rd. In Proceedings of the 20’th Annual ACM-SIAM Symposium on Discrete Algorithms. 1031–1040.Google Scholar
6. Tyson Brochu and Robert Bridson. 2009. Robust Topological Operations for Dynamic Explicit Surfaces. SIAM Journal on Scientific Computing 31, 4 (2009), 2472–2493. Google ScholarDigital Library
7. J. Bény, C. Kotsalos, and J. Latt. 2019. Toward Full GPU Implementation of Fluid-Structure Interaction. In 2019 18th International Symposium on Parallel and Distributed Computing (ISPDC). 16–22.Google Scholar
8. Mark Carlson, Peter J. Mucha, and Greg Turk. 2004. Rigid Fluid: Animating the Interplay between Rigid Bodies and Fluid. In ACM SIGGRAPH 2004 Papers. Association for Computing Machinery, 377–384.Google ScholarDigital Library
9. Yi-Lu Chen, Jonathan Meier, Barbara Solenthaler, and Vinicius C. Azevedo. 2020. An Extended Cut-Cell Method for Sub-Grid Liquids Tracking with Surface Tension. ACM Trans. Graph. 39, 6, Article 169 (Nov. 2020), 13 pages.Google ScholarDigital Library
10. Nuttapong Chentanez, Tolga G. Goktekin, Bryan E. Feldman, and James F. O’Brien. 2006. Simultaneous Coupling of Fluids and Deformable Bodies. In ACM SIGGRAPH 2006 Sketches (SIGGRAPH ’06). 65–es.Google Scholar
11. Ka Chun Cheung, Leevan Ling, and Steven J Ruuth. 2015. A localized meshless method for diffusion on folded surfaces. J. of Computational Physics 297 (2015), 194–206.Google ScholarDigital Library
12. Fang Da, Christopher Batty, Chris Wojtan, and Eitan Grinspun. 2015. Double bubbles sans toil and trouble: Discrete circulation-preserving vortex sheets for soap films and foams. ACM Trans. on Graphics 34, 4 (2015), 1–9.Google ScholarDigital Library
13. Fang Da, David Hahn, Christopher Batty, Chris Wojtan, and Eitan Grinspun. 2016. Surface-only liquids. ACM Trans. on Graphics 35, 4 (2016), 1–12.Google ScholarDigital Library
14. Leonardo Dagum and Ramesh Menon. 1998. OpenMP: An Industry-Standard API for Shared-Memory Programming. IEEE Comput. Sci. Eng. 5, 1 (1998), 46–55.Google ScholarDigital Library
15. Richard Fitzpatrick. 2017. Surface tension. In Theoretical Fluid Mechanics. IOP Publishing, 1–21.Google Scholar
16. Olivier G’enevaux, Arash Habibi, and Jean-Michel Dischler. 2003. Simulating Fluid-Solid Interaction. In Proceedings of the Graphics Interface 2003 Conference, June 11-13, 2003, Halifax, Nova Scotia, Canada. 31–38.Google Scholar
17. Robert A Gingold and Joseph J Monaghan. 1977. Smoothed particle hydrodynamics: theory and application to non-spherical stars. Monthly Notices of the Royal Astronomical Society 181, 3 (1977), 375–389.Google ScholarCross Ref
18. Eran Guendelman, Andrew Selle, Frank Losasso, and Ronald Fedkiw. 2005. Coupling water and smoke to thin deformable and rigid shells. ACM Trans. on Graphics 24, 3 (2005), 973–981.Google ScholarDigital Library
19. David L. Hu, Manu Prakash, Brian Chan, and John W. M. Bush. 2010. Water-walking devices. Springer Berlin Heidelberg, 131–140.Google Scholar
20. David AB Hyde and Ronald Fedkiw. 2019. A unified approach to monolithic solid-fluid coupling of sub-grid and more resolved solids. J. of Computational Physics 390 (2019), 490–526.Google ScholarDigital Library
21. Sadashige Ishida, Masafumi Yamamoto, Ryoichi Ando, and Toshiya Hachisuka. 2017. A hyperbolic geometric flow for evolving films and foams. ACM Trans. on Graphics 36, 6 (2017), 1–11.Google ScholarDigital Library
22. Stoffel D. Janssens, Vikash Chaurasia, and Eliot Fried. 2017. Effect of a surface tension imbalance on a partly submerged cylinder. Journal of Fluid Mechanics 830 (2017), 369–386.Google ScholarCross Ref
23. Myungjoo Kang, Ronald Fedkiw, and Xu dong Liu. 2000. A Boundary Condition Capturing Method for Multiphase Incompressible Flow. J. of Scientific Computing 15 (2000), 323–360.Google ScholarDigital Library
24. Rongjie Lai, J. Liang, and H. Zhao. 2013. A local mesh method for solving PDEs on point clouds. Inverse Problems and Imaging 7 (2013), 737–755.Google ScholarCross Ref
25. P. Lancaster and K. Salkauskas. 1981. Surfaces generated by moving least squares methods. Math. Comp. 37 (1981), 141–158.Google ScholarCross Ref
26. Richard M Murray, Zexiang Li, S Shankar Sastry, and S Shankara Sastry. 1994. A mathematical introduction to robotic manipulation. CRC press.Google Scholar
27. G Navascues. 1979. Liquid surfaces: theory of surface tension. Reports on Progress in Physics 42, 7 (jul 1979), 1131–1186.Google ScholarCross Ref
28. Frank H.P. NVIDIA and Vingelmann, Péter and Fitzek. 2020. CUDA, release: 10.2.89. https://developer.nvidia.com/cuda-toolkitGoogle Scholar
29. O. Ozcan, H. Wang, J. Taylor, and M. Sitti. 2014. STRIDE II: A Water Strider-inspired Miniature Robot with Circular Footpads. International Journal of Advanced Robotic Systems 11 (2014).Google Scholar
30. Stéphane Popinet. 2018. Numerical Models of Surface Tension. Annual Review of Fluid Mechanics 50, 1 (2018), 49–75.Google ScholarCross Ref
31. Avi Robinson-Mosher, R Elliot English, and Ronald Fedkiw. 2009. Accurate tangential velocities for solid fluid coupling. In Proc. ACM SIGGRAPH/Eurographics Symp. on Computer Animation. 227–236.Google ScholarDigital Library
32. Avi Robinson-Mosher, Craig Schroeder, and Ronald Fedkiw. 2011. A symmetric positive definite formulation for monolithic fluid structure interaction. J. of Computational Physics 230, 4 (2011), 1547–1566.Google ScholarDigital Library
33. Avi Robinson-Mosher, Tamar Shinar, Jon Gretarsson, Jonathan Su, and Ronald Fedkiw. 2008. Two-way coupling of fluids to rigid and deformable solids and shells. ACM Trans. on Graphics 27, 3 (2008), 1–9.Google ScholarDigital Library
34. Craig Schroeder, Wen Zheng, and Ronald Fedkiw. 2012. Semi-implicit surface tension formulation with a Lagrangian surface mesh on an Eulerian simulation grid. J. Comput. Phys. 231, 4 (2012), 2092–2115.Google ScholarDigital Library
35. Side Effects Software. 2021. Houdini. https://www.sidefx.com.Google Scholar
36. Y. S. Song and M. Sitti. 2007. Surface-Tension-Driven Biologically Inspired Water Strider Robots: Theory and Experiments. IEEE Trans. on Robotics 23 (2007), 578–589.Google ScholarDigital Library
37. Jos Stam. 1999. Stable fluids. In Proc. of the 26th Annual Conference on Computer Graphics and Interactive Techniques. 121–128.Google ScholarDigital Library
38. Steve H. Suhr, Y. S. Song, S. Lee, and M. Sitti. 2005. Biologically Inspired Miniature Water Strider Robot. In Robotics: Science and Systems.Google Scholar
39. Mark Sussman and Mitsuhiro Ohta. 2009. A stable and efficient method for treating surface tension in incompressible two-phase flow. SIAM J. on Scientific Computing 31, 4 (2009), 2447–2471.Google ScholarDigital Library
40. Hui Wang, Yongxu Jin, Anqi Luo, Xubo Yang, and Bo Zhu. 2020. Codimensional surface tension flow using moving-least-squares particles. ACM Trans. on Graphics 39, 4 (2020), 42–1.Google ScholarDigital Library
41. Huamin Wang, Peter J Mucha, and Greg Turk. 2005. Water drops on surfaces. ACM Trans. on Graphics 24, 3 (2005), 921–929.Google ScholarDigital Library
42. Ying Wang, Nicholas J. Weidner, Margaret A. Baxter, Yura Hwang, Danny M. Kaufman, and Shinjiro Sueda. 2019. REDMAX: Efficient & Flexible Approach for Articulated Dynamics. ACM Trans. on Graphics 38, 4 (2019), 1–10.Google ScholarDigital Library
43. Omar Zarifi and Christopher Batty. 2017. A positive-definite cut-cell method for strong two-way coupling between fluids and deformable bodies. In Proc. ACM SIGGRAPH/Eurographics Symp. on Computer Animation. 1–11.Google ScholarDigital Library
44. Yizhong Zhang, Huamin Wang, Shuai Wang, Yiying Tong, and Kun Zhou. 2011. A deformable surface model for real-time water drop animation. IEEE Trans. Visualization & Computer Graphics 18, 8 (2011), 1281–1289.Google ScholarDigital Library
45. Wen Zheng, Jun-Hai Yong, and Jean-Claude Paul. 2009. Simulation of bubbles. Graphical Models 71, 6 (2009), 229–239.Google ScholarDigital Library
46. Wen Zheng, Bo Zhu, Byungmoon Kim, and Ronald Fedkiw. 2015. A new incompressibility discretization for a hybrid particle MAC grid representation with surface tension. J. of Computational Physics 280 (2015), 96–142.Google ScholarDigital Library
47. Bo Zhu, Minjae Lee, Ed Quigley, and Ronald Fedkiw. 2015. Codimensional non-Newtonian fluids. ACM Trans. on Graphics 34, 4 (2015), 1–9.Google ScholarDigital Library
48. B. Zhu, Ed Quigley, Matthew Cong, J. Solomon, and Ronald Fedkiw. 2014. Codimensional surface tension flow on simplicial complexes. ACM Trans. on Graphics 33 (2014), 1 — 11.Google ScholarDigital Library