“Robust fitting of super-helices to parametric curves” by Bergbom, Museth and Roble

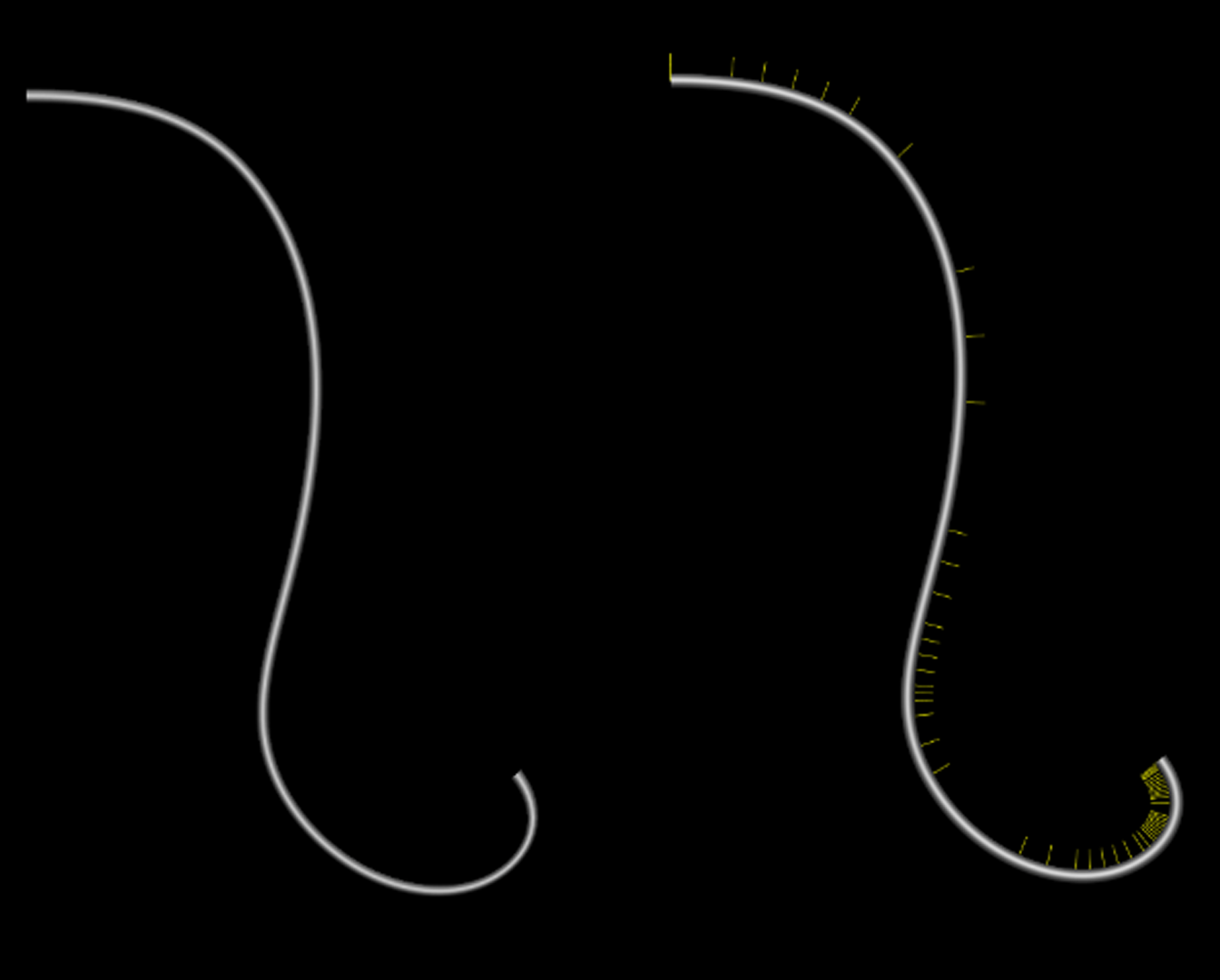
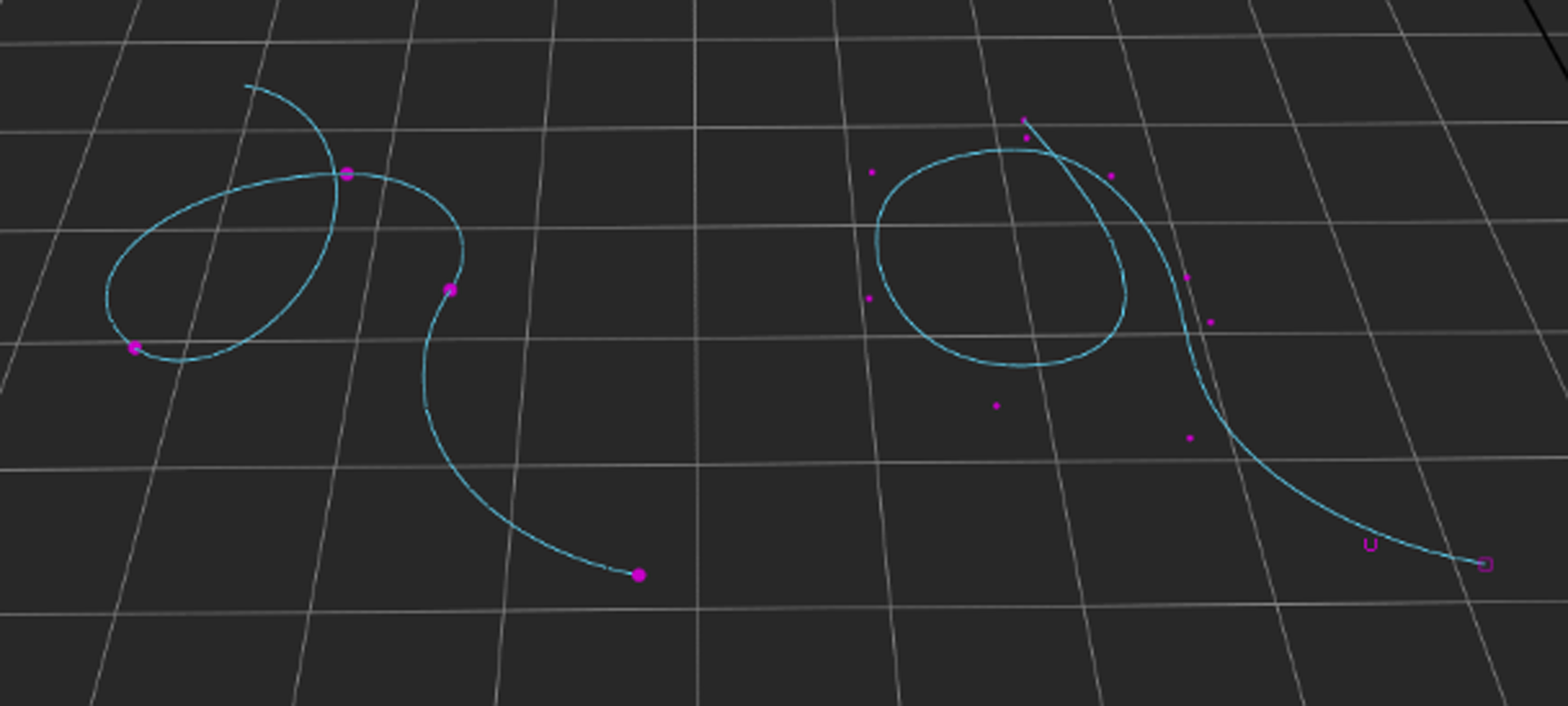
We present a robust technique for Super-Helix (SH) modeling based on a variational and iterative fitting of SH curves to arbitrary regular parametric curves. Recently Bertails et al. [2006] proposed using Cosserat curves with piecewise constant curvature – so called Super-Helices – for dynamic simulations of hair. This approach mainly has two advantages over previous work; it can easily handle curly hair, as well as preserve its length during deformations (i.e. prevent stretching). These are simple consequences of the underlying mathematical SH model. However, this all comes at the price of having to represent curves (or hair strands) in an unfamiliar and largely unintuitive parameter-space of curvatures and twists. Consequently the modeling and styling of SH curves is a challenging task that was not addressed in [Bertails et al. 2006]. In our method we employ data reduction and error-analysis known from mesh decimation algorithms as well as non-linear minimization, in order to fit SH curves to arbitrary parametric curves. This approach allows us to take advantag of the large body of existing work on parametric curve modeling. In particular, several commercially available packages already exist for grooming hair represented by NURBS curves. Our fitting procedure enables us to convert NURBS curves to SH curves, which in turn serve as input for subsequent dynamic simulation of the hair.

1. Bertails, F., Audoly, B., Cani, M.-P., Querleux, B., Leroy, F., and Lévêque, J.-L. 2006. Super-helices for predicting the dynamics of natural hair. In ACM Transactions on Graphics (Proceedings of the SIGGRAPH conference). accepted to Siggraph’06.