“Reciprocal frame structures made easy” by Fu, Song, Prashant, Zheng, Mitra, et al. …
Conference:
Type(s):
Title:
- Reciprocal frame structures made easy
Session/Category Title: Building Structures & Layouts
Presenter(s)/Author(s):
Moderator(s):
Abstract:
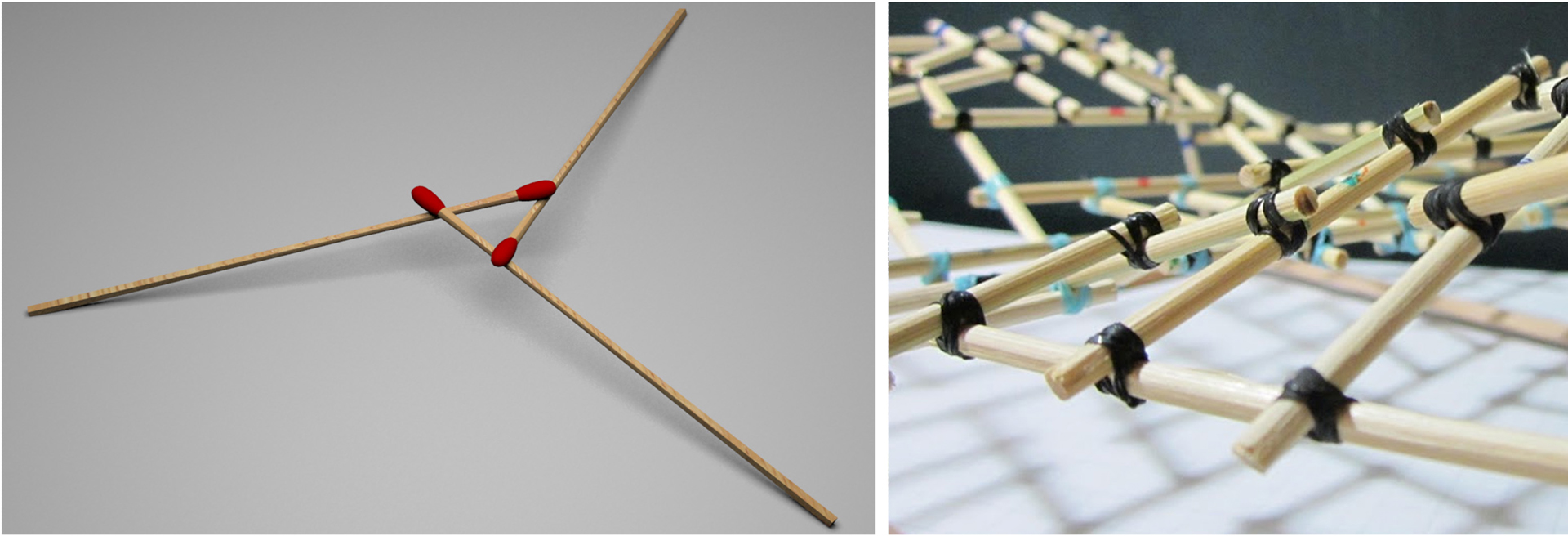
A reciprocal frame (RF) is a self-supported three-dimensional structure made up of three or more sloping rods, which form a closed circuit, namely an RF-unit. Large RF-structures built as complex grillages of one or a few similar RF-units have an intrinsic beauty derived from their inherent self-similar and highly symmetric patterns. Designing RF-structures that span over large domains is an intricate and complex task. In this paper, we present an interactive computational tool for designing RF-structures over a 3D guiding surface, focusing on the aesthetic aspect of the design.There are three key contributions in this work. First, we draw an analogy between RF-structures and plane tiling with regular polygons, and develop a computational scheme to generate coherent RF-tessellations from simple grammar rules. Second, we employ a conformal mapping to lift the 2D tessellation over a 3D guiding surface, allowing a real-time preview and efficient exploration of wide ranges of RF design parameters. Third, we devise an optimization method to guarantee the collinearity of contact joints along each rod, while preserving the geometric properties of the RF-structure. Our tool not only supports the design of wide variety of RF pattern classes and their variations, but also allows preview and refinement through interactive controls.
References:
1. Baverel, O., Nooshin, H., and Kuroiwa, Y. 2004. Configuration processing of nexorades using genetic algorithms. Jour. of the Intl. Assoc. for Shell and Spatial Structures 45, 2, 99–108.Google Scholar
2. Bertin, V. 2001. Variations of lever beam structures. In Proceedings of Conference on Growth and Form: The Engineering of Nature, School of Architecture, University of Waterloo.Google Scholar
3. Brocato, M., and Mondardini, L. 2010. Geometric methods and computational mechanics for the design of stone domes based on Abeille’s bond. In Advances in Architectural Geometry, Springer, 149–162.Google Scholar
4. Chilton, J. 1995. History of timber structures, Lecture E1. In STEP 2, Timber Engineering. STEP 2, Timber Engineering 2, E1–E13.Google Scholar
5. Chilton, J. 2009. Development of timber reciprocal frame structures in the UK. In Proceedings of IASS Symposium 2009: Evolution and trends in design, analysis and construction of shell and spatial structures, 1877–1884.Google Scholar
6. Douthe, C., and Baverel, O. 2009. Design of nexorades or reciprocal frame systems with the dynamic relaxation method. Computers & Structures 87, 21 (Nov.), 1296–1307. Google ScholarDigital Library
7. Eigensatz, M., Kilian, M., Schiftner, A., Mitra, N., Pottmann, H., and Pauly, M. 2010. Paneling architectural freeform surfaces. ACM Tran. on Graphics (SIGGRAPH) 29, 4, 45:1–45:10. Google ScholarDigital Library
8. Fu, C.-W., Lai, C.-F., He, Y., and Cohen-Or, D. 2010. K-set tileable surfaces. ACM Tran. on Graphics (SIGGRAPH) 29, 4, 44:1–44:6. Google ScholarDigital Library
9. Galassi, M., Davies, J., Theiler, J., Gough, B., Jungman, G., Alken, P., Booth, M., and Rossi, F. 2009. GNU Scientific Library Reference Manual. 3rd Ed., ISBN 0954612078.Google Scholar
10. Gelez, S., and Saby, V. 2011. Nexorades, facing an emergency situation. Intl. Jour. of Space Structures 26, 4 (Nov.), 359–362.Google Scholar
11. GRünbaum, B., and Shephard, G. C. 1986. Tilings and patterns. W. H. Freeman & Co. Google ScholarDigital Library
12. Igarashi, Y., Igarashi, T., and Mitani, J. 2012. Beady: interactive beadwork design and construction. ACM Tran. on Graphics (SIGGRAPH) 31, 4, 49:1–49:9. Google ScholarDigital Library
13. Kohlhammer, T., and Kotnik, T. 2010. Systemic behaviour of plane reciprocal frame structures. Structural Engineering Intl. 21, 1, 80–86.Google ScholarCross Ref
14. Larsen, O. P. 2008. Reciprocal Frame Structures. Elsevier Science and Technology.Google Scholar
15. Li, X.-Y., Shen, C.-H., Huang, S.-S., Ju, T., and Hu, S.-M. 2010. Popup: automatic paper architectures from 3D models. ACM Tran. on Graphics (SIGGRAPH) 29, 4, 111:1–111:9. Google ScholarDigital Library
16. Mitani, J., and Suzuki, H. 2004. Making papercraft toys from meshes using strip-based approximate unfolding. ACM Tran. on Graphics (SIGGRAPH) 23, 3, 259–263. Google ScholarDigital Library
17. Mori, Y., and Igarashi, T. 2007. Plushie: an interactive design system for plush toys. ACM Tran. on Graphics (SIGGRAPH) 26, 3, 45:1–45:8. Google ScholarDigital Library
18. Paczkowski, P., Kim, M. H., Morvan, Y., Dorsey, J., Rushmeier, H., and O’Sullivan, C. 2011. Insitu: sketching architectural designs in context. ACM Tran. on Graphics (SIGGRAPH ASIA) 30, 6, 182:1–182:10. Google ScholarDigital Library
19. Parigi, D., and Pugnale, A. 2012. Three-dimensional reciprocal structures: morphology, concepts, generative rules. In IASS-APCS Proc. from spatial structures to space structures.Google Scholar
20. Parigi, D., KirkeGaard, P. H., and Sassone, M. 2012. Hybrid optimization in the design of reciprocal structures. In Proceedings of the IASS Symposium 2012: from spatial structures to spaces structures. 8 pages.Google Scholar
21. Pugnale, A., Parigi, D., Kirkegaard, P. H., and Sassone, M. 2011. The principle of structural reciprocity: history, properties and design issues. In IASS: Intl. Conference on Space Structures, 414–421.Google Scholar
22. Sheffer, A., Lévy, B., Mogilnitsky, M., and Bogomyakov, A. 2005. ABF++: Fast and robust angle based flattening. ACM Tran. on Graphics 24, 2 (Apr.), 311–330. Google ScholarDigital Library
23. Singh, M., and Schaefer, S. 2010. Triangle surfaces with discrete equivalence classes. ACM Tran. on Graphics (SIGGRAPH) 29, 46:1–46:7. Google ScholarDigital Library
24. Thönnissen, U., and Werenfels, N. 2011. Reciprocal frames – teaching experiences. Intl. Jour. Of Space Structures 26, 4, 369–372. (Rhino-script developed by Prof. Annette Spiro).Google ScholarCross Ref
25. Umetani, N., Igarashi, T., and Mitra, N. 2012. Guided exploration of physically valid shapes for furniture design. ACM Tran. on Graphics (SIGGRAPH) 31, 4, 86:1–86:11. Google ScholarDigital Library
26. Whiting, E., Ochsendorf, J., and Durand, F. 2009. Procedural modeling of structurally-sound masonry buildings. ACM Tran. on Graphics (SIGGRAPH ASIA) 28, 5, 112:1–112:9. Google ScholarDigital Library
27. Whiting, E., Shin, H., Wang, R., Ochsendorf, J., and Durand, F. 2012. Structural optimization of 3D masonry buildings. ACM Tran. on Graphics (SIGGRAPH ASIA) 31, 6, 159:1–159:11. Google ScholarDigital Library
28. Xin, S.-Q., Lai, C.-F., Fu, C.-W., Wong, T.-T., He, Y., and Cohen-Or, D. 2011. Making burr puzzles from 3D models. ACM Tran. on Graphics (SIGGRAPH) 30, 4, 97:1–97:8. Google ScholarDigital Library
29. Yang, Y.-L., Yang, Y.-J., Pottmann, H., and Mitra, N. J. 2011. Shape space exploration of constrained meshes. ACM Tran. on Graphics (SIGGRAPH ASIA) 30, 6, 124:1–124:10. Google ScholarDigital Library