“Preserving topology and elasticity for embedded deformable models” by Nesme, Kry, Jeřábková and Faure
Conference:
Type(s):
Title:
- Preserving topology and elasticity for embedded deformable models
Presenter(s)/Author(s):
Abstract:
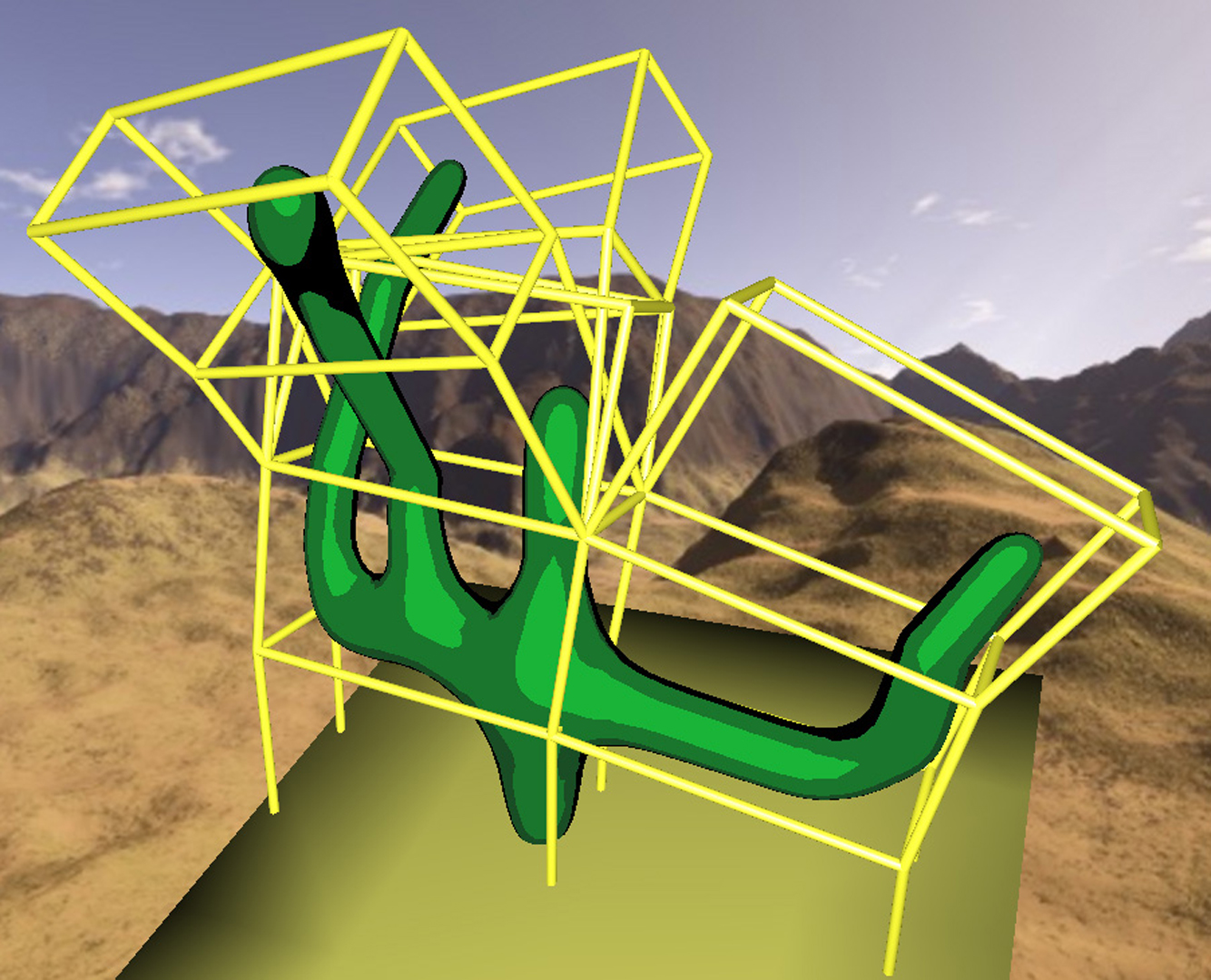
In this paper we introduce a new approach for the embedding of linear elastic deformable models. Our technique results in significant improvements in the efficient physically based simulation of highly detailed objects. First, our embedding takes into account topological details, that is, disconnected parts that fall into the same coarse element are simulated independently. Second, we account for the varying material properties by computing stiffness and interpolation functions for coarse elements which accurately approximate the behaviour of the embedded material. Finally, we also take into account empty space in the coarse embeddings, which provides a better simulation of the boundary. The result is a straightforward approach to simulating complex deformable models with the ease and speed associated with a coarse regular embedding, and with a quality of detail that would only be possible at much finer resolution.
References:
1. Baraff, D., and Witkin, A. 1998. Large steps in cloth simulation. In Proceedings of SIGGRAPH 1998, ACM, 43–54. Google ScholarDigital Library
2. Barbič J., and James D. L. 2005. Real-time subspace integration for St. Venant-Kirchhoff deformable models. ACM Transactions on Graphics 24, 3, 982–990. Google ScholarDigital Library
3. Bathe, K. 1982. Finite Element Procedures in Engineering Analysis. Prentice-Hall.Google Scholar
4. Belytschko, T., and Black, T. 1999. Elastic crack growth in finite elements with minimal remeshing. International Journal for Numerical Methods in Engineering 45, 5, 601–620.Google ScholarCross Ref
5. Botsch, M., Pauly, M., Wicke, M., and Gross, M. 2007. Adaptive space deformations based on rigid cells. Computer Graphics Forum 26, 3, 339–347.Google ScholarCross Ref
6. Bro-Nielsen, M., and Cotin, S. 1996. Real-time volumetric deformable models for surgery simulation using finite elements and condensation. In Computer Graphics Forum, 57–66.Google Scholar
7. Capell, S., Green, S., Curless, B., Duchamp, T., and Popović, Z. 2002. Interactive skeleton-driven dynamic deformations. ACM Transactions on Graphics 21, 3, 586–593. Google ScholarDigital Library
8. Debunne, G., Desbrun, M., Cani, M.-P., and Barr, A. H. 2001. Dynamic real-time deformations using space & time adaptive sampling. In Proceedings of SIGGRAPH 2001, ACM, 31–36. Google ScholarDigital Library
9. Faloutsos, P., van de Panne, M., and Terzopoulos, D. 1997. Dynamic free-form deformations for animation synthesis. IEEE Transactions on Visualization and Computer Graphics 3, 3, 201–214. Google ScholarDigital Library
10. Faure, F., Barbier, S., Allard, J., and Falipou, F. 2008. Image-based collision detection and response between arbitrary volumetric objects. In ACM Siggraph/Eurographics Symposium on Computer Animation. Google ScholarDigital Library
11. Galoppo, N., Otaduy, M. A., Mecklenburg, P., Gross, M., and Lin, M. C. 2006. Fast simulation of deformable models in contact using dynamic deformation textures. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 73–82. Google ScholarDigital Library
12. Grinspun, E., Krysl, P., and Schröder, P. 2002. CHARMS: A simple framework for adaptive simulation. ACM Transactions on Graphics 21, 3, 281–290. Google ScholarDigital Library
13. Hauth, M., and Strasser, W. 2004. Corotational simulation of deformable solids. In J. Winter School of Computer Graphics, vol. 12, 137–145.Google Scholar
14. Hsu, W. M., Hughes, J. F., and Kaufman, H. 1992. Direct manipulation of free-form deformations. In Computer Graphics (Proceedings of SIGGRAPH 92), ACM Press, New York, NY, USA, 177–184. Google ScholarDigital Library
15. James, D. L., and Pai, D. K. 1999. ArtDefo: Accurate real time deformable objects. In Proceedings of SIGGRAPH 1999, ACM Press / ACM SIGGRAPH, 65–72. Google ScholarDigital Library
16. James, D. L., and Pai, D. K. 2003. Multiresolution Green’s function methods for interactive simulation of large-scale elastostatic objects. ACM Transactions on Graphics 22, 1, 47–82. Google ScholarDigital Library
17. James, D. L., Barbič, J., and Twigg, C. D. 2004. Squashing cubes: Automating deformable model construction for graphics. In ACM SIGGRAPH Conference on Sketches & Applications. Google ScholarDigital Library
18. Jeřábková, L., and Kuhlen, T. 2009. Stable cutting of deformable objects in virtual environments using XFEM. In IEEE Computer Graphics and Applications, vol. 29, 61–71.Google ScholarCross Ref
19. Kaufmann, P., Martin, S., Botsch, M., and Gross, M. 2008. Flexible simulation of deformable models using discontinuous Galerkin FEM. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 105–115. Google ScholarDigital Library
20. Marchal, M., Allard, J., Duriez, C., and Cotin, S. 2008. Towards a framework for assessing deformable models in medical simulation. In International Symposium on Biomedical Simulation, 176–184. Google ScholarDigital Library
21. Martin, S., Kaufmann, P., Botsch, M., Wicke, M., and Gross, M. 2008. Polyhedral finite elements using harmonic basis functions. Computer Graphics Forum 27, 5, 1521–1529.Google ScholarDigital Library
22. Molino, N., Bao, Z., and Fedkiw, R. 2004. A virtual node algorithm for changing mesh topology during simulation. ACM Transactions on Graphics 23, 3, 385–392. Google ScholarDigital Library
23. Müller, M., Dorsey, J., McMillan, L., Jagnow, R., and Cutler, B. 2002. Stable real-time deformations. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 49–54. Google ScholarDigital Library
24. Müller, M., Teschner, M., and Gross, M. 2004. Physically-based simulation of objects represented by surface meshes. In IEEE Computer Graphics International, 26–33. Google ScholarDigital Library
25. Müller, M., Heidelberger, B., Teschner, M., and Gross, M. 2005. Meshless deformations based on shape matching. ACM Transactions on Graphics 24, 3, 471–478. Google ScholarDigital Library
26. Müller, M., Heidelberger, B., Hennix, M., and Ratcliff, J. 2006. Position based dynamics. In Eurographics Virtual Reality Interactions and Physical Simulations, 71–80.Google Scholar
27. Nealen, A., Müller, M., Keiser, R., Boxerman, E., and Carlson, M. 2005. Physically based deformable models in computer graphics. In Computer Graphics Forum, vol. 25 (4), 809–836.Google ScholarCross Ref
28. Nesme, M., Payan, Y., and Faure, F. 2006. Animating shapes at arbitrary resolution with non-uniform stiffness. In Eurographics Virtual Reality Interactions and Physical Simulations.Google Scholar
29. Pauly, M., Pai, D. K., and Guibas, L. J. 2004. Quasi-rigid objects in contact. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 109–119. Google ScholarDigital Library
30. Rivers, A. R., and James, D. L. 2007. FastLSM: Fast lattice shape matching for robust real-time deformation. ACM Transactions on Graphics 26, 3, 82:1–82:6. Google ScholarDigital Library
31. Sederberg, T. W., and Parry, S. R. 1986. Free-form deformation of solid geometric models. In Computer Graphics (Proceedings of SIGGRAPH 86), ACM, 151–160. Google ScholarDigital Library
32. Sifakis, E., Der, K. G., and Fedkiw, R. 2007. Arbitrary cutting of deformable tetrahedralized objects. In ACM SIGGRAPH/Eurographics Symp. on Computer Animation, 73–80. Google ScholarDigital Library
33. Sifakis, E., Shinar, T., Irving, G., and Fedkiw, R. 2007. Hybrid simulation of deformable solids. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 81–90. Google ScholarDigital Library
34. Terzopoulos, D., Platt, J., Barr, A., and Fleischer, K. 1987. Elastically deformable models. In Computer Graphics (Proceedings of SIGGRAPH 87), ACM, 205–214. Google ScholarDigital Library
35. Teschner, M., Heidelberger, B., Müller, M., and Gross, M. 2004. A versatile and robust model for geometrically complex deformable solids. In Computer Graphics International, 312–319. Google ScholarDigital Library
36. Wicke, M., Botsch, M., and Gross, M. 2007. A finite element method on convex polyhedra. Computer Graphics Forum 26, 3, 355–364.Google ScholarCross Ref
37. Wojtan, C., and Turk, G. 2008. Fast viscoelastic behavior with thin features. ACM Transactions on Graphics 27, 3, 47:1–47:8. Google ScholarDigital Library