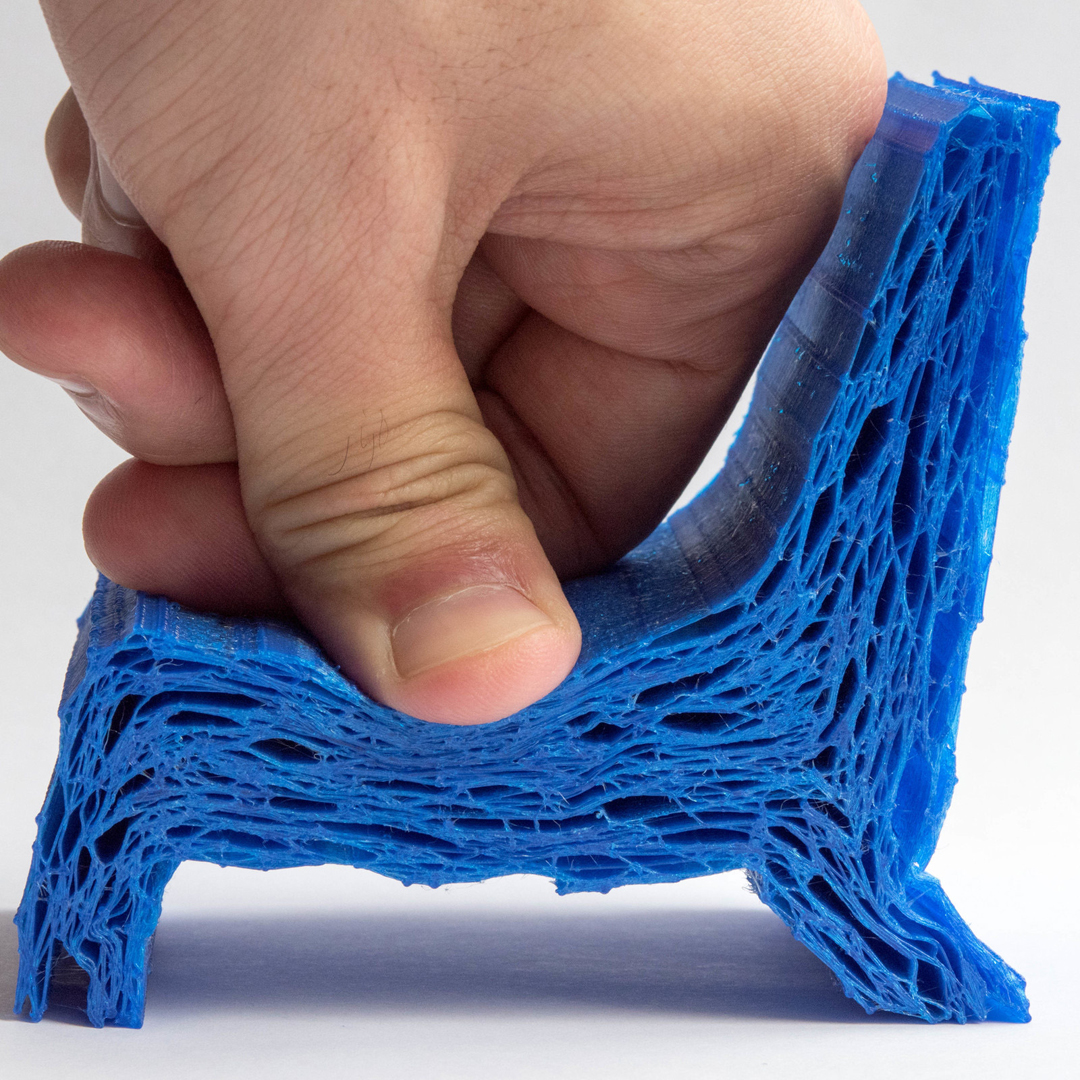
“Orthotropic k-nearest foams for additive manufacturing” by Martínez, Song, Dumas and Lefebvre
Conference:
Type(s):
Title:
- Orthotropic k-nearest foams for additive manufacturing
Session/Category Title: Fabricating Look & Feel
Presenter(s)/Author(s):
Moderator(s):
Abstract:
Additive manufacturing enables the fabrication of objects embedding meta-materials. By creating fine-scale structures, the object’s physical properties can be graded (e.g. elasticity, porosity), even though a single base material is used for fabrication. Designing the fine and detailed geometry of a metamaterial while attempting to achieve specific properties is difficult. In addition, the structures are intended to fill comparatively large volumes, which quickly leads to large data structures and intractable simulation costs. Thus, most metamaterials are defined as periodic structures repeated in regular lattices. The periodicity simplifies modeling, simulation, and reduces memory costs – however it limits the possibility to smoothly grade properties along free directions.In this work, we propose a novel metamaterial with controllable, freely orientable, orthotropic elastic behavior – orthotropy means that elasticity is controlled independently along three orthogonal axes, which leads to materials that better adapt to uneven, directional load scenarios, and offer a more versatile material design primitive. The fine-scale structures are generated procedurally by a stochastic process, and resemble a foam. The absence of global organization and periodicity allows the free gradation of density, orientation, and stretch, leading to the controllable orthotropic behavior. The procedural nature of the synthesis process allows it to scale to arbitrarily large volumes at low memory costs.We detail the foam structure synthesis, analyze and discuss its properties through numerical and experimental verifications, and finally demonstrate the use of orthotropic materials for the design of 3D printed objects.
References:
1. R. M. Jones. 1975. Mechanics of Composite Materials. Vol. 193. Scripta Book Company Washington, DC.Google Scholar
2. G. Allaire and G. A. Francfort. 1993. A Numerical Algorithm for Topology and Shape Optimization. Springer Netherlands, 239–248. Google ScholarCross Ref
3. L. J. Gibson and M. F. Ashby. 1997. Cellular Solids: Structure and Properties. Cambridge university press. Google ScholarCross Ref
4. G. Allaire. 2012. Shape Optimization by the Homogenization Method. Springer Science & Business Media.Google Scholar
5. E. Andreassen, B. S. Lazarov, and O. Sigmund. 2014. Design of Manufacturable 3D Extremal Elastic Microstructure. Mechanics of Materials 69, 1 (2014), 1–10. Google ScholarCross Ref
6. P. Balister, B. Bollobas, A. Sarkar, and M. Walters. 2005. Connectivity of Random k-nearest-neighbour Graphs. Advances in Applied Probability 37, 1 (2005), 1–24. Google ScholarCross Ref
7. M. P. Bendsøe. 1989. Optimal Shape Design as a Material Distribution Problem. Struct. Optimization 1, 4 (1989), 193–202. Google ScholarCross Ref
8. M. P. Bendsøe, J. Guedes, R. B. Haber, P. Pedersen, and J. Taylor. 1994. An Analytical Model to Predict Optimal Material Properties in the Context of Optimal Structural Design. J. Appl. Mech. 61, 4 (1994), 930–937. Google ScholarCross Ref
9. M. P. Bendsøe and N. Kikuchi. 1988. Generating Optimal Topologies in Structural Design Using a Homogenization Method. Computer Methods in Applied Mechanics and Engineering 71, 2 (1988), 197–224. Google ScholarDigital Library
10. V. Bentkus and F. Götze. 1997. On the Lattice Point Problem for Ellipsoids. Acta Arithmetica 80, 2 (1997), 101–125. http://pldml.icm.edu.pl/pldml/element/bwmeta1.element.bwnjournal-article-aav80i2p101bwmGoogle ScholarCross Ref
11. B. Bickel, M. Bächer, M. A. Otaduy, H. R. Lee, H. Pfister, M. Gross, and W. Matusik. 2010. Design and Fabrication of Materials With Desired Deformation Behavior. ACM Trans. Graph. 29, 4 (2010), 1. Google ScholarDigital Library
12. J. Brennan-Craddock. 2011. The Investigation of a Method to Generate Conformal Lattice Structures for Additive Manufacturing. Ph.D. Dissertation. Loughborough Univ. https://dspace.lboro.ac.uk/2134/9146Google Scholar
13. D. Chen, D. I. W. Levin, P. Didyk, P. Sitthi-Amorn, and W. Matusik. 2013. Spec2Fab: A Reducer-Tuner Model for Translating Specifications to 3D Prints. ACM Trans. Graph. 32, 4 (2013), 1. Google ScholarDigital Library
14. Y. Chen. 2007. 3D Texture Mapping for Rapid Manufacturing. Comput. Aided Des. Appl. 4, 6 (2007), 761–771. Google ScholarCross Ref
15. Q. Du and D. Wang. 2005. Anisotropic Centroidal Voronoi Tessellations and Their Applications. SIAM Journal on Scientific Computing 26, 3 (2005), 737–761. Google ScholarDigital Library
16. H. A. Eschenauer and N. Olhoff. 2001. Topology Optimization of Continuum Structures: A Review. Applied Mechanics Reviews 54, 4 (2001), 331. Google ScholarCross Ref
17. O. Fryazinov, T. Vilbrandt, and A. Pasko. 2013. Multi-Scale Space-Variant FRep Cellular Structures. Computer-Aided Design 45, 1 (2013), 26–34. Google ScholarDigital Library
18. G. H. Hardy. 1915. On the Expression of a Number as the Sum of Two Squares. Quart. J. Math. 46 (1915), 263–283.Google Scholar
19. A. Ion, J. Frohnhofen, L. Wall, R. Kovacs, M. Alistar, J. Lindsay, P. Lopes, H.-T. Chen, and P. Baudisch. 2016. Metamaterial Mechanisms. In Proceedings of the 29th Annual Symposium on User Interface Software and Technology – UIST ’16. Association for Computing Machinery (ACM), 529–539. Google ScholarDigital Library
20. S. G. Johnson. 2016. The NLopt Nonlinear-Optimization Package. http://ab-initio.mit.edu/nlopt. (2016).Google Scholar
21. M. Kočvara and M. Stingl. 2007. Free Material Optimization for Stress Constraints. Struct. Multidiscip. Opt. 33, 4 (2007), 323–335. Google ScholarCross Ref
22. B. Lévy and N. Bonneel. 2013. Variational Anisotropic Surface Meshing with Voronoi Parallel Linear Enumeration. In Proc. of the 21st International Meshing Roundtable. Springer Berlin Heidelberg. Google ScholarCross Ref
23. D. Li, N. Dai, X. Jiang, and X. Chen. 2015. Interior Structural Optimization Based on the Density-Variable Shape Modeling of 3D Printed Objects. The International Journal of Advanced Manufacturing Technology 83, 9 (2015), 1627–1635. Google ScholarCross Ref
24. Y. Li and J. Barbič. 2015. Stable Anisotropic Materials. IEEE Trans. Vis. Comput. Graph. 21, 10 (2015), 1129–1137. Google ScholarDigital Library
25. R. Lipton. 1994. Optimal Bounds on Effective Elastic Tensors for Orthotropic Composites. Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences 444, 1921 (1994), 399–410. Google ScholarCross Ref
26. L. Lu, B. Chen, A. Sharf, H. Zhao, Y. Wei, Q. Fan, X. Chen, Y. Savoye, C. Tu, and D. Cohen-Or. 2014. Build-To-Last: Strength to Weight 3D Printed Objects. ACM Trans. Graph. 33, 4 (2014), 1–10. Google ScholarDigital Library
27. J. Martínez, J. Dumas, and S. Lefebvre. 2016. Procedural Voronoi Foams for Additive Manufacturing. ACM Trans. Graph. 35, 4 (2016), 44:1–44:12. Google ScholarDigital Library
28. M. Moakher and A. N. Norris. 2006. The Closest Elastic Tensor of Arbitrary Symmetry to an Elasticity Tensor of Lower Symmetry. Journal of Elasticity 85, 3 (2006), 215–263. Google ScholarCross Ref
29. J. Panetta, Q. Zhou, L. Malomo, N. Pietroni, P. Cignoni, and D. Zorin. 2015. Elastic Textures for Additive Fabrication. ACM Trans. Graph. 34, 4 (2015), 135:1–135:12. Google ScholarDigital Library
30. O. Pantz and K. Trabelsi. 2008. A Post-Treatment of the Homogenization Method for Shape Optimization. SIAM Journal on Control and Optimization 47, 3 (2008), 1380–1398. Google ScholarDigital Library
31. A. Pasko, O. Fryazinov, T. Vilbrandt, P.-A. Fayolle, and V. Adzhiev. 2011. Procedural Function-Based Modelling of Volumetric Microstructures. Graphical Models 73, 5 (2011), 165–181. Google ScholarDigital Library
32. P. Pedersen. 1989. On Optimal Orientation of Orthotropic Materials. Struct. Optimization 1, 2 (1989), 101–106. Google ScholarCross Ref
33. A. Radman, X. Huang, and Y. M. Xie. 2013. Topology Optimization of Functionally Graded Cellular Materials. Journal of Materials Science 48, 4 (2013), 1503–1510. Google ScholarCross Ref
34. J. Robbins, S. Owen, B. Clark, and T. Voth. 2016. An Efficient and Scalable Approach for Generating Topologically Optimized Cellular Structures for Additive Manufacturing. Additive Manufacturing 12, B (2016), 296–304. Google ScholarCross Ref
35. A. P. Roberts and E. J. Garboczi. 2002. Elastic Properties of Model Random Three-Dimensional Open-Cell Solids. J. Mech. Phys. Solids 50, 1 (2002), 33–55. Google ScholarCross Ref
36. D. W. Rosen. 2007. Computer-Aided Design for Additive Manufacturing of Cellular Structures. Computer-Aided Design and Applications 4, 5 (2007), 585–594. Google ScholarCross Ref
37. G. I. N. Rozvany. 2001. Aims, Scope, Methods, History and Unified Terminology of Computer-Aided Topology Optimization in Structural Mechanics. Struct. Multidiscip. Opt. 21, 2 (2001), 90–108. Google ScholarDigital Library
38. C. Schumacher, B. Bickel, J. Rys, S. Marschner, C. Daraio, and M. Gross. 2015. Microstructures to Control Elasticity in 3D Printing. ACM Trans. Graph. 34, 4 (2015), 136:1–136:13. Google ScholarDigital Library
39. O. Sigmund. 1994. Materials With Prescribed Constitutive Parameters: An Inverse Homogenization Problem. Int. J. Solids Struct. 31, 17 (1994), 2313–2329. Google ScholarCross Ref
40. O. Sigmund. 1995. Tailoring Materials With Prescribed Elastic Properties. Mechanics of Materials 20, 4 (1995), 351–368. Google ScholarCross Ref
41. O. Sigmund and S. Torquato. 1999. Design of Smart Composite Materials Using Topology Optimization. Smart Materials and Structures 8, 3 (1999), 365. http://stacks.iop.org/0964-1726/8/i=3/a=308Google ScholarCross Ref
42. M. Skouras, B. Thomaszewski, S. Coros, B. Bickel, and M. Gross. 2013. Computational Design of Actuated Deformable Characters. ACM Trans. Graph. 32, 4 (2013), 1. Google ScholarDigital Library
43. K. Suzuki and N. Kikuchi. 1991. A Homogenization Method for Shape and Topology Optimization. Comput. Method. Appl. M. 93, 3 (1991), 291–318. Google ScholarCross Ref
44. T. Ting. 1996. Positive Definiteness of Anisotropic Elastic Constants. Math. Mech. Solids 1, 3 (1996), 301–314. Google ScholarCross Ref
45. T. C. T. Ting and T. Chen. 2005. Poisson’s Ratio for Anisotropic Elastic Materials Can Have No Bounds. Q. J. Mech. Appl. Math. 58, 1 (2005), 73–82. Google ScholarCross Ref
46. K. Vidimče, A. Kaspar, Y. Wang, and W. Matusik. 2016. Foundry: Hierarchical Material Design for Multi-Material Fabrication. In Proc. of the 29th Annual Symposium on User Interface Software and Technology—UIST’16. ACM, New York, NY, USA, 563–574. Google ScholarDigital Library
47. K. Vidimče, S.-P. Wang, J. Ragan-Kelley, and W. Matusik. 2013. OpenFab: A Programmable Pipeline for Multi-Material Fabrication. ACM Trans. Graph. 32, 4 (2013), 1. Google ScholarDigital Library
48. H. Wang, Y. Chen, and D. W. Rosen. 2005. A Hybrid Geometric Modeling Method for Large Scale Conformal Cellular Structures. In Volume 3: 25th Computers and Information in Engineering Conference, Parts A and B. 421–427. Google ScholarCross Ref
49. W. Wang, T. Y. Wang, Z. Yang, L. Liu, X. Tong, W. Tong, J. Deng, F. Chen, and X. Liu. 2013. Cost-Effective Printing of 3D Objects With Skin-Frame Structures. ACM Trans. Graph. 32, 6 (2013), 1–10. Google ScholarDigital Library
50. S. Worley. 1996. A Cellular Texture Basis Function. In Proceedings of the 23rd annual conference on Computer graphics and interactive techniques – SIGGRAPH ’96. ACM, New York, NY, USA, 291–294. Google ScholarDigital Library
51. J. Wu, N. Aage, R. Westermann, and O. Sigmund. 2016a. Infill Optimization for Additive Manufacturing-Approaching Bone-Like Porous Structures. arXiv (2016). http://arxiv.org/abs/1608.04366Google Scholar
52. J. Wu, C. C. Wang, X. Zhang, and R. Westermann. 2016b. Self-Supporting Rhombic Infill Structures for Additive Manufacturing. Computer-Aided Design 80 (2016), 32–42. Google ScholarCross Ref
53. L. Xia and P. Breitkopf. 2015. Design of Materials Using Topology Optimization and Energy-Based Homogenization Approach in Matlab. Struct. Multidiscip. Opt. 52, 6 (2015), 1229–1241. Google ScholarDigital Library
54. H. Xu, Y. Li, Y. Chen, and J. Barbič. 2015. Interactive Material Design Using Model Reduction. ACM Trans. Graph. (TOG) 34, 2 (2015), 18. Google ScholarDigital Library
55. F. Xue and P. Kumar. 2004. The Number of Neighbors Needed for Connectivity of Wireless Networks. Wireless Networks 10, 2 (2004), 169–181. Google ScholarDigital Library
56. X. Zhang, Y. Xia, J. Wang, Z. Yang, C. Tu, and W. Wang. 2015. Medial Axis Tree—an Internal Supporting Structure for 3D Printing. Computer Aided Geometric Design 35–36 (2015), 149–162. Google ScholarDigital Library
57. S. Zhou and Q. Li. 2008. Design of Graded Two-Phase Microstructures for Tailored Elasticity Gradients. Journal of Materials Science 43, 15 (2008), 5157–5167. Google ScholarCross Ref