“Optimal gait and form for animal locomotion” by Wampler and Popovic
Conference:
Type(s):
Title:
- Optimal gait and form for animal locomotion
Presenter(s)/Author(s):
Abstract:
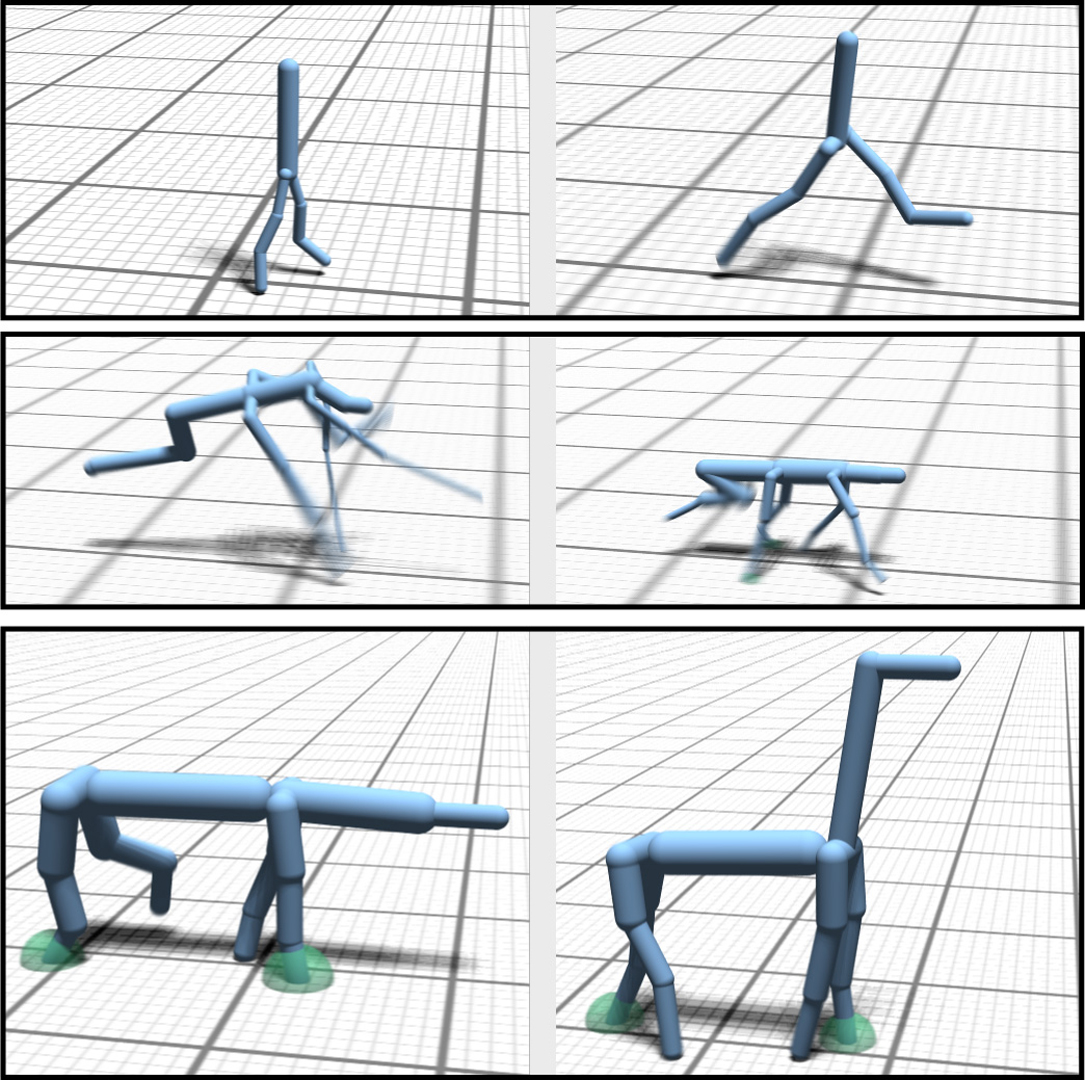
We present a fully automatic method for generating gaits and morphologies for legged animal locomotion. Given a specific animal’s shape we can determine an efficient gait with which it can move. Similarly, we can also adapt the animal’s morphology to be optimal for a specific locomotion task. We show that determining such gaits is possible without the need to specify a good initial motion, and without manually restricting the allowed gaits of each animal. Our approach is based on a hybrid optimization method which combines an efficient derivative-aware spacetime constraints optimization with a derivative-free approach able to find non-local solutions in high-dimensional discontinuous spaces. We demonstrate the effectiveness of this approach by synthesizing dynamic locomotions of bipeds, a quadruped, and an imaginary five-legged creature.
References:
1. Alexander, M. 1996. Optima for Animals. Princeton University Press.Google Scholar
2. Auslander, J., Fukunaga, A., Partovi, H., Christensen, J., Hsu, L., Reiss, P., Shuman, A., Marks, J., and Ngo, J. T. 1995. Further experiences with controller-based automatic motion synthesis for articulated figures. ACM Transactions on Graphics 14, 4 (Oct.), 311–336. Google ScholarDigital Library
3. Fang, A. C., and Pollard, N. S. 2003. Efficient synthesis of physically valid human motion. ACM Trans. Graph. 22, 3, 417–426. Google ScholarDigital Library
4. Gill, P. E., Murray, W., and Saunders, M. A. 2005. Snopt: An sqp algorithm for large-scale constrained optimization. SIAM Review 47, 1, 99–131. Google ScholarDigital Library
5. Grzeszczuk, R., Terzopoulos, D., and Hinton, G. 1998. Neuroanimator: Fast neural network emulation and control of physics-based models. In Proceedings of SIGGRAPH 98, Computer Graphics Proceedings, Annual Conference Series, 9–20. Google ScholarDigital Library
6. Guenter, B. 2007. Efficient symbolic differentiation for graphics applications. ACM Trans. Graph. 26, 3, 108. Google ScholarDigital Library
7. Häkkinen, K., and Keskinen, K. L. 2006. Muscle cross-sectional area and voluntary force production characteristics in elite strength- and endurance-trained athletes and sprinters. European Journal of Applied Physiology (Apr), 215–220.Google Scholar
8. Hansen, N., and Kern, S. 2004. Evaluating the CMA evolution strategy on multimodal test functions. In Parallel Problem Solving from Nature PPSN VIII, Springer, X. Yao et al., Eds., vol. 3242 of LNCS, 282–291.Google Scholar
9. Hansen, N., Hansen, N., Ostermeier, A., and Ostermeier, A. 1996. Adapting arbitrary normal mutation distributions in evolution strategies: the covariance matrix adaptation. Morgan Kaufmann, 312–317.Google Scholar
10. Hecker, C., Raabe, B., Enslow, R. W., Deweese, J., Maynard, J., and van Prooijen, K. 2008. Real-time motion retargeting to highly varied user-created morphologies. ACM Trans. Graph. 27, 3, 1–11. Google ScholarDigital Library
11. Hodgins, J. K., and Pollard, N. S. 1997. Adapting simulated behaviors for new characters. In Proceedings of SIGGRAPH 97, 153–162. Google ScholarDigital Library
12. Hutchinson, J. R., and Gatesy, S. M. 2006. Dinosaur locomotion: Beyond the bones. Nature 440, 7082 (Mar), 292–294.Google ScholarCross Ref
13. Koh, B.-I., Reinbolt, J. A., George, A. D., Haftka, R. T., and Fregly, B. J. 2008. Limitations of parallel global optimization for large-scale human movement problems. Medical Engineering and Physics.Google Scholar
14. Kry, P. G., Reveret, L., Faure, F., and Cani, M.-P. 2009. Modal locomotion: Animating virtual characters with natural vibrations. Computer Graphics Forum.Google Scholar
15. Liu, Z., Gortler, S. J., and Cohen, M. F. 1994. F.: Hierarchical spacetime control of linked figures. In In Proceedings of the 21st annual conference on Computer graphics and interactive techniques, ACM Press, 35–42. Google ScholarDigital Library
16. Liu, C. K., Hertzmann, A., and Popović, Z. 2005. Learning physics-based motion style with nonlinear inverse optimization. ACM Trans. Graph. 24, 3, 1071–1081. Google ScholarDigital Library
17. Liu, C. K., Hertzmann, A., and Popović, Z. 2006. Composition of complex optimal multi-character motions. In SCA ’06: Proceedings of the 2006 ACM SIGGRAPH/Eurographics symposium on Computer animation, Eurographics Association, Aire-la-Ville, Switzerland, Switzerland, 215–222. Google ScholarDigital Library
18. Paul, A., and Bongard, J. C. 2001. The road less travelled: Morphology in the optimization of biped robot locomotion. In In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS2001, IEEE Press, 226–232.Google Scholar
19. Popović, Z., and Witkin, A. 1999. Physically based motion transformation. In SIGGRAPH ’99: Proceedings of the 26th annual conference on Computer graphics and interactive techniques, ACM Press/Addison-Wesley Publishing Co., New York, NY, USA, 11–20. Google ScholarDigital Library
20. Raibert, M. H., and Hodgins, J. K. 1991. Animation of dynamic legged locomotion. In Computer Graphics (Proceedings of SIGGRAPH 91), vol. 25, 349–358. Google ScholarDigital Library
21. Rose, C., Guenter, B., Bodenheimer, B., and Cohen, M. F. 1996. Efficient generation of motion transitions using spacetime constraints. 147–154.Google Scholar
22. Safonova, A., Hodgins, J. K., and Pollard, N. S. 2004. Synthesizing physically realistic human motion in low-dimensional, behavior-specific spaces. ACM Trans. Graph. 23, 3, 514–521. Google ScholarDigital Library
23. Sims, K. 1994. Evolving virtual creatures. In SIGGRAPH ’94: Proceedings of the 21st annual conference on Computer graphics and interactive techniques, ACM, New York, NY, USA, 15–22. Google ScholarDigital Library
24. Srinivasan, M., and Ruina, A. 2006. Computer optimization of a minimal biped model discovers walking and running. Nature 439, 7072 (Jan), 72–75.Google ScholarCross Ref
25. Thompson, D. W. 1992. On Growth and Form: The Complete Revised Edition. Dover.Google Scholar
26. van de Panne, M. 1996. Parameterized gait synthesis. IEEE Comput. Graph. Appl. 16, 2, 40–49. Google ScholarDigital Library
27. Weibel, E. R., Tayor, C. R., and Bolis, L. 1998. Principles of Animal Design. Cambridge University Press.Google Scholar
28. Witkin, A., and Kass, M. 1988. Spacetime constraints. In SIGGRAPH ’88: Proceedings of the 15th annual conference on Computer graphics and interactive techniques, ACM, New York, NY, USA, 159–168. Google ScholarDigital Library