“Modified subdivision surfaces with continuous curvature” by Levin

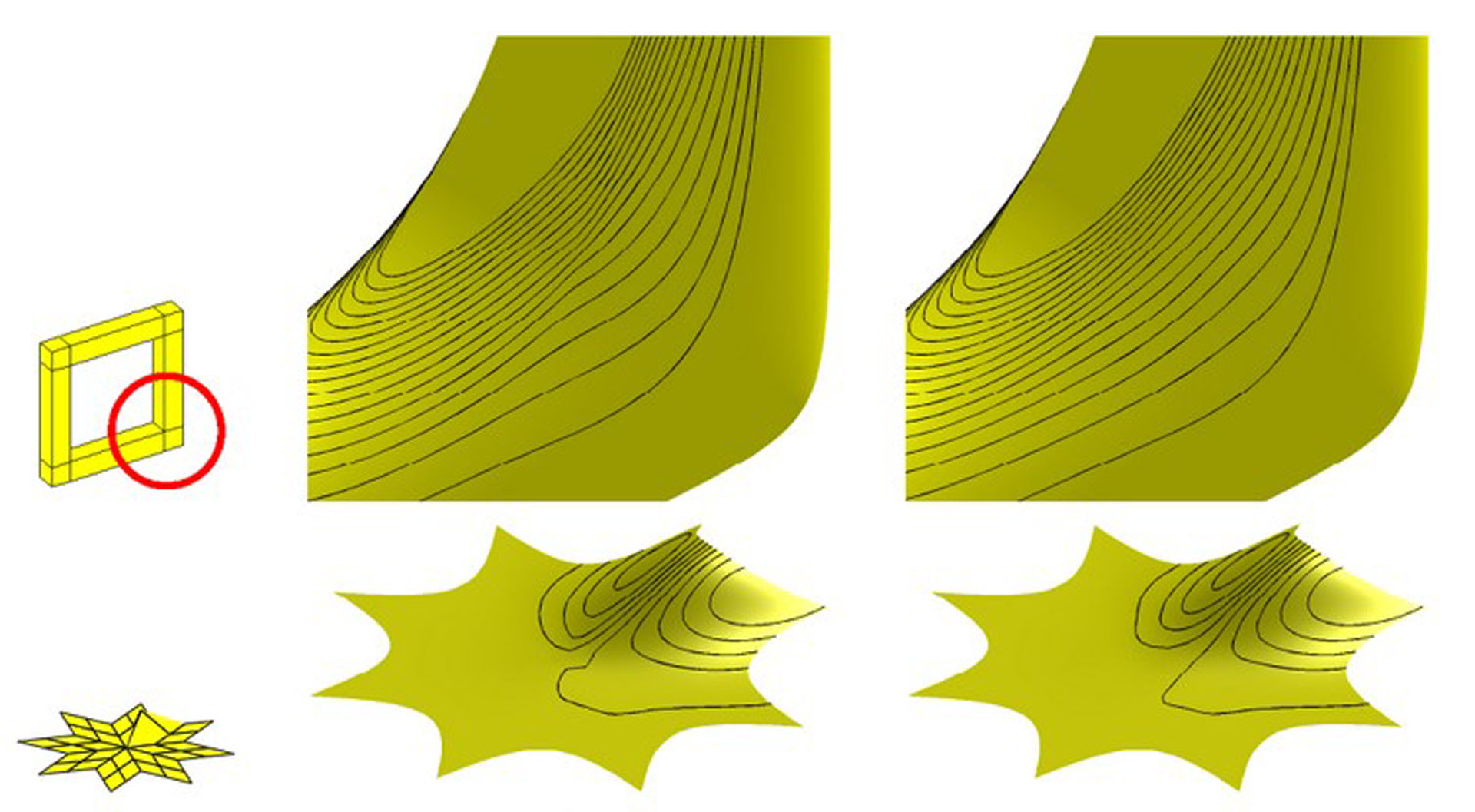
We present a modification to subdivision surfaces, which guarantees second-order smoothness everywhere in the surface, including extraordinary points. The idea is to blend the limit surface with a low degree polynomial defined over the characteristic map, in the vicinity of each extraordinary point. We demonstrate our method on Catmull-Clark surfaces, but a similar modification can be applied to other schemes as well. The proposed modification to Catmull-Clark is simple to implement and can be applied to quad meshes of arbitrary topological type, even when extraordinary vertices share edges.

1. Barthe, L., and Kobbelt, L. 2004. Subdivision scheme tuning around extraordinary vertices. Computer Aided Geometric Design 21, 6, 561–583. Google ScholarDigital Library
2. Biermann, H., Levin, A., and Zorin, D. 2000. Piecewise smooth subdivision surfaces with normal control. In Proceedings of ACM SIGGRAPH 2000, Annual Conference Series, ACM, 113–120. Google ScholarDigital Library
3. Catmull, E., and Clark, J. 1978. Recursively generated b-spline surfaces on arbitrary topological meshes. Computer Aided Design 10, 350–355.Google ScholarCross Ref
4. DeRose, T., Kass, M., and Truong, T. 1998. Subdivision surfaces in character animation. In Proceedings of ACM SIGGRAPH 98, Annual Conference Series, ACM, 85–94. Google ScholarDigital Library
5. Karĉiauskas, K., Peters, J., and Reif, U. 2004. Shape characterization of subdivision surfaces: case studies. Computer Aided Geometric Design 21, 6, 601–614. Google ScholarDigital Library
6. Kobbelt, L. 2000. √3-subdivision. In Proceedings of ACM SIGGRAPH 2000, Annual Conference Series, ACM, 103–112. Google ScholarDigital Library
7. Loop, C. 1987. Smooth spline surfaces based on triangles. Master’s thesis, University of Utah, Department of Mathematics.Google Scholar
8. Loop, C. 2002. Bounded curvature triangular mesh subdivision with the convex hull property. The Visual Computer 18, (5-6), 316–325.Google ScholarCross Ref
9. Loop, C. 2004. Second order smoothness over extraordinary vertices. In Proceedings of the Symposium on Geometry Processing, Nice, France, 169–178. Google ScholarDigital Library
10. Navau, J. C., and Garcia, N. P. 2000. Modeling surfaces from meshes of arbitrary topology. Computer Aided Geometric Design 17, 7, 643–671. Google ScholarDigital Library
11. Peters, J. 1996. Curvature continuous spline surfaces over irregular meshes. Computer Aided Geometric Design 13, 2, 101–131. Google ScholarDigital Library
12. Peters, J. 2002. C2 free-form surfaces of degree (3, 5). Computer Aided Geometric Design 19, 2, 113–126. Google ScholarDigital Library
13. Prautzsch, H., and Reif, U. 1999. Degree estimates of Ck piecewise polynomial subdivision surfaces. Advances in Computational Mathematics 10, 209–217.Google ScholarCross Ref
14. Prautzsch, H., and Umlauf, G. 1998. A G2 subdivision algorithm. In Geometric Modeling, Computing Supplements, G. Farin, H. Bieri, G. Brunnet, and T. DeRose, Eds. New York: Springer-Verlag, 217–224. Google ScholarDigital Library
15. Prautzsch, H., and Umlauf, G. 2000. Triangular G2 splines. In Curves and Surfaces in Design, P. J. Laurent, P. Sablonniere, and L. L. Schumaker, Eds. Vanderbilt University Press, 335–342.Google Scholar
16. Prautzsch, H. 1997. Freeform splines. Computer Aided Geometric Design 14, 3, 201–206. Google ScholarDigital Library
17. Reif, U. 1995. A unified approach to subdivision algorithms near extraordinary points. Computer Aided Geometric Design 12, 2, 153–174. Google ScholarDigital Library
18. Reif, U. 1998. TURBS: topologically unrestricted b-splines. Constructive Approximation 4, 55–77.Google Scholar
19. Sabin, M., and Barthe, L. 2003. Artifacts in recursive subdivision surfaces. In Curve and Surface Fitting: St Malo 2002, Nashboro Press, A. Cohen, J.-L. Merrien, and L. L. Schumaker, Eds., 353–362.Google Scholar
20. Sabin, M. 1991. Cubic recursive division with bounded curvature. In Curves and Surfaces, P. J. Laurent, A. le Mehaute, and L. L. Schumaker, Eds. Academic Press, 411–414. Google ScholarDigital Library
21. Stam, J. 1998. Exact evaluation of Catmull-Clark subdivision surfaces at arbitrary parameter values. In Proceedings of ACM SIGGRAPH 98, Annual Conference Series, ACM, 395–404. Google ScholarDigital Library
22. Warren, J., and Weimer, H. 2002. Subdivision Methods for Geometric Design. Morgan Kaufmann. Google ScholarDigital Library
23. Ying, L., and Zorin, D. 2004. A simple manifold-based construction of surfaces of arbitrary smoothness. ACM Transactions on Graphics 23, 3, 271–275. Google ScholarDigital Library
24. Zorin, D. 2000. Smoothness of stationary subdivision on irregular meshes. Constructive Approximation 16, 359–398.Google ScholarCross Ref
25. Zorin, D. 2000. Constructing curvature-continuous surfaces by blending. NYU, Technical Report.Google Scholar
26. Zulti, A., Levin, A., Levin, D., and Teicher, M. 2006. C2 subdivision over triangulations with one extraordinary point. Computer Aided Geometric Design 23, 2, 157–178. Google ScholarDigital Library