“iWIRES: an analyze-and-edit approach to shape manipulation” by Gal, Sorkine-Hornung, Mitra and Cohen-Or

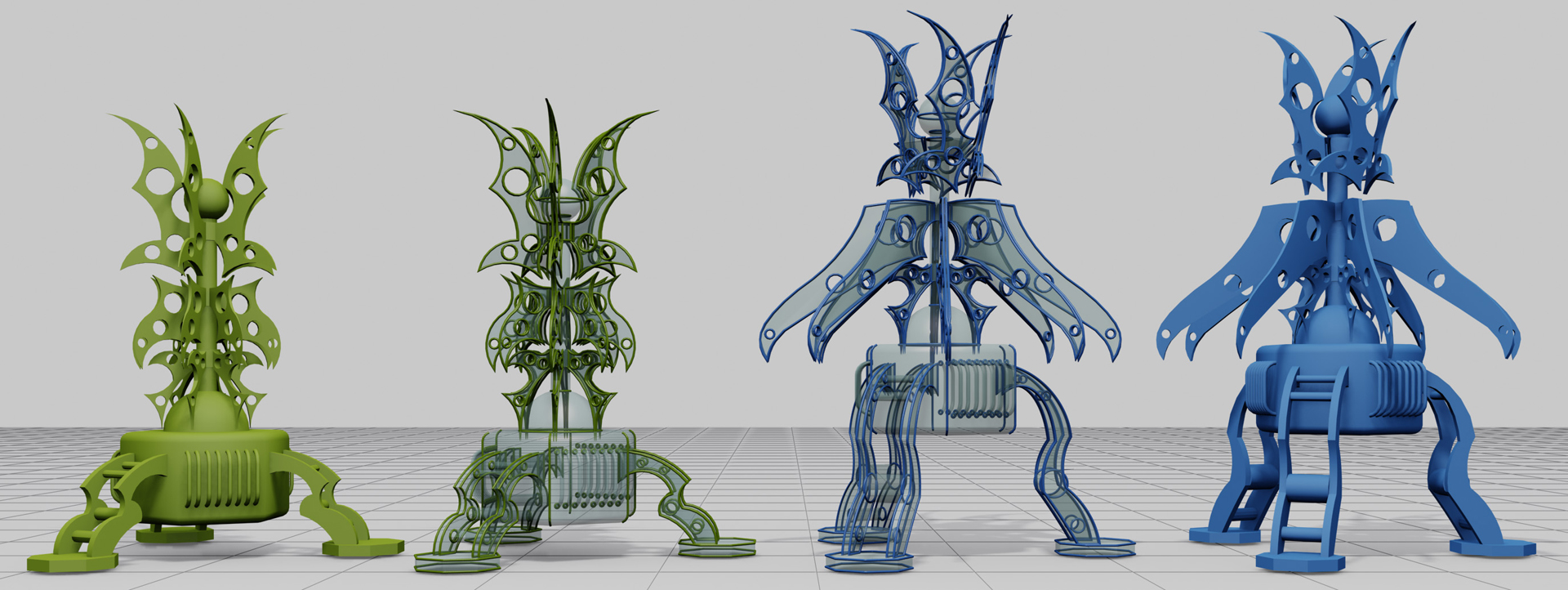
Man-made objects are largely dominated by a few typical features that carry special characteristics and engineered meanings. State-of-the-art deformation tools fall short at preserving such characteristic features and global structure. We introduce iWIRES, a novel approach based on the argument that man-made models can be distilled using a few special 1D wires and their mutual relations. We hypothesize that maintaining the properties of such a small number of wires allows preserving the defining characteristics of the entire object. We introduce an analyze-and-edit approach, where prior to editing, we perform a light-weight analysis of the input shape to extract a descriptive set of wires. Analyzing the individual and mutual properties of the wires, and augmenting them with geometric attributes makes them intelligent and ready to be manipulated. Editing the object by modifying the intelligent wires leads to a powerful editing framework that retains the original design intent and object characteristics. We show numerous results of manipulation of man-made shapes using our editing technique.

1. Angelidis, A., Cani, M.-P., Wyvill, G., and King, S. 2004. Swirling-sweepers: Constant-volume modeling. In Proc. of Pacific Graphics, 10–15. Google ScholarDigital Library
2. Attene, M., Robbiano, F., Spagnuolo, M., and Falcidieno, B. 2007. Semantic annotation of 3D surface meshes based on feature characterization. Lecture Notes in Computer Science 4816, 126–139. Google ScholarDigital Library
3. Au, O. K.-C., Fu, H., Tai, C.-L., and Cohen-Or, D. 2007. Handle-aware isolines for scalable shape editing. ACM Trans. Graph. 26, 3, 83. Google ScholarDigital Library
4. Benkö, P., Martin, R. R., and Várady, T. 2001. Algorithms for reverse engineering boundary representation models. Computer Aided Design 33, 11, 839–851.Google ScholarCross Ref
5. Botsch, M., and Kobbelt, L. 2003. Multiresolution surface representation based on displacement volumes. In Proc. of Eurographics, 483–491.Google Scholar
6. Botsch, M., and Kobbelt, L. 2005. Real-time shape editing using radial basis functions. In Proc. of Eurographics, 611–621.Google Scholar
7. Botsch, M., and Sorkine, O. 2008. On linear variational surface deformation methods. IEEE Trans. on Visualization and Computer Graphics 14, 1, 213–230. Google ScholarDigital Library
8. Botsch, M., Pauly, M., Gross, M., and Kobbelt, L. 2006. PriMo: Coupled prisms for intuitive surface modeling. In Proc. of Sym. on Geometry Processing, 11–20. Google ScholarDigital Library
9. Botsch, M., Pauly, M., Wicke, M., and Gross, M. 2007. Adaptive space deformations based on rigid cells. In Proc. of Eurographics, 339–347.Google Scholar
10. Cabral, M., Lefebvre, S., Dachsbacher, C., and Drettakis, G. 2009. Structure preserving reshape for textured architectural scenes. In Proc. of Eurographics, 469–480.Google Scholar
11. Coleman, T., and Li, Y. 1996. An interior, trust region approach for nonlinear minimization subject to bounds. SIAM Journal on Optimization 6, 418–445.Google ScholarCross Ref
12. Coquillart, S. 1990. Extended free-form deformation: A sculpturing tool for 3D geometric modeling. In Proc. of ACM SIGGRAPH, 187–196. Google ScholarDigital Library
13. Funkhouser, T., Kazhdan, M., Shilane, P., Min, P., Kiefer, W., Tal, A., Rusinkiewicz, S., and Dobkin, D. 2004. Modeling by example. ACM Trans. Graph. 23, 3, 652–663. Google ScholarDigital Library
14. Huang, J., Shi, X., Liu, X., Zhou, K., Wei, L.-Y., Teng, S., Bao, H., Guo, B., and Shum, H.-Y. 2006. Subspace gradient domain mesh deformation. ACM Trans. Graph. 25, 3, 1126–1134. Google ScholarDigital Library
15. Joshi, P., Meyer, M., DeRose, T., Green, B., and Sanocki, T. 2007. Harmonic coordinates for character articulation. ACM Trans. Graph. 26, 3, #71. Google ScholarDigital Library
16. Ju, T., Schaefer, S., and Warren, J. 2005. Mean value coordinates for closed triangular meshes. ACM Trans. Graph. 24, 3, 561–566. Google ScholarDigital Library
17. Kraevoy, V., Sheffer, A., Cohen-Or, D., and Shamir, A. 2008. Non-homogeneous resizing of complex models. ACM Trans. Graph. 27, 5, #111. Google ScholarDigital Library
18. Lee, Y., and Lee, S. 2002. Geometric snakes for triangular meshes. In Proc. of Eurographics, 229–238.Google Scholar
19. Lipman, Y., Sorkine, O., Levin, D., and Cohen-Or, D. 2005. Linear rotation-invariant coordinates for meshes. ACM Trans. Graph. 24, 3, 479–487. Google ScholarDigital Library
20. Lipman, Y., Cohen-Or, D., Gal, R., and Levin, D. 2007. Volume and shape preservation via moving frame manipulation. ACM Trans. Graph. 26, 1. Google ScholarDigital Library
21. Lipman, Y., Levin, D., and Cohen-Or, D. 2008. Green coordinates. ACM Trans. Graph. 27, 3. Google ScholarDigital Library
22. Masuda, H., and Ogawa, K. 2007. Application of interactive deformation to assembled mesh models for CAE analysis. In ASME Int. Design Engineering Technical Conferences.Google Scholar
23. Masuda, H., Yoshioka, Y., and Furukawa, Y. 2007. Preserving form features in interactive mesh deformation. Computer Aided Design 39, 5, 361–368. Google ScholarDigital Library
24. Milliron, T., Jensen, R. J., Barzel, R., and Finkelstein, A. 2002. A framework for geometric warps and deformations. ACM Trans. Graph. 21, 1, 20–51. Google ScholarDigital Library
25. Mitra, N. J., Guibas, L., and Pauly, M. 2006. Partial and approximate symmetry detection for 3D geometry. ACM Trans. Graph. 25, 3, 560–568. Google ScholarDigital Library
26. Nealen, A., Igarashi, T., Sorkine, O., and Alexa, M. 2007. FiberMesh: Designing freeform surfaces with 3D curves. ACM Trans. Graph. 26, 3, 41. Google ScholarDigital Library
27. Ohtake, Y., Belyaev, A., and Seidel, H.-P. 2004. Ridgevalley lines on meshes via implicit surface fitting. ACM Trans. Graph. 23, 3, 609–612. Google ScholarDigital Library
28. Orzan, A., Bousseau, A., Winnemöller, H., Barla, P., Thollot, J., and Salesin, D. 2008. Diffusion curves: a vector representation for smooth-shaded images. ACM Trans. Graph. 27, 3. Google ScholarDigital Library
29. Pauly, M., Mitra, N. J., Wallner, J., Pottmann, H., and Guibas, L. 2008. Discovering structural regularity in 3D geometry. ACM Trans. Graph. 27, 3, #43, 1–11. Google ScholarDigital Library
30. Popa, T., Julius, D., and Sheffer, A. 2007. Interactive and linear material aware deformations. Proc. of Shape Modeling International 13, 1, 73–100. Google ScholarDigital Library
31. Sederberg, T. W., and Parry, S. R. 1986. Free-form deformation of solid geometric models. In Proc. of ACM SIGGRAPH, 151–160. Google ScholarDigital Library
32. Shi, X., Zhou, K., Tong, Y., Desbrun, M., Bao, H., and Guo, B. 2007. Mesh puppetry: cascading optimization of mesh deformation with inverse kinematics. ACM Trans. Graph. 26, 3. Google ScholarDigital Library
33. Singh, K., and Fiume, E. 1998. Wires: a geometric deformation technique. In Proc. of ACM SIGGRAPH, 405–414. Google ScholarDigital Library
34. Sorkine, O., and Alexa, M. 2007. As-rigid-as-possible surface modeling. In Proc. of Sym. on Geometry Processing, 109–116. Google ScholarDigital Library
35. Sorkine, O., Lipman, Y., Cohen-Or, D., Alexa, M., Rössl, C., and Seidel, H.-P. 2004. Laplacian surface editing. In Proc. of Sym. on Geometry Processing, 179–188. Google ScholarDigital Library
36. Sumner, R. W., Schmid, J., and Pauly, M. 2007. Embedded deformation for shape manipulation. ACM Trans. Graph. 26, 3. Google ScholarDigital Library
37. von Funck, W., Theisel, H., and Seidel, H.-P. 2006. Vector field based shape deformations. ACM Trans. Graph. 25, 3. Google ScholarDigital Library
38. Zhou, K., Huang, J., Snyder, J., Liu, X., Bao, H., Guo, B., and Shum, H.-Y. 2005. Large mesh deformation using the volumetric graph Laplacian. ACM Trans. Graph. 24, 3, 496–503. Google ScholarDigital Library
39. Zimmermann, J., Nealen, A., and Alexa, M. 2007. SilSketch: automated sketch-based editing of surface meshes. In Proc. of Sketch-based Interfaces and Modeling, 23–30. Google ScholarDigital Library