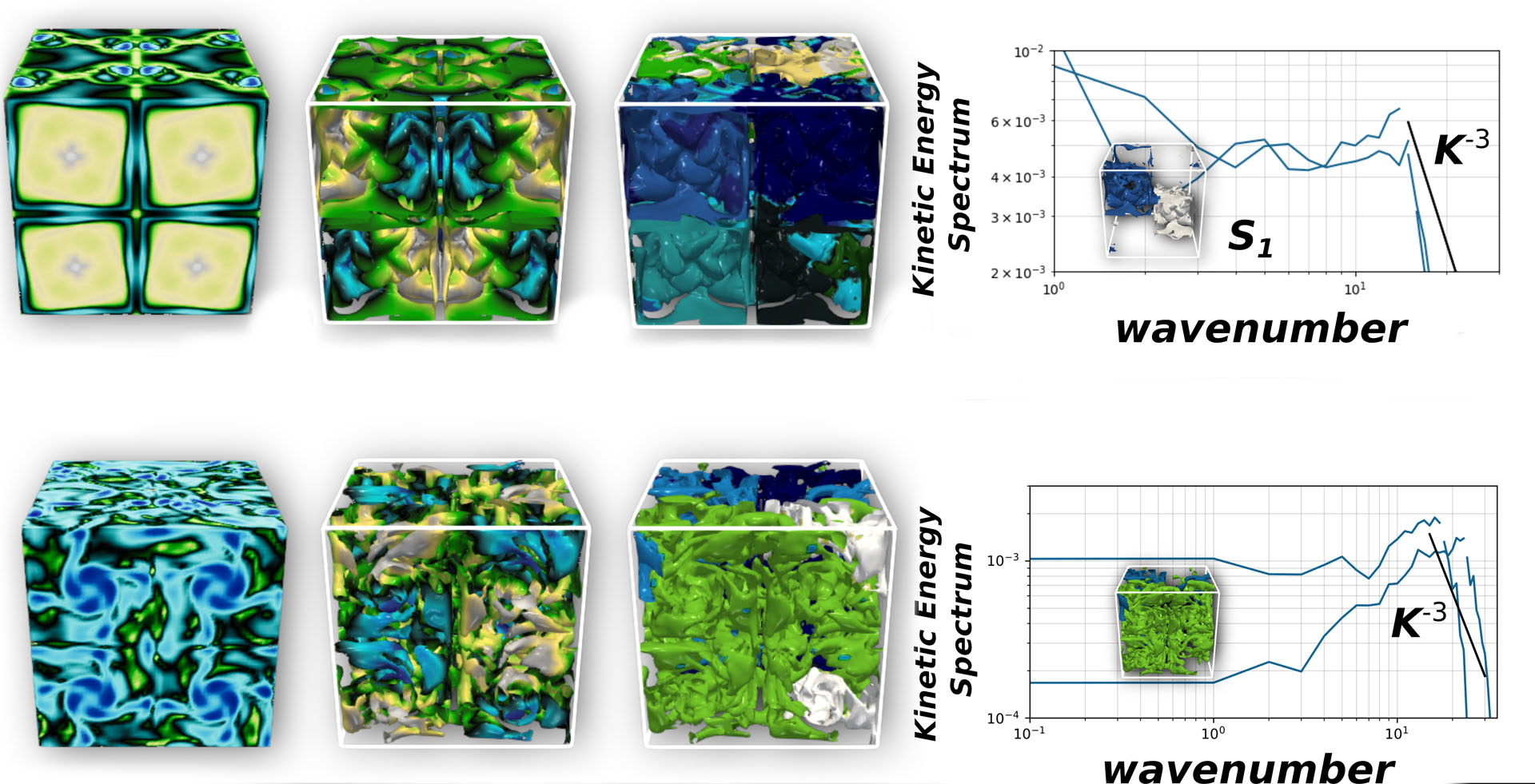
“In Situ Segmentation of Turbulent Flow With Topological Data Analysis” by Nauleau, Fovet and Vivodtzev
Conference:
Type(s):
Entry Number: 61
Title:
- In Situ Segmentation of Turbulent Flow With Topological Data Analysis
Presenter(s)/Author(s):
References:
Utkarsh Ayachit, Andrew Bauer, Berk Geveci, Patrick O’Leary, Kenneth Moreland, Nathan Fabian, and Jeffrey Mauldin. 2015. ParaView Catalyst: Enabling In Situ Data Analysis and Visualization. In Proceedings of the First Workshop on In Situ Infrastructures for Enabling Extreme-Scale Analysis and Visualization (Austin, TX, USA) (ISAV2015). Association for Computing Machinery, New York, NY, USA, 25–29. https://doi.org/10.1145/2828612.2828624Google ScholarDigital Library
Thibault Bridel-Bertomeu. 2021. Immersed boundary conditions for hypersonic flows using ENO-like least-square reconstruction. Computers & Fluids 215(2021), 104794.Google ScholarCross Ref
H. Edelsbrunner and J. Harer. 2009. Computational Topology: An Introduction. AMS.Google ScholarCross Ref
Lin Fu. 2019. A low-dissipation finite-volume method based on a new TENO shock-capturing scheme. Computer Physics Communications 235 (2019), 25–39.Google ScholarCross Ref
Yukio Kaneda, Takashi Ishihara, Mitsuo Yokokawa, Ken’ichi Itakura, and Atsuya Uno. 2003. Energy dissipation rate and energy spectrum in high resolution direct numerical simulations of turbulence in a periodic box. Physics of Fluids 15, 2 (2003), L21–L24.Google ScholarCross Ref
Keiichi Kitamura and Eiji Shima. 2013. Towards shock-stable and accurate hypersonic heating computations: A new pressure flux for AUSM-family schemes. J. Comput. Phys. 245(2013), 62–83.Google ScholarCross Ref
Meng-Sing Liou. 2006. A sequel to AUSM, Part II: AUSM+-up for all speeds. Journal of computational physics 214, 1 (2006), 137–170.Google ScholarDigital Library
Katate Masatsuka. 2013. I do Like CFD, vol. 1. Vol. 1. Lulu. com.Google Scholar
Omer San and Kursat Kara. 2015. Evaluation of Riemann flux solvers for WENO reconstruction schemes: Kelvin–Helmholtz instability. Computers & Fluids 117(2015), 24–41.Google ScholarCross Ref
Julien Tierny. 2018. Topological Data Analysis for Scientific Visualization. Springer.Google Scholar
Julien Tierny, Guillaume Favelier, Joshua A. Levine, Charles Gueunet, and Michael Michaux. 2017. The Topology ToolKit. (2017). https://topology-tool-kit.github.io/.Google Scholar