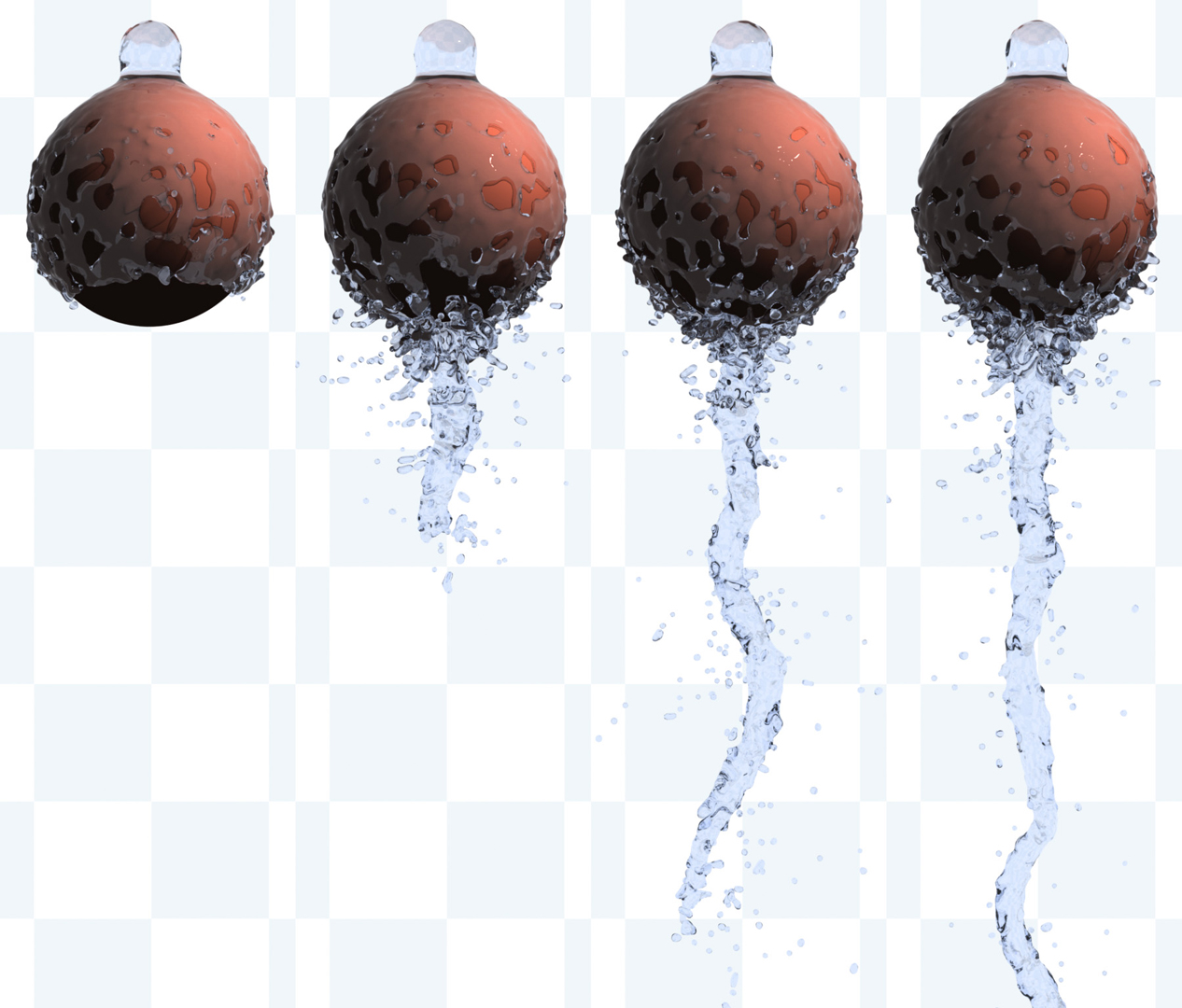“Ghost SPH for animating water” by Schechter and Bridson

We propose a new ghost fluid approach for free surface and solid boundary conditions in Smoothed Particle Hydrodynamics (SPH) liquid simulations. Prior methods either suffer from a spurious numerical surface tension artifact or drift away from the mass conservation constraint, and do not capture realistic cohesion of liquid to solids. Our Ghost SPH scheme resolves this with a new particle sampling algorithm to create a narrow layer of ghost particles in the surrounding air and solid, with careful extrapolation and treatment of fluid variables to reflect the boundary conditions. We also provide a new, simpler form of artificial viscosity based on XSPH. Examples demonstrate how the new approach captures real liquid behaviour previously unattainable by SPH with very little extra cost.

1. Adams, B., Pauly, P., Keiser, R., and Guibas, L. J. 2007. Adaptively sampled particle fluids. ACM Trans. on Graphics (Proc. SIGGRAPH) 26, 3. Google ScholarDigital Library
2. Batty, C., Bertails, F., and Bridson, R. 2007. A fast variational framework for accurate solid-fluid coupling. ACM Trans. Graph. (Proc. SIGGRAPH) 26, 3. Google ScholarDigital Library
3. Becker, M., and Teschner, M. 2007. Weakly compressible sph for free surface flows. In Proc. ACM SIGGRAPH/Eurographics SCA, 63–72. Google ScholarDigital Library
4. Becker, M., Tessendorf, H., and Teschner, M. 2009. Direct forcing for Lagrangian rigid-fluid coupling. IEEE Transactions on Visualization and Computer Graphics 15, 3, 493–503. Google ScholarDigital Library
5. Bonet, J., and Kulasegaram, S. 2002. A simplified approach to enhance the performance of smooth particle hydrodynamics methods. Appl. Math. Comput. 126, 2-3, 133–155. Google ScholarDigital Library
6. Bridson, R. 2007. Fast Poisson disk sampling in arbitrary dimensions. In ACM SIGGRAPH Technical Sketches. Google ScholarDigital Library
7. Chentanez, N., and Müller, M. 2011. A multigrid fluid pressure solver handling separating solid boundary conditions. In Proc. Symp. Comp. Anim., 83–90. Google ScholarDigital Library
8. Colagrossi, A., and Landrini, M. 2003. Numerical simulation of interfacial flows by Smoothed Particle Hydrodynamics. J. Comp. Phys. 191, 2, 448–475. Google ScholarDigital Library
9. Cook, R. L. 1986. Stochastic sampling in computer graphics. ACM Trans. Graph. 5, 1. Google ScholarDigital Library
10. Dunbar, D., and Humphreys, G. 2006. A spatial data structure for fast Poisson-disk sample generation. ACM Trans. on Graphics (Proc. SIGGRAPH) 25, 3. Google ScholarDigital Library
11. Fedkiw, R., Aslam, T., Merriman, B., and Osher, S. 1999. A non-osillatory Eulerian approach in multimaterial flows (the Ghost Fluid Method). J. Comput. Phys. 152, 457–492. Google ScholarDigital Library
12. Fedkiw, R. 2002. Coupling an Eulerian fluid calculation to a Lagrangian solid calculation with the Ghost Fluid Method. J. Comp. Phys. 175, 200–224. Google ScholarDigital Library
13. Gingold, R. A., and Monaghan, J. J. 1977. Smoothed Particle Hydrodynamics – theory and application to non-spherical stars. Mon. Not. R. Astron. Soc. 181, 375–389.Google ScholarCross Ref
14. Ihmsen, M., Akinci, N., Gissler, M., and Teschner, M. 2010. Boundary handling and adaptive time-stepping for PCISPH. In Proc. VRIPHYS, 79–88.Google Scholar
15. Keiser, R., Adams, B., Guibas, L. J., Dutré, P., and Pauly, M. 2006. Multiresolution particle-based fluids. Tech. Rep. 520.Google Scholar
16. Lucy, L. B. 1977. A numerical approach to the testing of the fission hypothesis. Astron. J 82, 1013–1024.Google ScholarCross Ref
17. Monaghan, J. J. 1989. On the problem of penetration in particle methods. J. Comput. Phys. 82, 1–15. Google ScholarDigital Library
18. Monaghan, J. J. 1994. Simulating free surface flows with SPH. J. Comput. Phys. 110, 399–406. Google ScholarDigital Library
19. Monaghan, J. J. 2005. Smoothed Particle Hydrodynamics. Reports on Progress in Physics 68, 8, 1703–1759.Google ScholarCross Ref
20. Müller, M., Charypar, D., and Gross, M. 2003. Particle-based fluid simulation for interactive applications. In Proc. ACM SIGGRAPH/Eurographics SCA, 154–159. Google ScholarDigital Library
21. Müller, M., Solenthaler, B., and Keiser, R. 2005. Particle-based fluid-fluid interaction. In Proc. ACM SIGGRAPH/Eurographics SCA, 237–244. Google ScholarDigital Library
22. Solenthaler, B., and Gross, M. 2011. Two-scale particle simulation. ACM Trans. on Graphics (Proc. SIGGRAPH) 30, 4, 81:1–81:8. Google ScholarDigital Library
23. Solenthaler, B., and Pajarola, R. 2008. Density contrast SPH interfaces. In Proc. ACM SIGGRAPH/Eurographics SCA, 211–218. Google ScholarDigital Library
24. Solenthaler, B., and Pajarola, R. 2009. Predictive-corrective incompressible SPH. ACM Trans. on Graphics (Proc. SIGGRAPH) 28, 3. Google ScholarDigital Library
25. Turk, G. 1992. Re-tiling polygonal surfaces. ACM Trans. on Graphics (Proc. SIGGRAPH) 26, 2. Google ScholarDigital Library