“Galilean Invariance for Fluid Simulation” by Shah, Pighin and Cohen
Conference:
Type(s):
Title:
- Galilean Invariance for Fluid Simulation
Presenter(s)/Author(s):
Abstract:
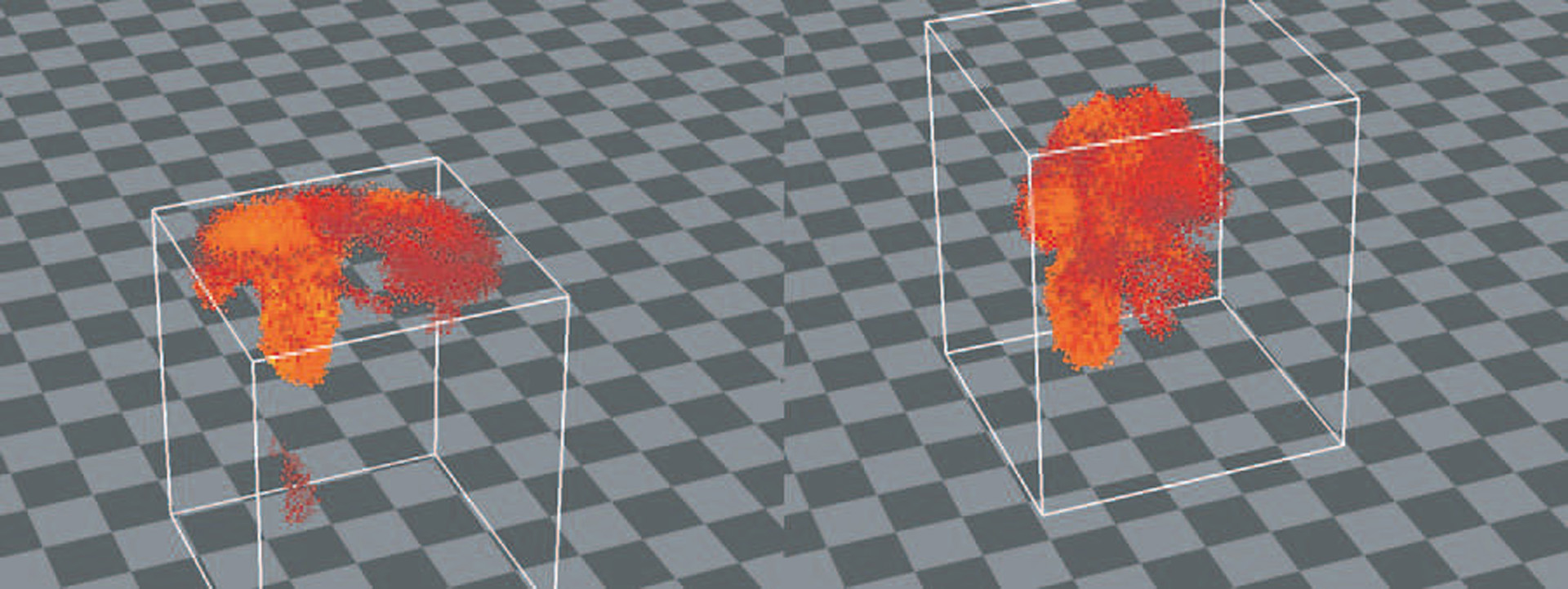
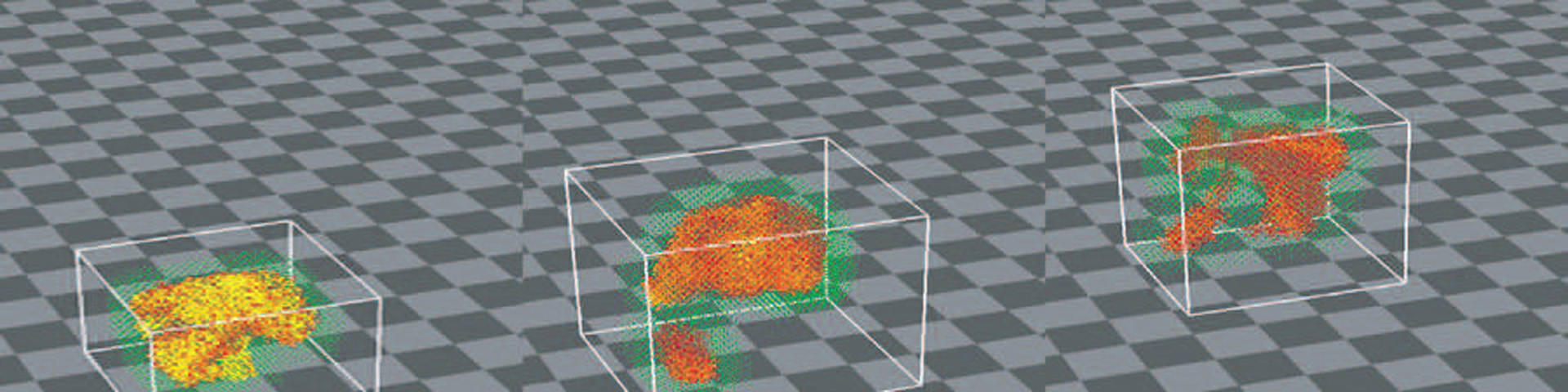
These partial differential equations are solved on a grid of voxels. This grid is a discrete representation of three-space. Unfortunately fluid simulations are notoriously difficult to predict. In particular slight variations in the initial conditions can have dramatic impact in turbulent flows. This makes the problem of deciding a grid size for the simulation extremely difficult. With open boundaries, the fluid quickly dissipates when it hits the boundaries of the simulation domain (as in figure 1 (a)). To prevent this, large simulation domains have to be used to keep the fluid away from the boundaries of the simulation. In this work, we describe a novel technique for implementing an adaptive grid for fluid simulation. The technique is largely based on the principle of Galilean Invariance.
References:
Stam, J. 1999. Stable fluids. In Proceedings of SIGGRAPH 99, 181–188.