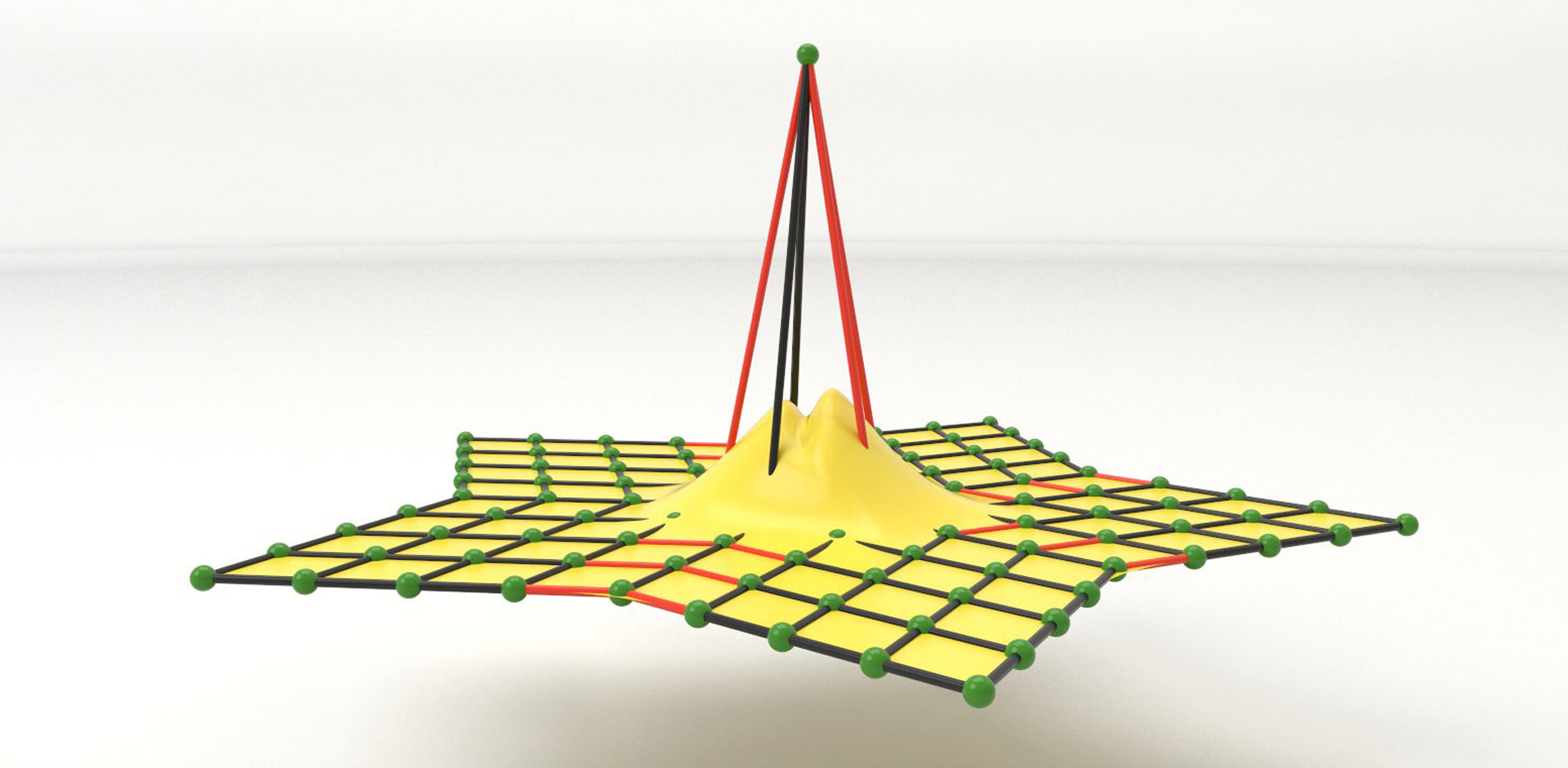
“G1 non-uniform Catmull-Clark surfaces”

  • ©Xin Li, G Thomas Finnigan, and Thomas (Tom) W. Sederberg

Conference:


Type:


Title:

    G1 non-uniform Catmull-Clark surfaces

Session/Category Title: MESHES & FIELDS

Presenter(s)/Author(s):


Moderator(s):


Abstract:


    This paper develops new refinement rules for non-uniform Catmull-Clark surfaces that produce G1 extraordinary points whose blending functions have a single local maximum. The method consists of designing an “eigen polyhedron” in R2 for each extraordinary point, and formulating refinement rules for which refinement of the eigen polyhedron reduces to a scale and translation. These refinement rules, when applied to a non-uniform Catmull-Clark control mesh in R3, yield a G1 extraordinary point.

References:


    1. Ball, A. A., and Storry, D. J. 1988. Conditions for tangent plane continuity over recursively generated b-spline surfaces. ACM Transactions on Graphics (TOG) 7, 2, 83–102. Google ScholarDigital Library
    2. Bazilevs, Y., Calo, V. M., Cottrell, J. A., Evans, J. A., Hughes, T. J. R., Lipton, S., Scott, M. A., and Sederberg, T. W. 2010. Isogeometric analysis using T-splines. Computer Methods in Applied Mechanics and Engineering 199, 5-8, 229–263.Google ScholarCross Ref
    3. Cashman, T. J., Augsdörfer, U. H., Dodgson, N. A., and Sabin, M. A. 2009. NURBS with Extraordinary Points: High-degree, Non-uniform, Rational Subdivision Schemes. ACM Transactions on Graphics 28, 3, 1–9. Google ScholarDigital Library
    4. Cashman, T. J. 2010. NURBS-compatible subdivision surfaces. Tech. rep., University of Cambridge.Google Scholar
    5. Floater, M. S. 2015. The inverse of a rational bilinear mapping. Computer Aided Geometric Design 33, 46–50. Google ScholarDigital Library
    6. Kovacs, D., Bisceglio, J., and Zorin, D. 2015. Dyadic T-mesh subdivision. ACM Transactions on Graphics (TOG) 34, 4, 143. Google ScholarDigital Library
    7. Müller, K., Reusche, L., and Fellner, D. 2006. Extended subdivision surfaces: Building a bridge between NURBS and Catmull-Clark surfaces. ACM Transactions on Graphics (TOG) 25, 2, 268–292. Google ScholarDigital Library
    8. Müller, K., Fünfzig, C., Reusche, L., Hansford, D., Farin, G., and Hagen, H. 2010. Dinus: Double insertion, nonuniform, stationary subdivision surfaces. ACM Transactions on Graphics (TOG) 29, 3, 1–21. Google ScholarDigital Library
    9. Peters, J., and Reif, U. 2008. Subdivision surfaces. Springer. Google ScholarDigital Library
    10. Peters, J. 2000. Patching Catmull-Clark meshes. In Proceedings of the 27th annual conference on Computer graphics and interactive techniques, ACM Press/Addison-Wesley Publishing Co., 255–258. Google ScholarDigital Library
    11. Reif, U. 1995. A unified approach to subdivision algorithms near extraordinary vertices. Computer Aided Geometry Design 12, 153–174. Google ScholarDigital Library
    12. Sederberg, T. W., Zheng, J., Sewell, D., and Sabin, M. 1998. Non-uniform recursive subdivision surfaces. In Proceedings of the 25th Annual Conference on Computer Graphics and Interactive Techniques, ACM, New York, NY, USA, SIGGRAPH ’98, ACM, 387–394. Google ScholarDigital Library
    13. Sederberg, T. W., Zheng, J., Bakenov, A., and Nasri, A. 2003. T-splines and T-NURCCSs. ACM Transactions on Graphics 22 (3), 477–484. Google ScholarDigital Library
    14. Sederberg, T. W., Zheng, J., and Song, X. 2003. Knot intervals and multi-degree splines. Computer Aided Geometric Design 20, 7, 455–468. Google ScholarDigital Library
    15. T-Splines for Rhino3D Plugin Developers, 2016. Implementation of extraordinary points. Private conversation.Google Scholar

ACM Digital Library Publication:


Overview Page: