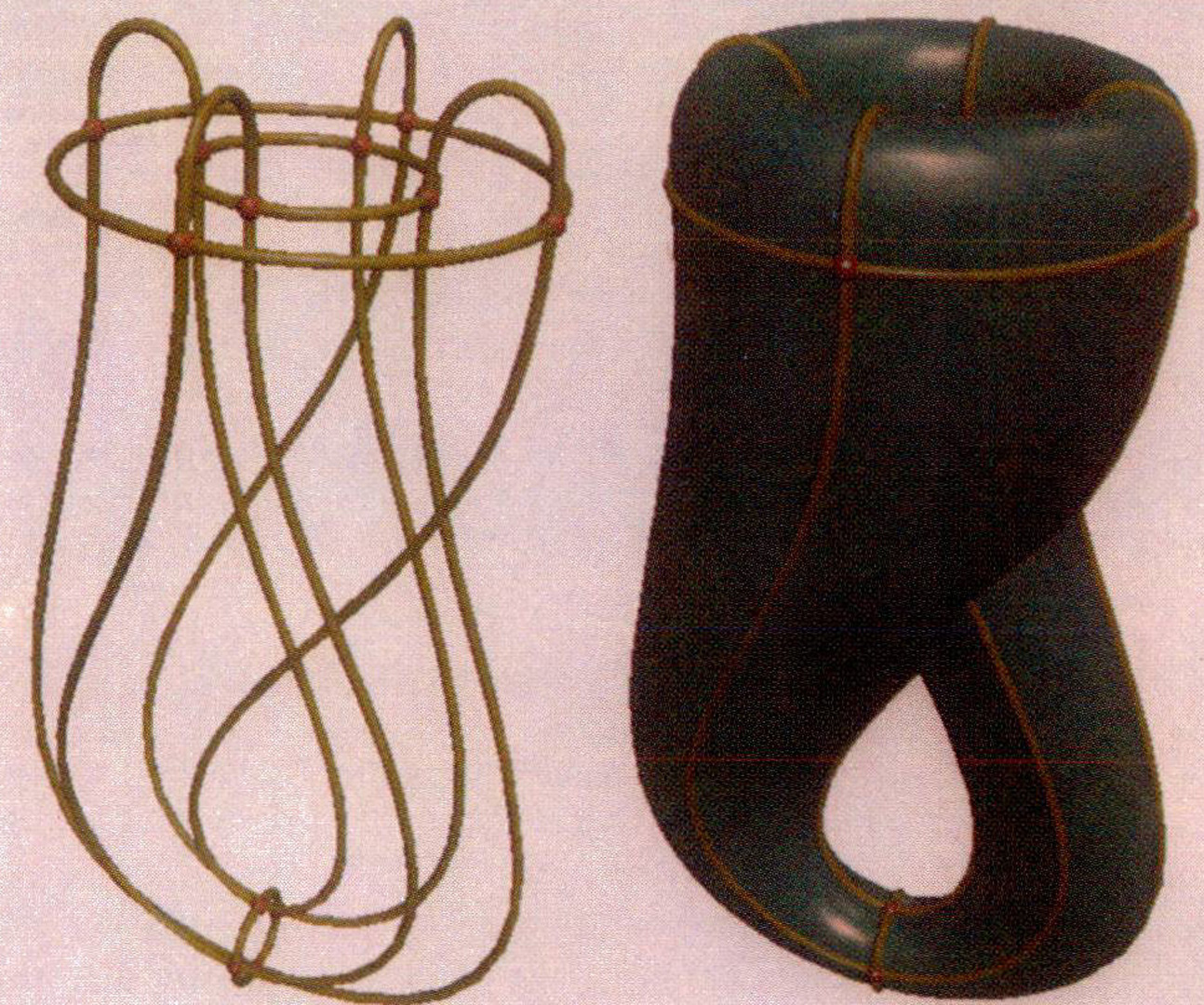
“Functional optimization for fair surface design” by Moreton and Séquin
Conference:
Type(s):
Title:
- Functional optimization for fair surface design
Presenter(s)/Author(s):
Abstract:
No abstract available.
References:
1. Abramowitz, M. and Stegun, I.A. (editors), Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, Dover, Inc., New York, N.Y. 1972.
2. Birkhoff, G.D., Aesthetic Measure, Harvard University, Cambridge, Mass., 1933.
3. Blinn, J.E, Newell, M.E., Texture and Reflection in Computer Generated Images, Communications of the ACM 19, 10 (Oct. 1976), 542-547.
4. BOhm, W., On Cyclides in Geometric Modeling, Computer Aided Geometric Design 7 (1990), 243-255.
5. Calladine, C.R., Gaussian Curvature and Shell Structures. In The Mathematics of Surfaces, Clarendon, Oxford, England, 1986, pp. 179-196.
6. Celniker, G. and Gossard, D., Deformable Curve and Surface Finite-Elements for Free-Form Shape Design, Proceedings of SIGGRAPH ’91 (Las Vegas, Nevada, July 29-August 2, 1991t. In Computer Graphics 25, 4 (July 1991 ), 257-266.
7. Conte, S.D. and de Boor, C. Elementary Numerical Analysis, An Algorithmic Approach, McGraw-Hill, New York, N.Y., 1980.
8. Courant, R. and Hilbert, D., Methods of Mathematical Physics, Volume I, Wiley-lnterScience, New York, N.Y., 1953.
9. DeRose, T.D. and Mann, S., An Approximately G I Cubic Surface Interpolation. To appear in the Proceedings of the 1991 Biri Conference.
10. DeRose, T.D., Necessary and Sufficient Conditions for Tangent Plane Continuity of B~zier Surfaces, Computer Aided Geometric Design 7 (1990), 165-179.
11. Do Carmo, M.P., Differential Geometry of Curves and Surfaces, Prentice-Hall, Englewood Cliffs, New Jersey, 1976.
12. Greene, N., Environment Mapping and Other Applications of World Projections, IEEE Computer Graphics and Applications 6, 11 (Nov. 1986), 21-29.
13. Hagen, H. and Schulze, G., Automatic Smoothing with Geometric Surface Patches, Computer Aided Geometric Design 4, (1987), 231-236.
14. Jones, A.K., Nonrectangular Surface Patches with Curvature Continuity, Computer Aided Design 20, 6 (Jul./Aug. 1988), 325-335.
15. Kallay, M. and Ravani, B., Optimal Twist Vectors as a Tool for Interpolating a Network of Curves with a Minimum Energy Surface, Computer Aided Geometric Design 7, 6 (1990), 465-473.
16. Lott, N.J. and Pullin, D.L., Method for Fairing B-spline Surfaces, Computer Aided Design 20, 10 (Dec. 1988), 597- 604.
17. Mann, S., Loop, C., Lounsbery, M., Meyers, D., Painter, J., DeRose, T., and Sloan, K., A Survey of Parametric Scattered Data Fitting Using Triangular Interpolants. In Curve and Surface Modeling, Hagen, H. (editor), SIAM.
18. Mehlum, E., Nonlinear Splines. In Computer Aided Geometric Design, R.E. Bamhill and R.E Riesenfeld (editors), Academic, Orlando, Florida, 1974, pp. 173-207.
19. Moreton, H.P. and S~quin, C.H., Surface Design with Minimum Energy Networks. In Proceeding of the Symposium on Solid Modeling Foundations and CAD~CAM Applications (Austin, Tex., June 5-7). ACM, New York, N.Y., 1991, pp. 291-301.
20. Nielson, G.M., A Method for Interpolating Scattered Data Based Upon a Minimum Norm Network, Mathematics of Computation 40, 161 (1983), 253-271.
21. Nowacki, H. and Reese, D., Design and Fairing of Ship Surfaces. In Surfaces in Computer Aided Geometric Design, Barnhill, R,E. and Boehm, W. (editors), North-Holland, Amsterdam, The Netherlands 1983, pp. 121-134.
22. Peters, J., Local Smooth Surface Interpolation: A Classification, Computer Aided Geometric Design 7 (1990), 191-195.
23. Peters, J., Smooth Interpolation of a Mesh of Curves, Constructive Approximation 7 (1991 ), 221-247.
24. Poeschl, T., Detecting Surface Irregularities Using Isophotes, Computer Aided Geometric Design, 1 (1984), 163-168.
25. Pottmann, H., Scattered Data Interpolation Based upon Generalized Minimum Norm Networks, Preprint Nr. 1232, Technische Hochschule, Darmstadt, May 1989.
26. Pramila, A., Ship Hull Surface Using Finite Elements, International Shipbuilding Progress 25, 284 (1978), 97-107.
27. Pratt, M.J., Cyclides in Computer Aided Geometric Design, Computer Aided Geometric Design 7 (1990), 221-242.
28. Rando, T. and Roulier, J.A., Designing Faired Parametric Surfaces, Computer Aided Design 23, 7 (Sept. 1991), 492- 497.
29. Sarraga, R.E, G1 Interpolation of Generally Unrestricted Cubic B~zier Curves, Computer Aided Geometric Design 6, 2 (1987), 23-40.
30. Shirman, L. and S6quin, C.H., Local Surface Interpolation with B6zier Patches, Computer Aided Geometric Design 4 (1987), 279-295.
31. Todd P.H. and McLeod, R.J.Y., Numerical Estimation of the Curvature of Surfaces, Computer Aided Design 18, 1 (Jan./ Feb. 1986),-33-37.
32. Williams, C.J.K., Use of Structural Analogy in Generation of Smooth Surfaces for Engineering Purposes, Computer Aided Design 19, 6 (Jul./Aug. 1987), 310-322.
33. Wolfram, S., Mathematica, A System for Doing Mathematics by Computer, 2nd ed., Addison-Wesley, Redwood City, California, 1991.
34. Zienkiewicz, O.C., The Finite Element Method, McGraw-Hill, London, England, 1977.