“Freeform surfaces from single curved panels” by Pottmann, Schiftner, Bo, Schmiedhofer, Wang, et al. …
Conference:
Type(s):
Title:
- Freeform surfaces from single curved panels
Presenter(s)/Author(s):
Abstract:
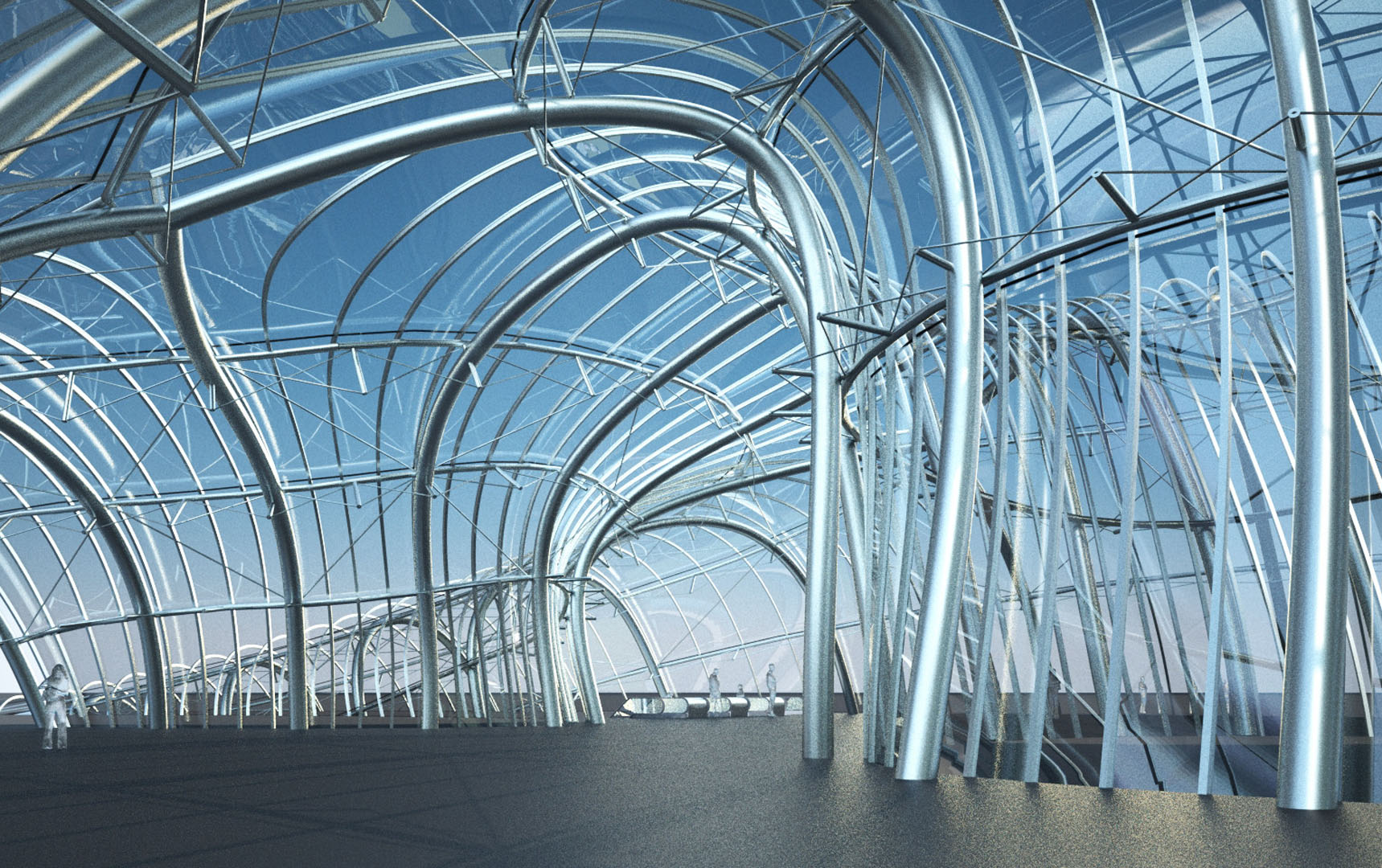
Motivated by applications in architecture and manufacturing, we discuss the problem of covering a freeform surface by single curved panels. This leads to the new concept of semi-discrete surface representation, which constitutes a link between smooth and discrete surfaces. The basic entity we are working with is the developable strip model. It is the semi-discrete equivalent of a quad mesh with planar faces, or a conjugate parametrization of a smooth surface. We present a B-spline based optimization framework for efficient computing with D-strip models. In particular we study conical and circular models, which semi-discretize the network of principal curvature lines, and which enjoy elegant geometric properties. Together with geodesic models and cylindrical models they offer a rich source of solutions for surface panelization problems.
References:
1. Aumann, G. 2004. Degree elevation and developable Bézier surfaces. Computer Aided Geom. Design 21, 661–670. Google ScholarDigital Library
2. Bobenko, A., and Suris, Yu. 2005. Discrete differential geometry. Consistency as integrability. arXiv math. DG/0504358.Google Scholar
3. Cerda, E., Chaieb, S., Melo, F., and Mahadevan, L. 1999. Conical dislocations in crumpling. Nature 401, 46–49.Google ScholarCross Ref
4. Chu, C. H., and Séquin, C. 2002. Developable Bézier patches: properties and design. Comp.-Aided Design 34, 511–528.Google ScholarCross Ref
5. Do Carmo, M. 1976. Differential Geometry of Curves and Surfaces. Prentice-Hall.Google Scholar
6. Frey, W. 2004. Modeling buckled developable surfaces by triangulation. Comp.-Aided Design 36, 4, 299–313.Google ScholarCross Ref
7. Huhnen-Venedey, E. 2007. Curvature line parametrized surfaces and orthogonal coordinate systems. Discretization with Dupin cyclides. Master’s thesis, TU Berlin.Google Scholar
8. Julius, D., Kraevoy, V., and Sheffer, A. 2005. D-charts: Quasi-developable mesh segmentation. Computer Graphics Forum 24, 3, 581–590. Proc. Eurographics.Google ScholarCross Ref
9. Kälberer, F., Nieser, M., and Polthier, K. 2007. Quad-Cover – surface parameterization using branched coverings. Computer Graphics Forum 26, 3, 375–384. Proc. Eurographics.Google ScholarCross Ref
10. Kelley, C. T. 1999. Iterative Methods for Optimization. SIAM.Google Scholar
11. Liu, Y., Pottmann, H., Wallner, J., Yang, Y.-L., and Wang, W. 2006. Geometric modeling with conical meshes and developable surfaces. ACM Trans. Graphics 25, 3, 681–689. Google ScholarDigital Library
12. Martin, R. R., De Pont, J., and Sharrock, T. J. 1986. Cyclide surfaces in computer aided design. In The mathematics of surfaces, J. A. Gregory, Ed. Clarendon Press, Oxford, 253–268.Google Scholar
13. Massarwi, F., Gotsman, C., and Elber, G. 2007. Papercraft models using generalized cylinders. In Pacific Graph., 148–157. Google ScholarDigital Library
14. Mitani, J., and Suzuki, H. 2004. Making papercraft toys from meshes using strip-based approximate unfolding. ACM Trans. Graphics 23, 3, 259–263. Google ScholarDigital Library
15. Pérez, F., and Suárez, J. A. 2007. Quasi-developable B-spline surfaces in ship hull design. Comp.-Aided Design 39, 853–862.Google ScholarCross Ref
16. Pottmann, H., and Peternell, M. 1998. Applications of Laguerre geometry in CAGD. Computer Aided Geom. Design 15, 165–186. Google ScholarDigital Library
17. Pottmann, H., and Wallner, J. 2001. Computational Line Geometry. Springer. Google ScholarDigital Library
18. Pottmann, H., and Wallner, J. 2007. The focal geometry of circular and conical meshes. Adv. Comp. Math. to appear.Google Scholar
19. Pottmann, H., Huang, Q.-X., Yang, Y.-L., and Hu, S.-M. 2006. Geometry and convergence analysis of algorithms for registration of 3D shapes. Int. J. Computer Vision 67, 3, 277–296. Google ScholarDigital Library
20. Pottmann, H., Asperl, A., Hofer, M., and Kilian, A. 2007. Architectural Geometry. Bentley Institute Press.Google Scholar
21. Pottmann, H., Liu, Y., Wallner, J., Bobenko, A., and Wang, W. 2007. Geometry of multi-layer freeform structures for architecture. ACM Trans. Graphics 26, 3, #65, 1–11. Google ScholarDigital Library
22. Rose, K., Sheffer, A., Wither, J., Cani, M., and Thibert, B. 2007. Developable surfaces from arbitrary sketched boundaries. In Symp. Geom. Processing, A. Belyaev and M. Garland, Eds. Eurographics, 163–172. Google ScholarDigital Library
23. Sauer, R. 1970. Differenzengeometrie. Springer.Google Scholar
24. Shatz, I., Tal, A., and Leifman, G. 2006. Papercraft models from meshes. Vis. Computer 22, 825–834. Google ScholarDigital Library
25. Shelden, D. 2002. Digital surface representation and the constructibility of Gehry’s architecture. PhD thesis, M.I.T.Google Scholar
26. Spuybroek, L. 2004. NOX: Machining Architecture. Thames & Hudson.Google Scholar
27. Subag, J., and Elber, G. 2006. Piecewise developable surface approximation of general NURBS surfaces with global error bounds. In GMP 2006, vol. 4077 of LNCS. Springer, 143–156. Google ScholarDigital Library
28. Toledo, S., 2003. TAUCS, a library of sparse linear solvers. C library, http://www.tau.ac.il/~stoledo/taucs/.Google Scholar
29. Wang, C., and Tang, K. 2004. Achieving developability of a polygonal surface by minimum deformation: a study of global and local optimization approaches. Vis. Computer 20, 521–539. Google ScholarDigital Library
30. Yamauchi, H., Gumhold, S., Zayer, R., and Seidel, H. P. 2005. Mesh segmentation driven by Gaussian curvature. Vis. Computer 21, 659–668.Google ScholarCross Ref
31. Yu, J., Yin, X., Gu, X., McMillan, L., and Gortler, S. J. 2007. Focal surfaces of discrete geometry. In Symp. Geom. Processing, A. Belyaev and M. Garland, Eds. Eurographics, 23–32. Google ScholarDigital Library