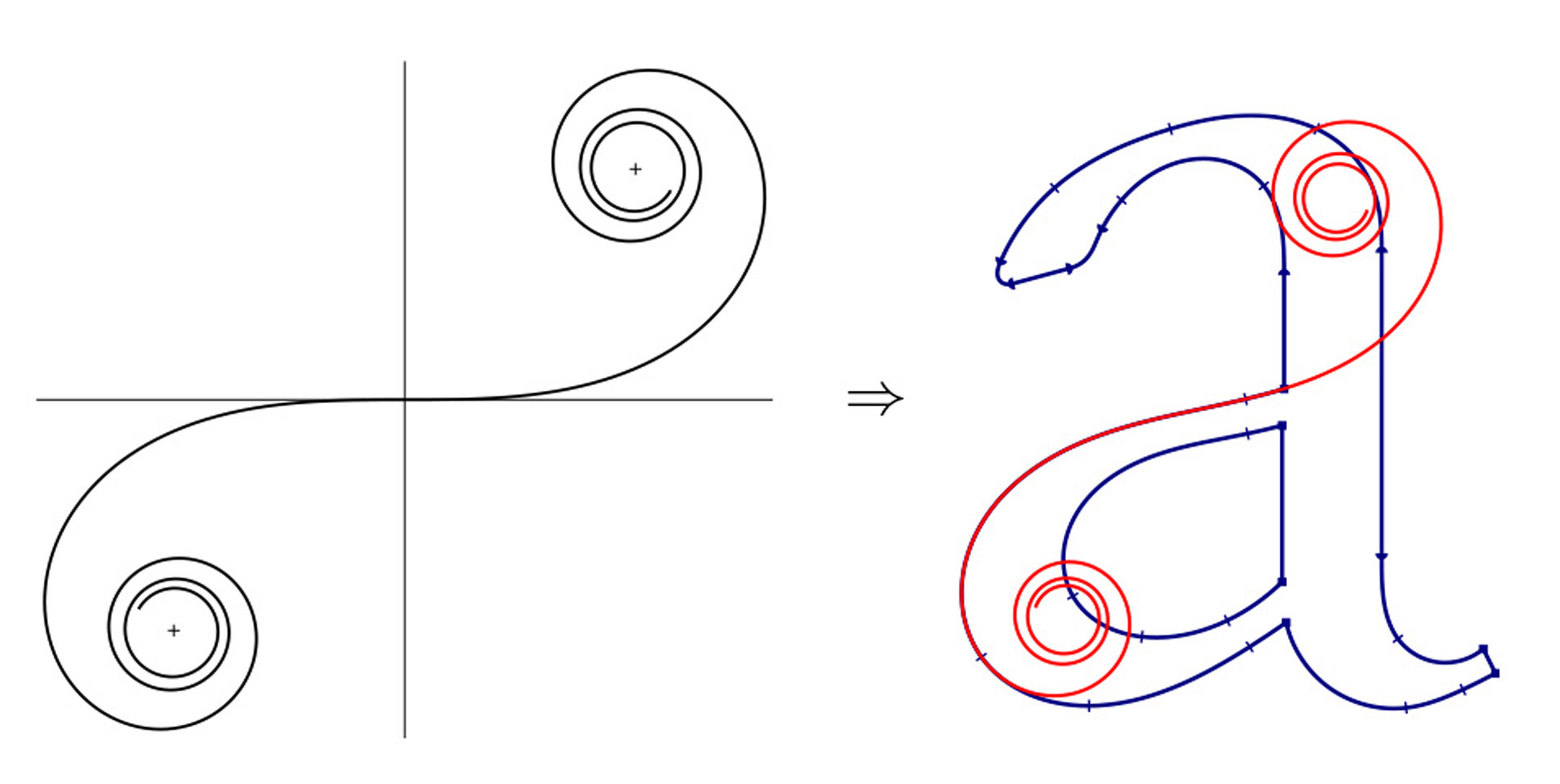
“Eureka: Euler spiral splines” by Levien and Séquin
Conference:
Type(s):
Title:
- Eureka: Euler spiral splines
Presenter(s)/Author(s):
Abstract:
For many applications, one would like to define a complex curve with just a few points so the resulting curve smoothly passes through all the points given. Interpolating splines are the most intuitive user interface for designing curves such as font outlines. Another application is to draw smooth roadways on maps given a sequence of point samples along their length. Given a sequence of points, if a curve is ideal with regard to some metric, then it will not change if another point is added along the curve. If they differ, then one or the other cannot have been ideal. A spline that is invariant with respect to the addition of on-curve points is considered to be extensible. All curves minimizing the value of some functional are extensible.