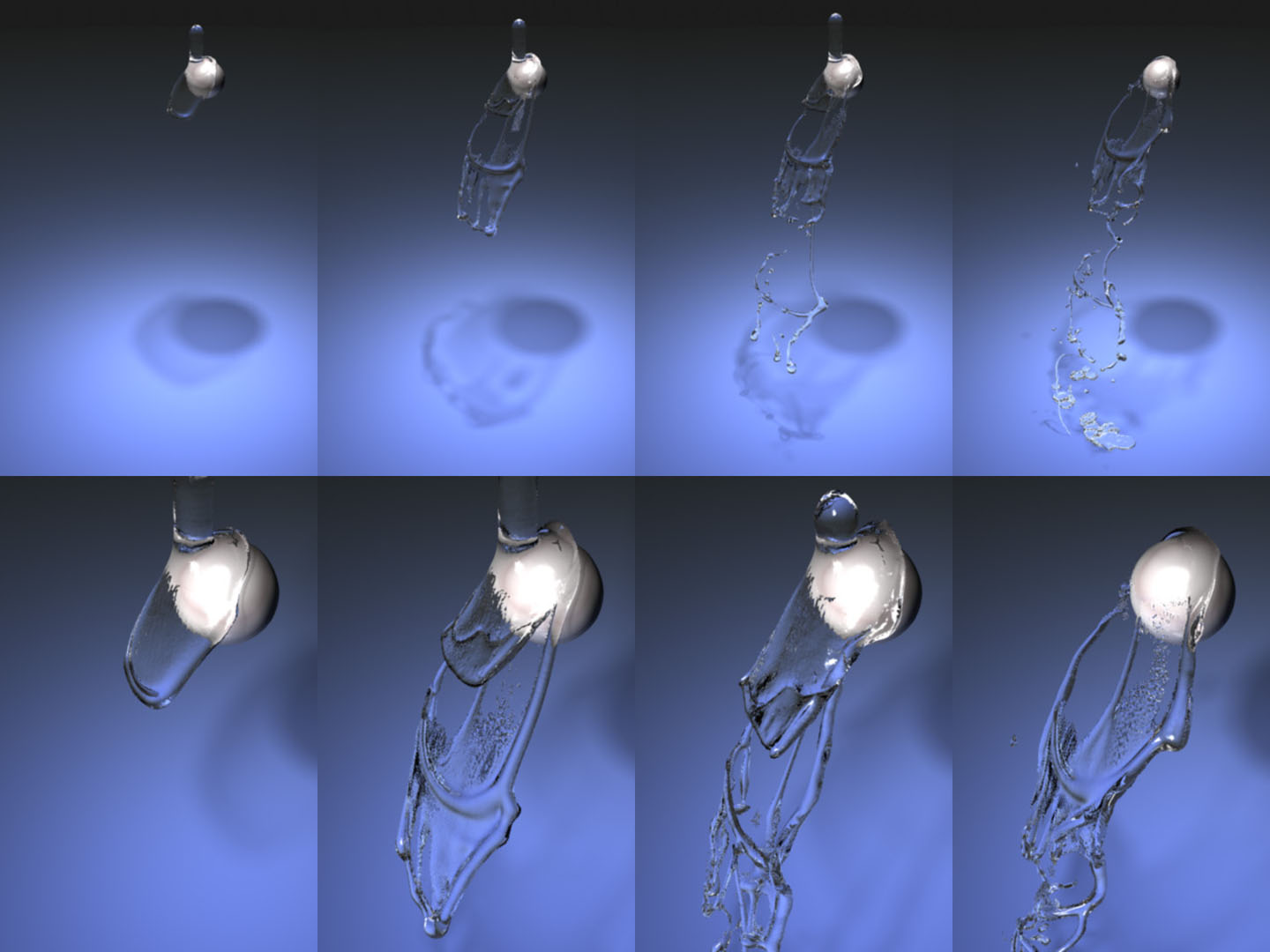
“Discontinuous fluids” by Hong and Kim
Conference:
Type(s):
Title:
- Discontinuous fluids
Presenter(s)/Author(s):
Abstract:
At interfaces between different fluids, properties such as density, viscosity, and molecular cohesion are discontinuous. To animate small-scale details of incompressible viscous multi-phase fluids realistically, we focus on the discontinuities in the state variables that express these properties. Surface tension of both free and bubble surfaces is modeled using the jump condition in the pressure field; and discontinuities in the velocity gradient field. driven by viscosity differences, are also considered. To obtain derivatives of the pressure and velocity fields with sub-grid accuracy, they are extrapolated across interfaces using continuous variables based on physical properties. The numerical methods that we present are easy to implement and do not impact the performance of existing solvers. Small-scale fluid motions, such as capillary instability, breakup of liquid sheets, and bubbly water can all be successfully animated.
References:
1. Brackbill, J. U., Kothe, D. B., and Zemach, C. 1992. A continuum method for modeling surface tension. Journal of Computational Physics, 335–354. Google ScholarDigital Library
2. Carlson, M., Mucha, P., Horn, B. V., and Turk, G. 2002. Melting and flowing. In ACM SIGGRAPH Symposium on Computer Animation, 167–174. Google ScholarDigital Library
3. Chorin, A. J. 1967. A numerical method for solving incompressible viscous flow problems. Journal of Computational Physics 2, 12–16.Google ScholarCross Ref
4. De Sousa, F., Mangiavacchi, N., Nonato, L., Castelo, A., Tome, M., Ferreira, V., Cuminato, J., and McKee, S. 2004. A front-tracking/front-capturing method for the simulation of 3d multi-fluid flows with free-surfaces. Journal of Computational Physics 198, 469–499. Google ScholarDigital Library
5. Dyke, M. V. 1982. An Album of Fluid Motion. The Parabolic Press.Google Scholar
6. Enright, D., Marschner, S., and Fedkiw, R. 2002. Animation and rendering of complex water surfaces. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2002) 21, 3, 736–744. Google ScholarDigital Library
7. Enright, D., Losasso. F., and Fedkiw, R. 2005. A fast and accurate semi-lagrangian particle level set method. Computers and Structures, 83, 479–490. Google ScholarDigital Library
8. Fattal, R., and Lischinski, D. 2004. Target-driven smoke animation. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2004) 23, 3. Google ScholarDigital Library
9. Fedkiw, R., Aslam, T., Merriman, B., and Osher, S. 1999. A non-oscillatory eulerian approach to interfaces in multimaterial flows (the ghost fluid method). Journal of Computational Physics 152, 457–492. Google ScholarDigital Library
10. Fedkiw, R., Stam, J., and Jensen, H. W. 2001. Visual simulation of smoke. In Proceedings of SIGGRAPH 2001, 15–22. Google ScholarDigital Library
11. Foster, N., and Fedkiw, R. 2001. Practical animation of liquids. In Proceedings of ACM SIGGRAPH 2001, 23–30. Google ScholarDigital Library
12. Foster, N., and Metaxas, D. 1996. Realistic animation of liquids. Graphical Models and Image Processing 58, 5, 471–483. Google ScholarDigital Library
13. Goktekin, T. G., Bargteil, A. W., and O’Brien, J. F. 2004. A method for animating viscoelastic fluids. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2004) 23, 3. Google ScholarDigital Library
14. Greenwood, S., and House, D. 2004. Better with bubbles: Enhancing the visual realism of simulated fluid. In ACM SIGGRAPH/Eurographics symposium on Computer animation. Google ScholarDigital Library
15. Harlow, F. H., and Welch, J. E. 1965. Numerical calculation of time-dependent viscous incompressible flow of fluid with free surfaces. Physics of Fluids 8, 2182–2189.Google ScholarCross Ref
16. Hong, J.-M., and Kim, C.-H. 2003. Animation of bubbles in liquid. Computer Graphics Forum (Eurographics 2003 Proceedings) 22, 3, 253–262.Google Scholar
17. Hong, J.-M., and Kim, C.-H. 2004. Controlling fluid animation with geometric potential. Computer Animation and Virtual Worlds 15, 147–157. Google ScholarDigital Library
18. Kang, M., Fedkiw, R. P., and Liu, X.-D. 2000. A boundary condition capturing method for multiphase incompressible flow. Journal of Scientific Computing 15, 323–360. Google ScholarDigital Library
19. Liu, X.-D., Fedkiw, R. P., and Kang, M.-J. 2000. A boundary condition capturing method for poisson’s equation on irregular domain. Journal of Computational Physics 172, 71–98. Google ScholarDigital Library
20. Losasso, F., Fedkiw, R., and Osher, S. 2004. Spatially adaptive techniques for level set methods and incompressible flow. Computers and FluidsGoogle Scholar
21. Losasso, F., Gibou, F., and Fedkiw, R. 2004. Simulating water and smoke with an octree data structure. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2004) 23, 3. Google ScholarDigital Library
22. McNamara, A., Treuille, A., Popovic, Z., and Stam, J. 2004. Fluid control using the adjoint method. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2004) 23, 3. Google ScholarDigital Library
23. Nguyen, D., Fedkiw, R., and Jensen, H. 2002. Physically based modeling and animation of fire. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2002) 21, 3, 721–728. Google ScholarDigital Library
24. Osher, S., and Fedkiw, R. 2002. Level Set Methods and Dynamic Implicit Surfaces. Springer-Verlag.Google Scholar
25. Rasmussen, N., Enright, D., Nguyen, D., Marino, S., Sumner, N., Geiger, W., Hoon, S., and Fedkiw, R. 2004. Directable photorealistic liquids. In ACM SIGGRAPH Symposium on Computer Animation, 193–202. Google ScholarDigital Library
26. Saad, Y. 1996. Iterative Methods for Sparse Linear Systems. PWS Publishing. Google ScholarDigital Library
27. Song, O.-Y., Shin, H.-C., and Ko, H.-S. 2005. Stable but non-dissipative water. ACM Transactions on Graphics 24, 1. Google ScholarDigital Library
28. Stam, J. 1999. Stable fluids. In Proceedings of ACM SIGGRAPH 1999, 121–128. Google ScholarDigital Library
29. Sussman, M. 2003. A second order coupled level set and volume-of-fluid method for computing growth and collapse of vapor bubbles. Journal of Computational Physics 187, 110–136. Google ScholarDigital Library
30. Takahashi, T., Fujii, H., Kunimatsu, A., Hiwada, K., Saito, T., Tanaka, K., and Ueki, H. 2003. Realistic animation of fluid with splash and foam. Computer Graphics Forum (In Eurographics 2003 Proceedings) 22, 3, 391–400.Google Scholar
31. Treuille, A., McNamara, A., Popović, Z., and Stam, J. 2003. Keyframe control of smoke simulations. ACM Transactions on Graphics (Proceedings of ACM SIGGRAPH 2003) 22, 3, 716–723. Google ScholarDigital Library
32. Tryggvason, G., Bunner, B., Esmaeeli, A., Juric, D., Alrawahi, N., Tauber, W., Han, J., Nas, S., and Jan, Y.-J. 2001. A front-tracking method for the computations of multi-phase flow. Journal of Computational Physics 169, 708–759. Google ScholarDigital Library