“Dip transform for 3D shape reconstruction” by Aberman, Katzir, Zhou, Luo, Sharf, et al. …
Conference:
Type(s):
Title:
- Dip transform for 3D shape reconstruction
Session/Category Title: Reconstructing 3D Surfaces From Points, Lines, Images & Water
Presenter(s)/Author(s):
Moderator(s):
Abstract:
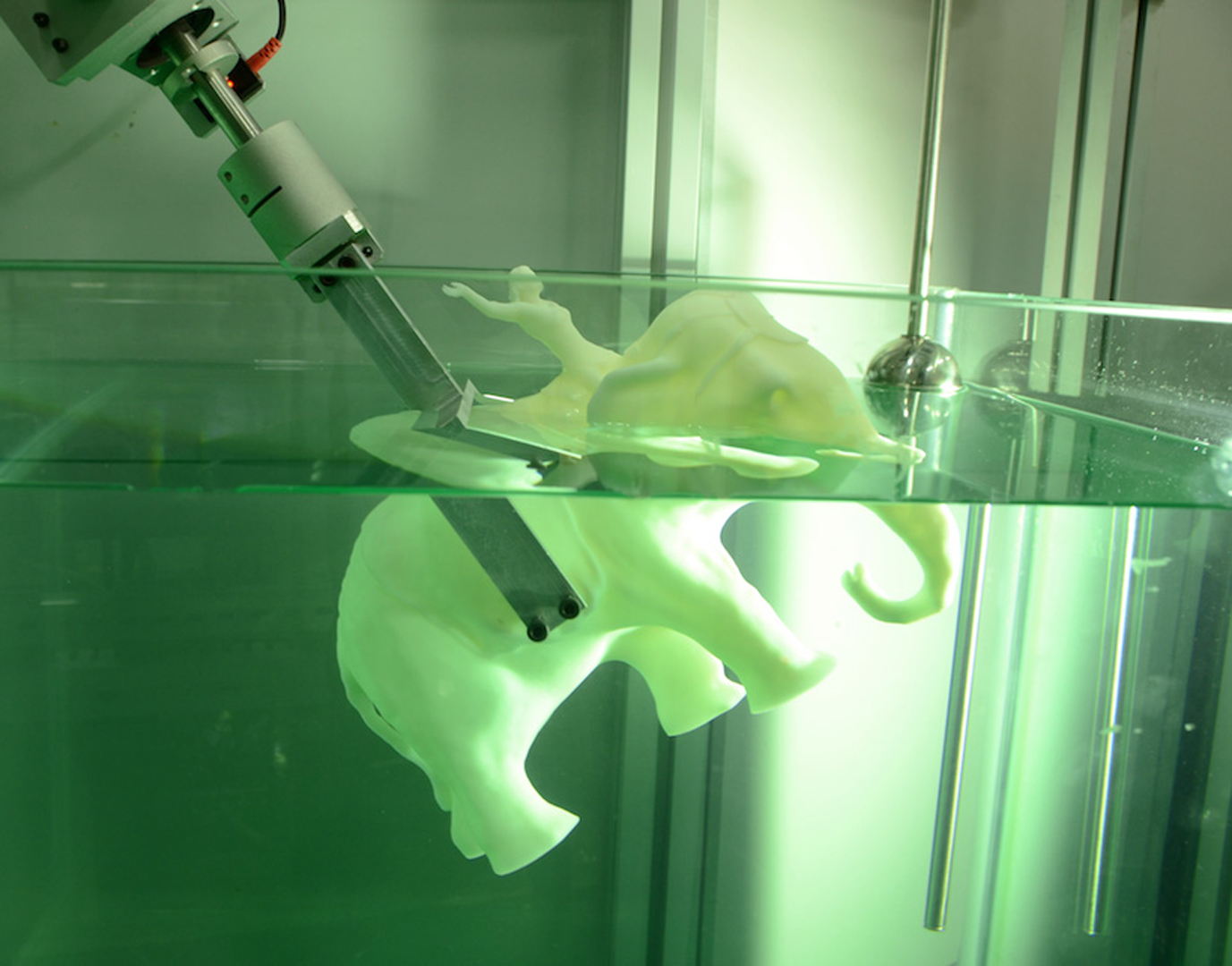
The paper presents a novel three-dimensional shape acquisition and reconstruction method based on the well-known Archimedes equality between fluid displacement and the submerged volume. By repeatedly dipping a shape in liquid in different orientations and measuring its volume displacement, we generate the dip transform: a novel volumetric shape representation that characterizes the object’s surface. The key feature of our method is that it employs fluid displacements as the shape sensor. Unlike optical sensors, the liquid has no line-of-sight requirements, it penetrates cavities and hidden parts of the object, as well as transparent and glossy materials, thus bypassing all visibility and optical limitations of conventional scanning devices. Our new scanning approach is implemented using a dipping robot arm and a bath of water, via which it measures the water elevation. We show results of reconstructing complex 3D shapes and evaluate the quality of the reconstruction with respect to the number of dips.
References:
1. Matthew Berger, Andrea Tagliasacchi, Lee M. Seversky, Pierre Alliez, Joshua A. Levine, Andrei Sharf, and Claudio T. Silva. 2014. State of the Art in Surface Reconstruction from Point Clouds. In Eurographics 2014 – State of the Art Reports, Sylvain Lefebvre and Michela Spagnuolo (Eds.). The Eurographics Association. Google ScholarCross Ref
2. R. N. Bracewell. 1956. Strip Integration in Radio Astronomy. Australian Journal of Physics 9 (June 1956), 198. Google ScholarCross Ref
3. Paolo Cignoni, Claudio Rocchini, and Roberto Scopigno. 1998. Metro: measuring error on simplified surfaces. In Computer Graphics Forum, Vol. 17. Wiley Online Library, 167–174. Google ScholarCross Ref
4. David Chin-Lung Fong and Michael Saunders. 2011. LSMR: An iterative algorithm for sparse least-squares problems. SIAM Journal on Scientific Computing 33, 5 (2011), 2950–2971. Google ScholarDigital Library
5. Richard Gordon, Robert Bender, and Gabor T. Herman. 1970. Algebraic reconstruction techniques (ART) for three-dimensional electron microscopy and x-ray photography. Journal of Theoretical Biology 29 (1970), 471–481. Google ScholarCross Ref
6. James Gregson, Michael Krimerman, Matthias B. Hullin, and Wolfgang Heidrich. 2012. Stochastic Tomography and Its Applications in 3D Imaging of Mixing Fluids. ACM Trans. Graph. 31, 4, Article 52 (July 2012), 10 pages. Google ScholarDigital Library
7. Gabor T. Herman. 2009. Fundamentals of Computerized Tomography: Image Reconstruction from Projections (2nd ed.). Springer Publishing Company, Incorporated. Google ScholarCross Ref
8. Ivo Ihrke, Kiriakos N. Kutulakos, Hendrik P. A. Lensch, Marcus Magnor, and Wolfgang Heidrich. 2010. Transparent and Specular Object Reconstruction. Computer Graphics Forum (2010). Google ScholarCross Ref
9. Takashi Ijiri, Shin Yoshizawa, Hideo Yokota, and Takeo Igarashi. 2014. Flower Modeling via X-ray Computed Tomography. ACM Trans. Graph. 33, 4, Article 48 (July 2014), 10 pages. Google ScholarDigital Library
10. Avinash C. Kak and Malcolm Slaney. 2001. Principles of computerized tomographic imaging. Society for Industrial and Applied Mathematics, Philadelphia (Pa.). http://opac.inria.fr/record=b1100961 Google ScholarCross Ref
11. Nobuyuki Otsu. 1975. A threshold selection method from gray-level histograms. Automatica 11, 285–296 (1975), 23–27.Google ScholarDigital Library
12. Christopher C Paige and Michael A Saunders. 1982. LSQR: An algorithm for sparse linear equations and sparse least squares. ACM transactions on mathematical software 8, 1 (1982), 43–71. Google ScholarDigital Library
13. Sandeep Patil and B Ravi. 2005. Voxel-based representation, display and thickness analysis of intricate shapes. In Ninth International Conference on Computer Aided Design and Computer Graphics (CAD-CG’05). IEEE, 6-pp. Google ScholarDigital Library
14. J. Radon. 1917. Über die Bestimmung von Funktionen durch ihre Integralwerte längs gewisser Mannigfaltigkeiten. Akad. Wiss. 69 (1917), 262–277.Google Scholar
15. Borislav Trifonov, Derek Bradley, and Wolfgang Heidrich. 2006. Tomographic Reconstruction of Transparent Objects. In Proceedings of the 17th Eurographics Conference on Rendering Techniques (EGSR ’06). Eurographics Association, Aire-la-Ville, Switzerland, Switzerland, 51–60. Google ScholarDigital Library
16. Shuang Zhao, Wenzel Jakob, Steve Marschner, and Kavita Bala. 2011. Building Volumetric Appearance Models of Fabric Using Micro CT Imaging. In ACM SIGGRAPH 2011 Papers (SIGGRAPH ’11). ACM, New York, NY, USA, Article 44, 10 pages. Google ScholarDigital Library