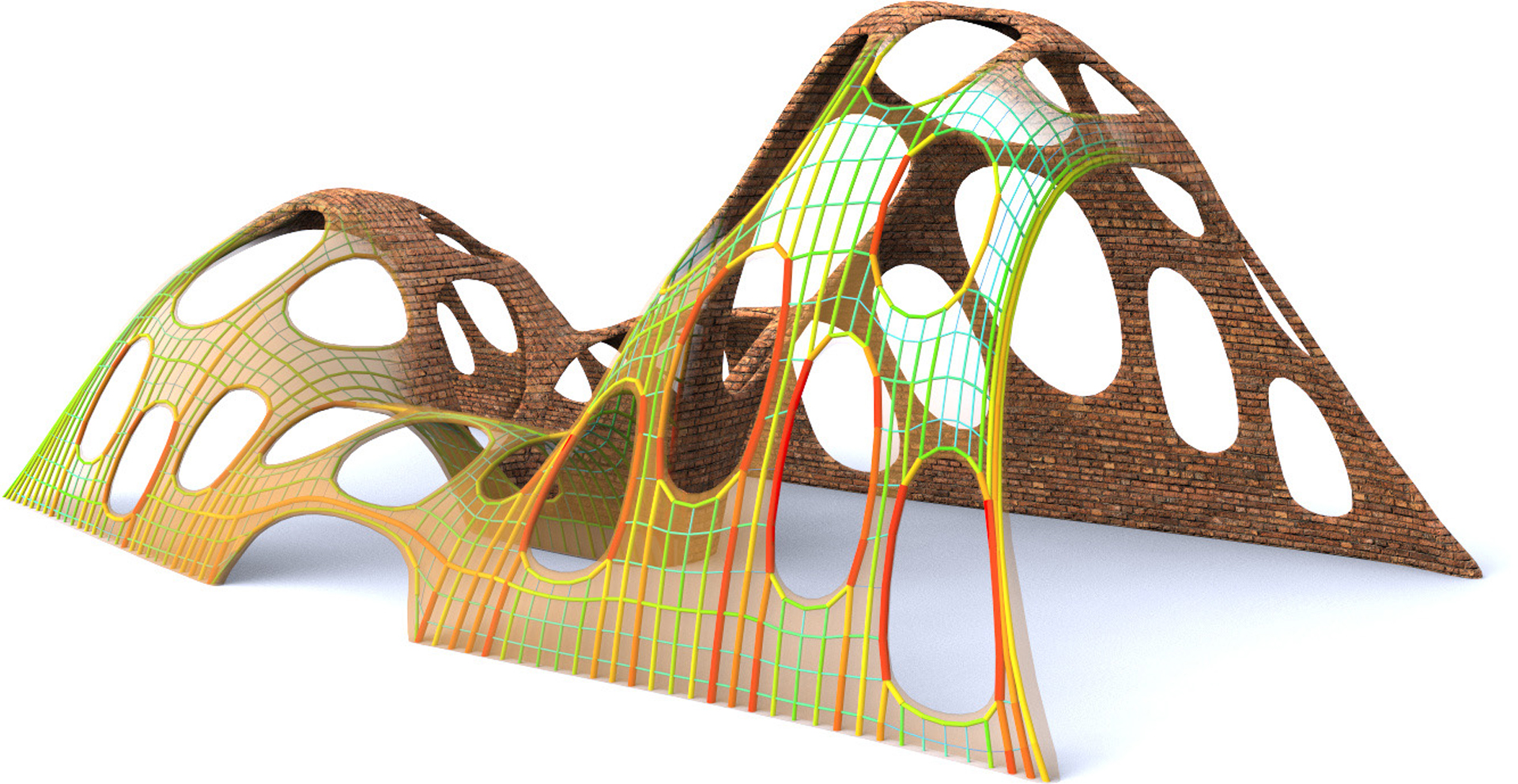
“Design of self-supporting surfaces” by Vouga, Hobinger, Wallner and Pottmann

Self-supporting masonry is one of the most ancient and elegant techniques for building curved shapes. Because of the very geometric nature of their failure, analyzing and modeling such strutures is more a geometry processing problem than one of classical continuum mechanics. This paper uses the thrust network method of analysis and presents an iterative nonlinear optimization algorithm for efficiently approximating freeform shapes by self-supporting ones. The rich geometry of thrust networks leads us to close connections between diverse topics in discrete differential geometry, such as a finite-element discretization of the Airy stress potential, perfect graph Laplacians, and computing admissible loads via curvatures of polyhedral surfaces. This geometric viewpoint allows us, in particular, to remesh self-supporting shapes by self-supporting quad meshes with planar faces, and leads to another application of the theory: steel/glass constructions with low moments in nodes.

1. Andreu, A., Gil, L., and Roca, P. 2007. Computational analysis of masonry structures with a funicular model. J. Engrg. Mechanics 133, 473–480.Google ScholarCross Ref
2. Ash, P., Bolker, E., Crapo, H., and Whiteley, W. 1988. Convex polyhedra, Dirichlet tessellations, and spider webs. In Shaping space (Northampton 1984). Birkhäuser, 231–250.Google Scholar
3. Barnes, M. R. 2009. Form finding and analysis of tension structures by dynamic relaxation. Int. J. Space Struct. 14, 2, 89–104.Google ScholarCross Ref
4. Block, P., and Lachauer, L. 2011. Closest-fit, compression-only solutions for free form shells. In IABSE — IASS 2011 London Symposium, Int. Ass. Shell Spatial Structures. {electronic}.Google Scholar
5. Block, P., and Ochsendorf, J. 2007. Thrust network analysis: A new methodology for three-dimensional equilibrium. J. Int. Assoc. Shell and Spatial Structures 48, 3, 167–173.Google Scholar
6. Block, P. 2009. Thrust Network Analysis: Exploring Three-dimensional Equilibrium. PhD thesis, Massachusetts Institute of Technology.Google Scholar
7. Block, P., 2011. Project webpage at http://block.arch.ethz.ch/projects/freeform-catalan-thin-tile-vaulting.Google Scholar
8. Bobenko, A., and Suris, Yu. 2008. Discrete differential geometry: Integrable Structure. No. 98 in Graduate Studies in Math. American Math. Soc.Google Scholar
9. Bobenko, A., Pottmann, H., and Wallner, J. 2010. A curvature theory for discrete surfaces based on mesh parallelity. Math. Annalen 348, 1–24.Google ScholarCross Ref
10. Cohen-Steiner, D., and Morvan, J.-M. 2003. Restricted Delaunay triangulations and normal cycle. In Proc. 19th Symp. Computational Geometry, ACM, 312–321. Google ScholarDigital Library
11. Davis, L., Rippmann, M., Pawlofsky, T., and Block, P. 2011. Efficient and expressive thin-tile vaulting using cardboard formwork. In IABSE — IASS 2011 London Symposium, Int. Ass. Shell Spatial Structures. {electronic}.Google Scholar
12. Fraternali, F., Angelillo, M., and Fortunato, A. 2002. A lumped stress method for plane elastic problems and the discrete-continuum approximation. Int. J. Solids Struct. 39, 6211–6240.Google ScholarCross Ref
13. Fraternali, F. 2010. A thrust network approach to the equilibrium problem of unreinforced masonry vaults via polyhedral stress functions. Mechanics Res. Comm. 37, 2, 198–204.Google ScholarCross Ref
14. Friedlander, M. P., 2007. BCLS: Bound constrained least squares. http://www.cs.ubc.ca/~mpf/bcls.Google Scholar
15. Giaquinta, M., and Giusti, E. 1985. Researches on the equilibrium of masonry structures. Archive for Rational Mechanics and Analysis 88, 4, 359–392.Google ScholarCross Ref
16. Green, A., and Zerna, W. 2002. Theoretical Elasticity. Dover. Reprint of the 1968 edition.Google Scholar
17. Gründig, L., Moncrieff, E., Singer, P., and Ströbel, D. 2000. A history of the principal developments and applications of the force density method in Germany 1970–1999. In 4th Int. Coll. Computation of Shell & Spatial Structures.Google Scholar
18. Heyman, J. 1966. The stone skeleton. Int. J. Solids Structures 2, 249–279.Google ScholarCross Ref
19. Heyman, J. 1995. The Stone Skeleton: Structural Engineering of Masonry Architecture. Cambridge University Press.Google Scholar
20. Heyman, J. 1998. Structural Analysis: A Historical Approach. Cambridge University Press.Google ScholarCross Ref
21. Kilian, A., and Ochsendorf, J. 2005. Particle-spring systems for structural form finding. J. Int. Assoc. Shell and Spatial Structures 46, 77–84.Google Scholar
22. Kotnik, T., and Weinstock, M. 2012. Material, form and force. Architectural Design 82, 104–111.Google ScholarCross Ref
23. Linkwitz, K., and Schek, H.-J. 1971. Einige Bemerkungen zur Berechnung von vorgespannten Seilnetzkonstruktionen. Ingenieur-Archiv 40, 145–158.Google ScholarCross Ref
24. Lipman, Y., Sorkine, O., Cohen-Or, D., Levin, D., Rossi, C., and Seidel, H. 2004. Differential coordinates for interactive mesh editing. In Shape Modeling Applications, 2004. Proceedings. IEEE, 181–190. Google ScholarDigital Library
25. Liu, Y., Pottmann, H., Wallner, J., Yang, Y.-L., and Wang, W. 2006. Geometric modeling with conical meshes and developable surfaces. ACM Trans. Graphics 25, 3, 681–689. Proc. SIGGRAPH. Google ScholarDigital Library
26. Livesley, R. K. 1992. A computational model for the limit analysis of three-dimensional masonry structures. Meccanica 27, 161–172.Google ScholarCross Ref
27. O’Dwyer, D. 1998. Funicular analysis of masonry vaults. Computers and Structures 73, 187–197.Google ScholarCross Ref
28. Paige, C. C., and Saunders, M. A. 1975. Solution of sparse indefinite systems of linear equations. SIAM J. Num. Analysis 12, 617–629.Google ScholarCross Ref
29. Pottmann, H., and Liu, Y. 2007. Discrete surfaces in isotropic geometry. In Mathematics of Surfaces XII, M. Sabin and J. Winkler, Eds. Springer, 341–363. Google ScholarDigital Library
30. Pottmann, H., Liu, Y., Wallner, J., Bobenko, A., and Wang, W. 2007. Geometry of multi-layer freeform structures for architecture. ACM Trans. Graphics 26, 3, #65,1–11. Proc. SIGGRAPH. Google ScholarDigital Library
31. Schiftner, A., and Balzer, J. 2010. Statics-sensitive layout of planar quadrilateral meshes. In Advances in Architectural Geometry 2010, C. Ceccato et al., Eds. Springer, Vienna, 221–236.Google Scholar
32. Schiftner, A. 2007. Planar quad meshes from relative principal curvature lines. Master’s thesis, TU Wien. http://dmg.tuwien.ac.at/aschiftner/doc/diplomarbeit.pdf.Google Scholar
33. Sorkine, O., Cohen-Or, D., and Toledo, S. 2003. High-pass quantization for mesh encoding. In Symposium Geometry processing, L. Kobbelt, P. Schröder, and H. Hoppe, Eds. Eurographics Assoc., 42–51. Google ScholarDigital Library
34. Van Mele, T., and Block, P. 2011. A novel form finding method for fabric formwork for concrete shells. J. Int. Assoc. Shell and Spatial Structures 52, 217–224.Google Scholar
35. Wächter, A., and Biegler, L. T. 2006. On the implementation of a primal-dual interior point filter line search algorithm for large-scale nonlinear programming. Math. Progr. 106, 25–57. Google ScholarDigital Library
36. Wardetzky, M., Mathur, S., Kälberer, F., and Grinspun, E. 2007. Discrete Laplace operators: No free lunch. In Symposium on Geometry Processing, A. Belyaev and M. Garland, Eds. Eurographics Assoc., 33–37. Google ScholarDigital Library
37. Whiting, E., Ochsendorf, J., and Durand, F. 2009. Procedural modeling of structurally-sound masonry buildings. ACM Trans. Graphics 28, 5, #112,1–9. Proc. SIGGRAPH Asia. Google ScholarDigital Library