“Computational design of weingarten surfaces” by Pellis, Kilian, Pottmann and Pauly

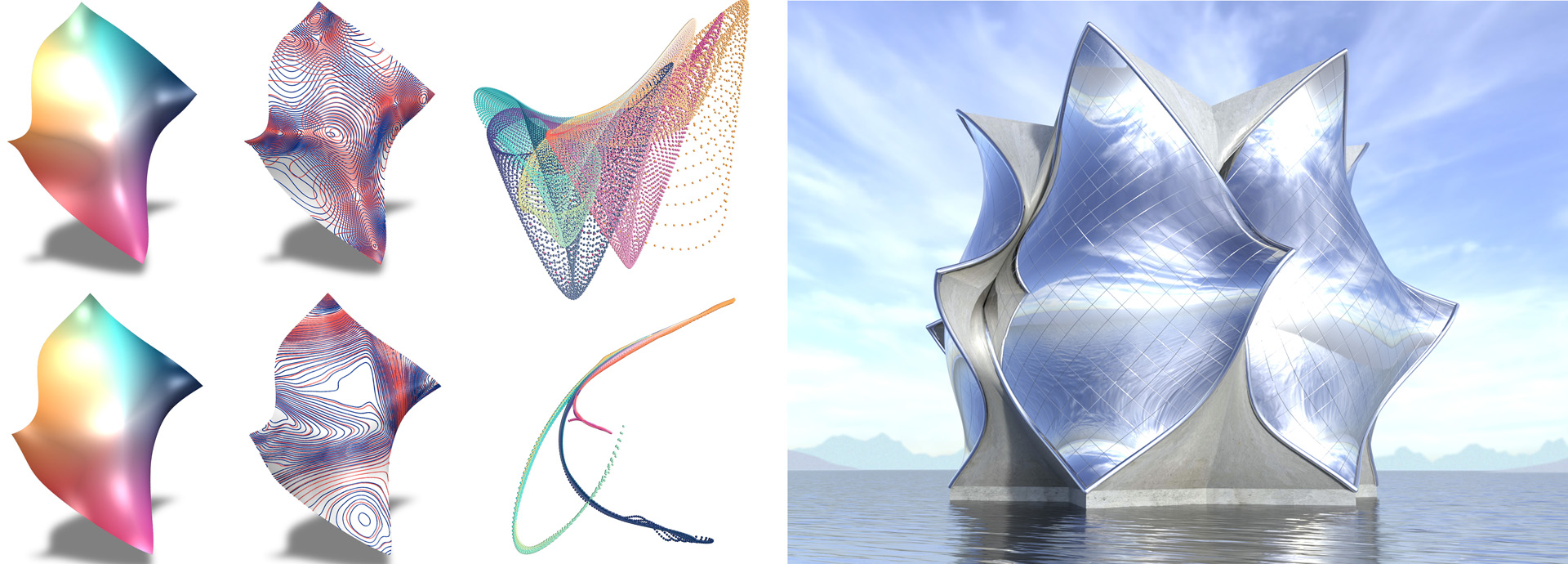
In this paper we study Weingarten surfaces and explore their potential for fabrication-aware design in freeform architecture. Weingarten surfaces are characterized by a functional relation between their principal curvatures that implicitly defines approximate local congruences on the surface. These symmetries can be exploited to simplify surface paneling of double-curved architectural skins through mold re-use.We present an optimization approach to find a Weingarten surface that is close to a given input design. Leveraging insights from differential geometry, our method aligns curvature isolines of the surface in order to contract the curvature diagram from a 2D region into a 1D curve. The unknown functional curvature relation then emerges as the result of the optimization. We show how a robust and efficient numerical shape approximation method can be implemented using a guided projection approach on a high-order B-spline representation. This algorithm is applied in several design studies to illustrate how Weingarten surfaces define a versatile shape space for fabrication-aware exploration in freeform architecture. Our optimization algorithm provides the first practical tool to compute general Weingarten surfaces with arbitrary curvature relation, thus enabling new investigations into a rich, but as of yet largely unexplored class of surfaces.

1. Pengbo Bo, Helmut Pottmann, Martin Kilian, Wenping Wang, and Johannes Wallner. 2011. Circular Arc Structures. ACM Trans. Graph. 30, 4, Article 101 (July 2011), 12 pages. Google ScholarDigital Library
2. Alexander Bobenko, Tim Hoffmann, and Boris Springborn. 2006. Minimal surfaces from circle patterns: Geometry from combinatorics. Ann. Math. 164 (2006), 231–264.Google ScholarCross Ref
3. Alexander I. Bobenko and Emanuel Huhnen-Venedey. 2012. Curvature line parametrized surfaces and orthogonal coordinate systems: discretization with Dupin cyclides. Geom. Dedicata 159 (2012), 207–237. Google ScholarCross Ref
4. Alexander I. Bobenko and Yuri B. Suris. 2008. Discrete differential geometry. Integrable structure. Graduate Studies in Mathematics, Vol. 98. American Mathematical Society, Providence, RI.Google Scholar
5. Kenneth A. Brakke. 1992. The Surface Evolver. Experimental Mathematics 1, 2 (1992), 141–165. Google ScholarCross Ref
6. Michael Eigensatz, Martin Kilian, Alexander Schiftner, Niloy J. Mitra, Helmut Pottmann, and Mark Pauly. 2010. Paneling Architectural Freeform Surfaces. ACM Trans. Graph. 29, 4 (2010), 45:1–45:10.Google ScholarDigital Library
7. Michael Eigensatz, Robert W. Sumner, and Mark Pauly. 2008. Curvature-Domain Shape Processing. Computer Graphics Forum 27, 2 (2008), 241–250. Google ScholarCross Ref
8. Konstantinos Gavriil, Ruslan Guseinov, Jesus Perez, Davide Pellis, Paul Henderson, Florian Rist, Helmut Pottmann, and Bernd Bickel. 2020. Computational design of cold bent glass facades. ACM Trans. Graphics 39, 6 (2020), 208:1–208:16. Proc. SIGGRAPH Asia.Google ScholarDigital Library
9. Heinz Hopf. 1951. Über Flächen mit einer Relation zwischen den Hauptkrümmungen. Math. Nachr. 4 (1951), 232–249.Google ScholarCross Ref
10. Emanuel Huhnen-Venedey and Thilo Rörig. 2014. Discretization of asymptotic line parametrizations using hyperboloid surface patches. Geom. Dedicata 168, 1 (2014), 265–289. Google ScholarCross Ref
11. Michael R. Jimenez, Christian Müller, and Helmut Pottmann. 2020. Discretizations of Surfaces with Constant Ratio of Principal Curvatures. Discrete Comput. Geom. 63, 3 (2020), 670–704.Google ScholarCross Ref
12. Wolfgang Kühnel. 2003. Differentialgeometrie (second ed.). Friedr. Vieweg & Sohn, Braunschweig. viii+256 pages. Kurven—Flächen—Mannigfaltigkeiten.Google Scholar
13. Juan Monterde. 2004. Bézier surfaces of minimal area: The Dirichlet approach. Computer Aided Geometric Design 21, 2 (2004), 117 — 136.Google ScholarDigital Library
14. Alvaro Pámpano. 2020. A variational characterization of profile curves of invariant linear Weingarten surfaces. Differential Geom. Appl. 68 (2020), 101564, 27.Google ScholarCross Ref
15. Hao Pan, Yi-King Choi, Yang Liu, Wenchao Hu, Qiang Du, Konrad Polthier, Caiming Zhang, and Wenping Wang. 2012. Robust Modeling of Constant Mean Curvature Surfaces. ACM Trans. Graph. 31, 4, Article 85 (2012), 11 pages. Google ScholarDigital Library
16. Qing Pan and Guoliang Xu. 2011. Construction of minimal subdivision surface with a given boundary. Computer-Aided Design 43, 4 (2011), 374 — 380.Google ScholarDigital Library
17. Davide Pellis, Martin Kilian, Hui Wang, Caigui Jiang, Christian Müller, and Helmut Pottmann. 2020a. Architectural freeform surfaces designed for cost-effective paneling through mold re-use. In Advances in Architectural Geometry.Google Scholar
18. Davide Pellis, Hui Wang, Florian Rist, Martin Kilian, Helmut Pottmann, and Christian Müller. 2020b. Principal Symmetric Meshes. ACM Trans. Graphics 39, 4 (2020), 127:1–127:17.Google ScholarDigital Library
19. Ulrich Pinkall and Konrad Polthier. 1993. Computing discrete minimal surfaces and their conjugates. Experiment. Math. 2, 1 (1993), 15–36. https://projecteuclid.org:443/euclid.em/1062620735Google ScholarCross Ref
20. Helmut Pottmann, Michael Eigensatz, Amir Vaxman, and Johannes Wallner. 2015. Architectural Geometry. Computers and Graphics 47 (2015), 145–164.Google ScholarDigital Library
21. Hartmut Prautzsch, Wolfgang Boehm, and Marco Paluszny. 2002. Bézier and B-Spline Techniques. Springer-Verlag.Google Scholar
22. Eike Schling. 2018. Repetitive Structures. Ph.D. Dissertation. TU Munich.Google Scholar
23. Eike Schling, Martin Kilian, Hui Wang, Denis Schikore, and Helmut Pottmann. 2018. Design and construction of curved support structures with repetitive parameters. In Adv. in Architectural Geometry, Lars Hesselgren et al. (Ed.). Klein Publ. Ltd, 140–165.Google Scholar
24. Chengcheng Tang, Xiang Sun, Alexandra Gomes, Johannes Wallner, and Helmut Pottmann. 2014. Form-finding with Polyhedral Meshes Made Simple. ACM Trans. Graph. 33, 4 (2014), 70:1–70:9.Google ScholarDigital Library
25. Xavier Tellier. 2020. Morphogenesis of curved structural envelopes under fabrication constraints. Ph.D. Dissertation. Univ. Paris-Est.Google Scholar
26. Xavier Tellier, Cyril Douthe, Laurent Hauswirth, and Olivier Baverel. 2019. Linear Weingarten surfaces for conceptual design of double-curvature envelopes. In Proceedings Int. Symposium on Conceptual Design of Structures, Madrid.Google Scholar
27. Bruce van Brunt and Katina Grant. 1994. Hyperbolic Weingarten Surfaces. Math. Proc. Camb. Phil. Soc. 116 (1994), 489–504.Google ScholarCross Ref
28. Bruce van Brunt and Katina Grant. 1996. Potential applications of Weingarten surfaces in CAGD, Part I: Weingarten surfaces and surface shape investigation. Computer Aided Geometric Design 13, 6 (1996), 569 — 582.Google ScholarDigital Library
29. Konrad Voss. 1959. Über geschlossene Weingartensche Flächen. Math. Annalen 138 (1959), 42–54.Google ScholarCross Ref
30. Julius Weingarten. 1861. Über eine Klasse aufeinander abwickelbarer Flächen. J. reine u. angewandte Mathematik 59 (1861), 382–393.Google Scholar
31. Gang Xu and Guozhao Wang. 2010. Quintic parametric polynomial minimal surfaces and their properties. Differential Geometry and its Applications 28, 6 (2010), 697 — 704.Google Scholar
32. Gang Xu, Yaguang Zhu, Guozhao Wang, Andre Galligo, Li Zhang, and Kin chuen Hui. 2015. Explicit form of parametric polynomial minimal surfaces with arbitrary degree. Appl. Math. Comput. 259 (2015), 124 — 131.Google ScholarDigital Library