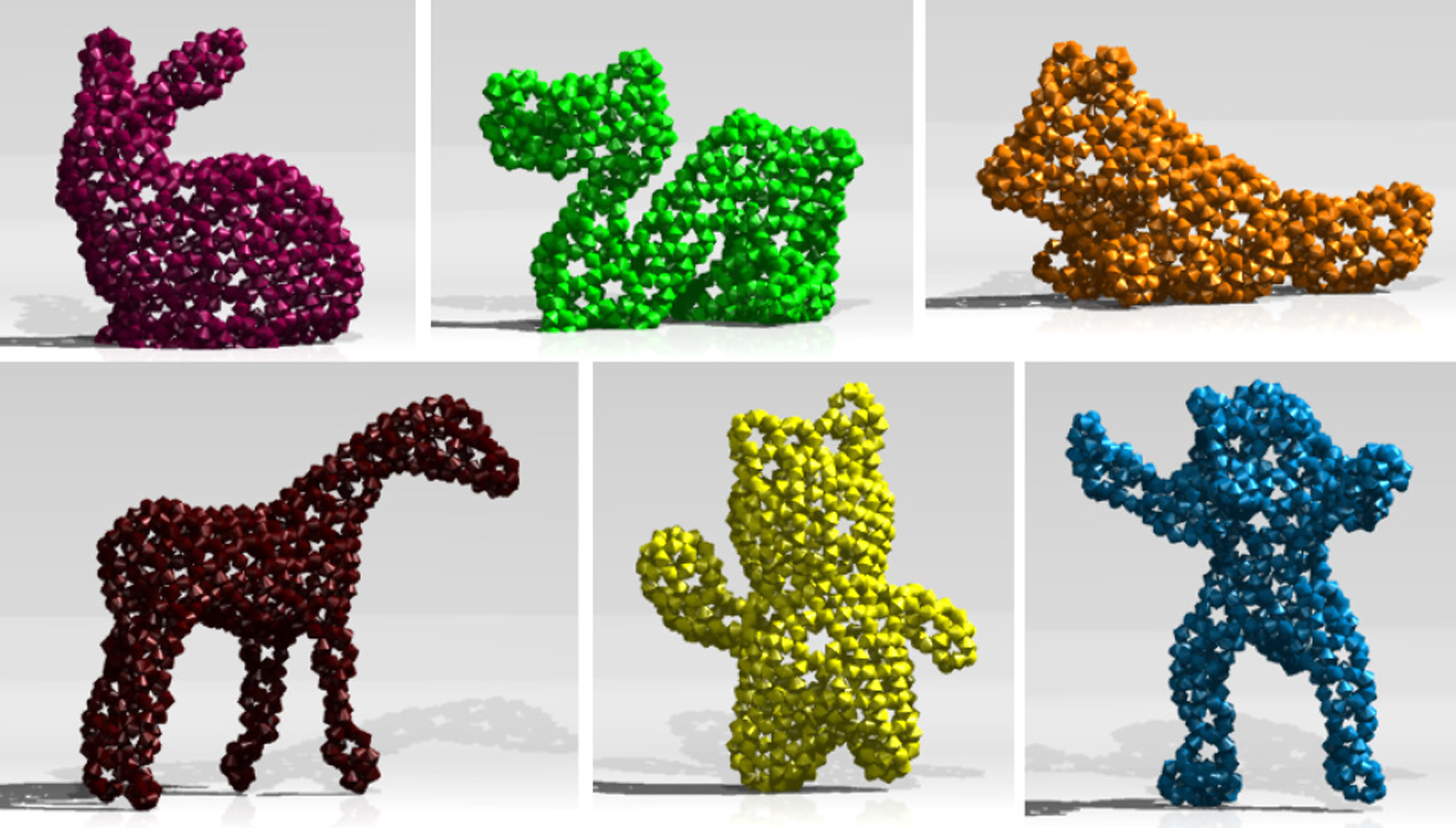“Beady: interactive beadwork design and construction” by Igarashi, Igarashi and Mitani

We introduce the interactive system “Beady” to assist the design and construction of customized 3D beadwork. The user first creates a polygonal mesh model called the design model that represents the overall structure of the beadwork. Each edge of the mesh model corresponds to a bead in the beadwork. We provide two methods to create the design model. One is interactive modeling from scratch. The user defines the mesh topology with gestural interaction and the system continuously adjusts edge lengths by considering the physical constraints among neighboring beads. The other is automatic conversion that takes an existing polygonal model as input and generates a near-hexagonal mesh model with a near-uniform edge length as output. The system then converts the design model into a beadwork model with the appropriate wiring. Computation of an appropriate wiring path requires careful consideration, and we present an algorithm based on face stripification of the mesh. The system also provides a visual step-by-step guide to assist the manual beadwork construction process. We show several beadwork designs constructed by the authors and by test users using the system.

1. Agrawala, M., Phan, D., Heiser, J., Haymaker, J., Klingner, J., Hanrahan, P., and Tversky, B. 2003. Designing effective step-by-step assembly instructions. ACM Trans. Graph. 22, 3, 828–837. Google ScholarDigital Library
2. Cohen-Steiner, D., Alliez, P., and Desbrun, M. 2004. Variational shape approximation. ACM Trans. Graph. 23, 3, 905–914. Google ScholarDigital Library
3. Decaudin, P., Julius, D., Wither, J., Boissieux, L., Sheffer, A., and Cani, M.-P. 2006. Virtual Garments: A fully geometric approach for clothing design. Comput. Graph. Forum 25, 3, 625–634.Google ScholarCross Ref
4. Eigensatz, M., Kilian, M., Schiftner, A., Mitra, N. J., Pottmann, H., and Pauly, M. 2010. Paneling architectural freeform surfaces. ACM Trans. Graph. 29, 4, 45:1–45:10. Google ScholarDigital Library
5. Evans, F., Skiena, S., and Varshney, A. 1996. Optimizing triangle strips for fast rendering. In Proc. of the 7th Conference on Visualization ’96, 319–326. Google ScholarDigital Library
6. Freese, A. 2007. Lovable Beaded Creatures. Sterling Pub Co Inc.Google Scholar
7. Fu, C.-W., Lai, C.-F., He, Y., and Cohen-Or, D. 2010. K-set tilable surfaces. ACM Trans. Graph. 29, 4, 44:1–44:6. Google ScholarDigital Library
8. Garland, M., and Heckbert, P. S. 1997. Surface simplification using quadric error metrics. In Proc. of SIGGRAPH ’97, 209–216. Google ScholarDigital Library
9. Igarashi, T., and Hughes, J. F. 2003. Smooth meshes for sketch-based freeform modeling. In Proc. of the 2003 Symposium on Interactive 3D Graphics, 139–142. Google ScholarDigital Library
10. Igarashi, Y., Igarashi, T., and Suzuki, H. 2008. Knitting a 3D model. Comput. Graph. Forum 27, 7, 1737–1743.Google ScholarCross Ref
11. Iizuka, S., Endo, Y., Mitani, J., Kanamori, Y., and Fukui, Y. 2011. An interactive design system for pop-up cards with a physical simulation. Vis. Comput. 27, 6-8, 605–612. Google ScholarDigital Library
12. Julius, D., Kraevoy, V., and Sheffer, A. 2005. D-charts: Quasi-developablemesh segmentation. Comput. Graph. Forum 24, 3, 581–590.Google ScholarCross Ref
13. Leblanc, L., Houle, J., and Poulin, P. 2011. Modeling with blocks. Vis. Comput. 27, 6-8, 555–563. Google ScholarDigital Library
14. Lévy, B., and Liu, Y. 2010. Lp centroidal voronoi tessellation and its applications. ACM Trans. Graph. 29, 4, 119:1–119:11. Google ScholarDigital Library
15. Li, W., Ritter, L., Agrawala, M., Curless, B., and Salesin, D. 2007. Interactive cutaway illustrations of complex 3D models. ACM Trans. Graph. 26, 3. Google ScholarDigital Library
16. Li, W., Agrawala, M., Curless, B., and Salesin, D. 2008. Automated generation of interactive 3D exploded view diagrams. ACM Trans. Graph. 27, 3, 101:1–101:7. Google ScholarDigital Library
17. Li, X.-Y., Shen, C.-H., Huang, S.-S., Ju, T., and Hu, S.-M. 2010. Popup: automatic paper architectures from 3D models. ACM Trans. Graph. 29, 4, 111:1–111:9. Google ScholarDigital Library
18. Li, X.-Y., Ju, T., Gu, Y., and Hu, S.-M. 2011. A geometric study of v-style pop-ups: theories and algorithms. ACM Trans. Graph. 30, 4,98:1–98:10. Google ScholarDigital Library
19. Liu, Y., Pottmann, H., Wallner, J., Yang, Y.-L., and Wang, W. 2006. Geometric modeling with conical meshes and developable surfaces. ACM Trans. Graph. 25, 3, 681–689. Google ScholarDigital Library
20. Lo, K.-Y., Fu, C.-W., and Li, H. 2009. 3D polyomino puzzle. ACM Trans. Graph. 28, 5, 157:1–157:8. Google ScholarDigital Library
21. Maki, Y. 2004. The Motifs of Puppy. Gakken (in Japanese).Google Scholar
22. Markosian, L., Cohen, J. M., Crulli, T., and Hughes, J. 1999. Skin: a constructive approach to modeling free-form shapes. In Proc. of SIGGRAPH ’99, 393–400. Google ScholarDigital Library
23. Mitani, J., and Suzuki, H. 2004. Making papercraft toys from meshes using strip-based approximate unfolding. ACM Trans. Graph. 23, 3, 259–263. Google ScholarDigital Library
24. Mori, Y., and Igarashi, T. 2007. Plushie: an interactive design system for plush toys. ACM Trans. Graph. 26, 3, 45:1–45:8. Google ScholarDigital Library
25. Nieser, M., Palacios, J., Polthier, K., and Zhang, E. 2010. Hexagonal global parameterization of arbitrary surfaces. In ACM SIGGRAPH ASIA 2010 Sketches, 5:1–5:2. Google ScholarDigital Library
26. Pottmann, H., Huang, Q., Deng, B., Schiftner, a., Kilian, M., Guibas, L., and Wallner, J. 2010. Geodesic patterns. ACM Trans. Graph. 29, 4, 43:1–43:10. Google ScholarDigital Library
27. Schiftner, a., Höbinger, M., Wallner, J., and Pottmann, H. 2009. Packing circles and spheres on surfaces. ACM Trans. Graph. 28, 5, 139:1–139:8. Google ScholarDigital Library
28. Shatz, I., Tal, A., and Leifman, G. 2006. Paper craft models from meshes. Vis. Comput. 22, 9, 825–834. Google ScholarDigital Library
29. Singh, M., and Schaefer, S. 2010. Triangle surfaces with discrete equivalence classes. ACM Trans. Graph. 29, 4, 46:1–46:7. Google ScholarDigital Library
30. Taubin, G., and Rossignac, J. 1998. Geometric compression through topological surgery. ACM Trans. Graph. 17, 2, 84–115. Google ScholarDigital Library
31. Turk, G. 1992. Re-tiling polygonal surfaces. SIGGRAPH Comput. Graph. 26, 2, 55–64. Google ScholarDigital Library
32. Weyrich, T., Deng, J., Barnes, C., Rusinkiewicz, S., and Finkelstein, A. 2007. Digital bas-relief from 3D scenes. ACM Trans. Graph. 26, 3. Google ScholarDigital Library
33. Yan, D.-M., Lévy, B., Liu, Y., Sun, F., and Wang, W. 2009. isotropic remeshing with fast and exact computation of restricted voronoi diagram. In Proc. of the Symposium on Geometry Processing, 1445–1454. Google ScholarDigital Library