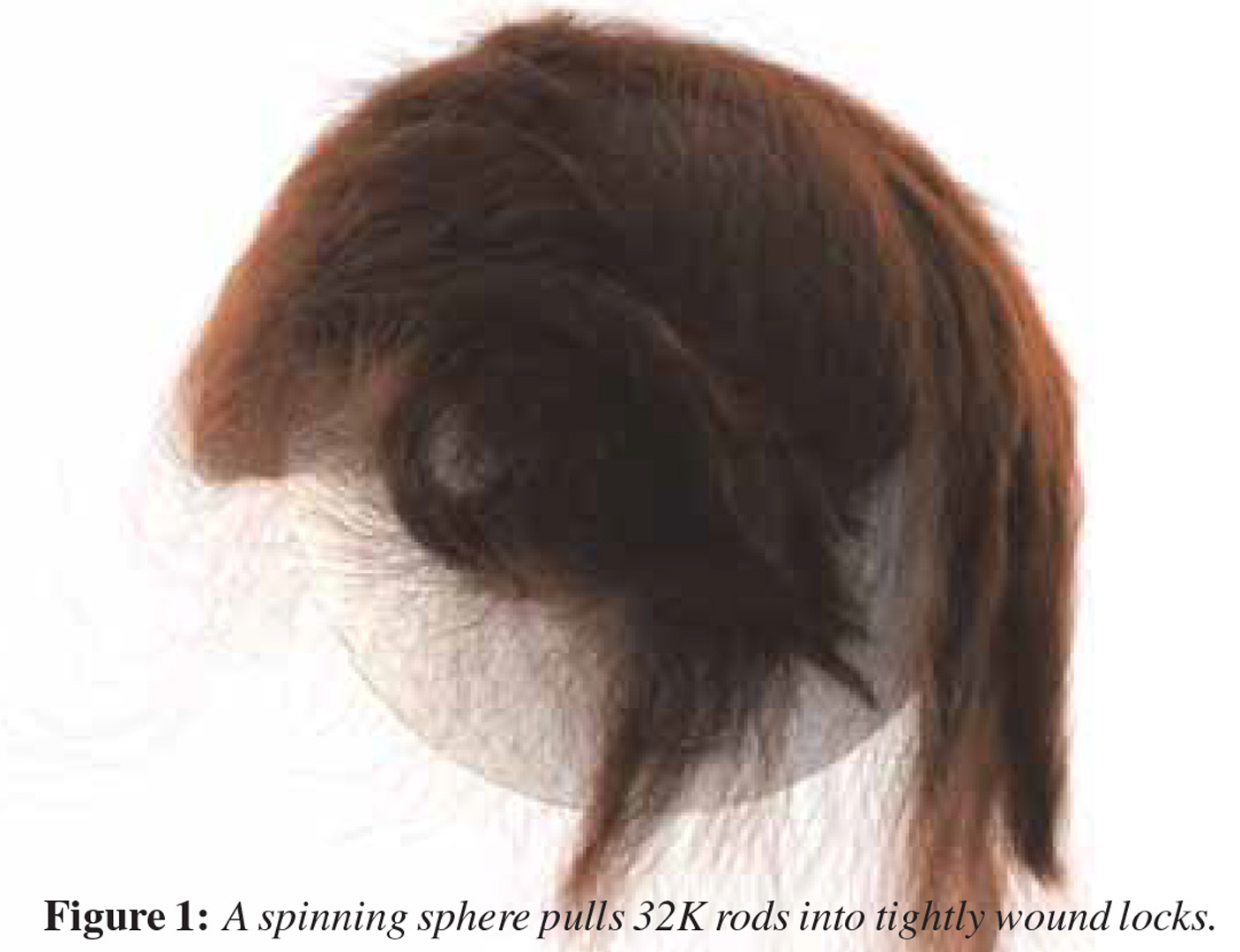
“Adaptive nonlinearity for collisions in complex rod assemblies” by Smith, Kaufman, Tamstorf, Grinspun and Aubry
Conference:
Type(s):
Title:
- Adaptive nonlinearity for collisions in complex rod assemblies
Session/Category Title: Hair & Collisions
Presenter(s)/Author(s):
Moderator(s):
Abstract:
We develop an algorithm for the efficient and stable simulation of large-scale elastic rod assemblies. We observe that the time-integration step is severely restricted by a strong nonlinearity in the response of stretching modes to transversal impact, the degree of this nonlinearity varying greatly with the shape of the rod. Building on these observations, we propose a collision response algorithm that adapts its degree of nonlinearity. We illustrate the advantages of the resulting algorithm by analyzing simulations involving elastic rod assemblies of varying density and scale, with up to 1.7 million individual contacts per time step.
References:
1. Alart, P., and Curnier, A. 1991. A mixed formulation for frictional contact problems prone to Newton like solution methods. Computer Methods in Applied Mechanics and Engineering 92, 3 (Nov.), 353–375. Google ScholarDigital Library
2. Allard, J., Faure, F., Courtecuisse, H., Falipou, F., Duriez, C., and Kry, P. G. 2010. Volume Contact Constraints at Arbitrary Resolution. ACM Trans. Graph. 29, 4 (July), 82:1–82:10. Google ScholarDigital Library
3. Baraff, D., and Witkin, A. 1998. Large Steps in Cloth Simulation. In Proceedings of SIGGRAPH 98, Annual Conference Series, 43–54. Google ScholarDigital Library
4. Baraff, D. 1989. Analytical Methods for Dynamic Simulation of Non-penetrating Rigid Bodies. Computer Graphics 23, 223–232. Google ScholarDigital Library
5. Bergou, M., Wardetzky, M., Robinson, S., Audoly, B., and Grinspun, E. 2008. Discrete Elastic Rods. ACM Trans. Graph. 27, 3 (Aug.), 63:1–63:12. Google ScholarDigital Library
6. Bergou, M., Audoly, B., Vouga, E., Wardetzky, M., and Grinspun, E. 2010. Discrete Viscous Threads. ACM Trans. Graph. 29, 4 (July), 116:1–116:10. Google ScholarDigital Library
7. Bertails-Descoubes, F., Cadoux, F., Daviet, G., and Acary, V. 2011. A Nonsmooth Newton Solver for Capturing Exact Coulomb Friction in Fiber Assemblies. ACM Trans. Graph. 30, 1 (Feb.), 6:1–6:14. Google ScholarDigital Library
8. Bonnefon, O., and Daviet, G. 2011. Quartic formulation of Coulomb 3D frictional contact. Tech. Rep. RT-0400, INRIA, Jan.Google Scholar
9. Bridson, R., Fedkiw, R., and Anderson, J. 2002. Robust Treatment of Collisions, Contact, and Friction for Cloth Animation. ACM Trans. Graph. 21, 3 (July), 594–603. Google ScholarDigital Library
10. Daviet, G., Bertails-Descoubes, F., and Boissieux, L. 2011. A Hybrid Iterative Solver for Robustly Capturing Coulomb Friction in Hair Dynamics. ACM Trans. Graph. 30, 6 (Dec.), 139:1–139:12. Google ScholarDigital Library
11. Duriez, C., Andriot, C., and Kheddar, A. 2004. Signorini’s contact model for deformable objects in haptic simulations. In IEEE/RSJ IROS, vol. 4, 3232–3237.Google Scholar
12. Duriez, C., Dubois, F., Kheddar, A., and Andriot, C. 2006. Realistic Haptic Rendering of Interacting Deformable Objects in Virtual Environments. IEEE Transactions on Visualization and Computer Graphics 12, 1 (Jan.), 36–47. Google ScholarDigital Library
13. Goyal, S., Ruina, A., and Papadopoulos, J. 1991. Planar sliding with dry friction Part 2. Dynamics of motion. Wear 143, 2, 331–352.Google ScholarCross Ref
14. Hadap, S., Cani, M.-P., Lin, M., Kim, T.-Y., Bertails, F., Marschner, S., Ward, K., and Kačić-Alesić, Z. 2007. Strands and Hair: Modeling, Animation, and Rendering. In ACM SIGGRAPH Courses, 1–150. Google ScholarDigital Library
15. Hairer, E., and Wanner, G. 2004. Solving Ordinary Differential Equations II: Stiff and Differential-Algebraic Problems, second ed. Springer.Google Scholar
16. Iben, H., Meyer, M., Petrovic, L., Soares, O., Anderson, J., and Witkin, A. 2013. Artistic Simulation of Curly Hair. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 63–71. Google ScholarDigital Library
17. Jean, M., and Moreau, J. J. 1992. Unilaterality and dry friction in the dynamics of rigid body collections. In Proceedings of Contact Mechanics International Symposium, vol. 1, 31–48.Google Scholar
18. Jourdan, F., Alart, P., and Jean, M. 1998. A Gauss-Seidel like algorithm to solve frictional contact problems. Computer Methods in Applied Mechanics and Engineering 155, 1 (Mar.), 31–47.Google ScholarCross Ref
19. Kaufman, D. M., Sueda, S., James, D. L., and Pai, D. K. 2008. Staggered Projections for Frictional Contact in Multibody Systems. ACM Trans. Graph. 27, 5 (Dec.), 164:1–164:11. Google ScholarDigital Library
20. Kikuchi, N., and Oden, J. T. 1988. Contact Problems in Elasticity: A Study of Variational Inequalities and Finite Element Methods, vol. 8 of SIAM Studies in Applied and Numerical Mathematics. Society for Industrial and Applied Mathematics.Google Scholar
21. Landau, L. D., and Lifshitz, E. 1986. Theory Of Elasticity, Course of Theoretical Physics, Vol. 7. Pergamon Press, Oxford.Google Scholar
22. McAdams, A., Selle, A., Ward, K., Sifakis, E., and Teran, J. 2009. Detail Preserving Continuum Simulation of Straight Hair. ACM Trans. Graph. 28, 3 (July), 62:1–62:6. Google ScholarDigital Library
23. Mirtich, B., and Canny, J. 1995. Impulse-based Simulation of Rigid Bodies. In Proceedings of the 1995 Symposium on Interactive 3D Graphics, 181–ff. Google ScholarDigital Library
24. Moreau, J. J. 1988. Unilateral Contact and Dry Friction in Finite Freedom Dynamics. Nonsmooth Mechanics and Applications, CISM Courses and Lectures, 302, 1–82.Google Scholar
25. Otaduy, M. A., Tamstorf, R., Steinemann, D., and Gross, M. 2009. Implicit Contact Handling for Deformable Objects. Computer Graphics Forum 28, 2, 559–568.Google ScholarCross Ref
26. Provot, X. 1997. Collision and self-collision handling in cloth model dedicated to design garments. In Computer Animation and Simulation, Eurographics, 177–189.Google Scholar
27. Robbins, C. R. 2012. Chemical and Physical Behavior of Human Hair, fifth ed. Springer.Google Scholar
28. Selle, A., Lentine, M., and Fedkiw, R. 2008. A Mass Spring Model for Hair Simulation. ACM Trans. Graph. 27, 3 (Aug.), 64:1–64:11. Google ScholarDigital Library
29. Spillmann, J., and Teschner, M. 2007. CORDE: Cosserat Rod Elements for the Dynamic Simulation of One-Dimensional Elastic Objects. In ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 63–72. Google ScholarDigital Library
30. Stewart, D. E. 2001. Finite-dimensional contact mechanics. Phil. Trans. R. Soc. Lond. A 359, 2467–2482.Google ScholarCross Ref
31. Stewart, D. E. 2011. Dynamics with Inequalities: Impacts and Hard Constraints. Society for Industrial and Applied Mathematics. Google ScholarDigital Library
32. Ward, K., Bertails, F., Kim, T.-Y., Marschner, S. R., Cani, M.-P., and Lin, M. C. 2007. A Survey on Hair Modeling: Styling, Simulation, and Rendering. IEEE Transactions on Visualization and Computer Graphics 13, 2 (Mar.), 213–234. Google ScholarDigital Library
33. Zheng, C., and James, D. L. 2011. Toward High-Quality Modal Contact Sound. ACM Trans. Graph. 30, 4 (July), 38:1–38:12. Google ScholarDigital Library