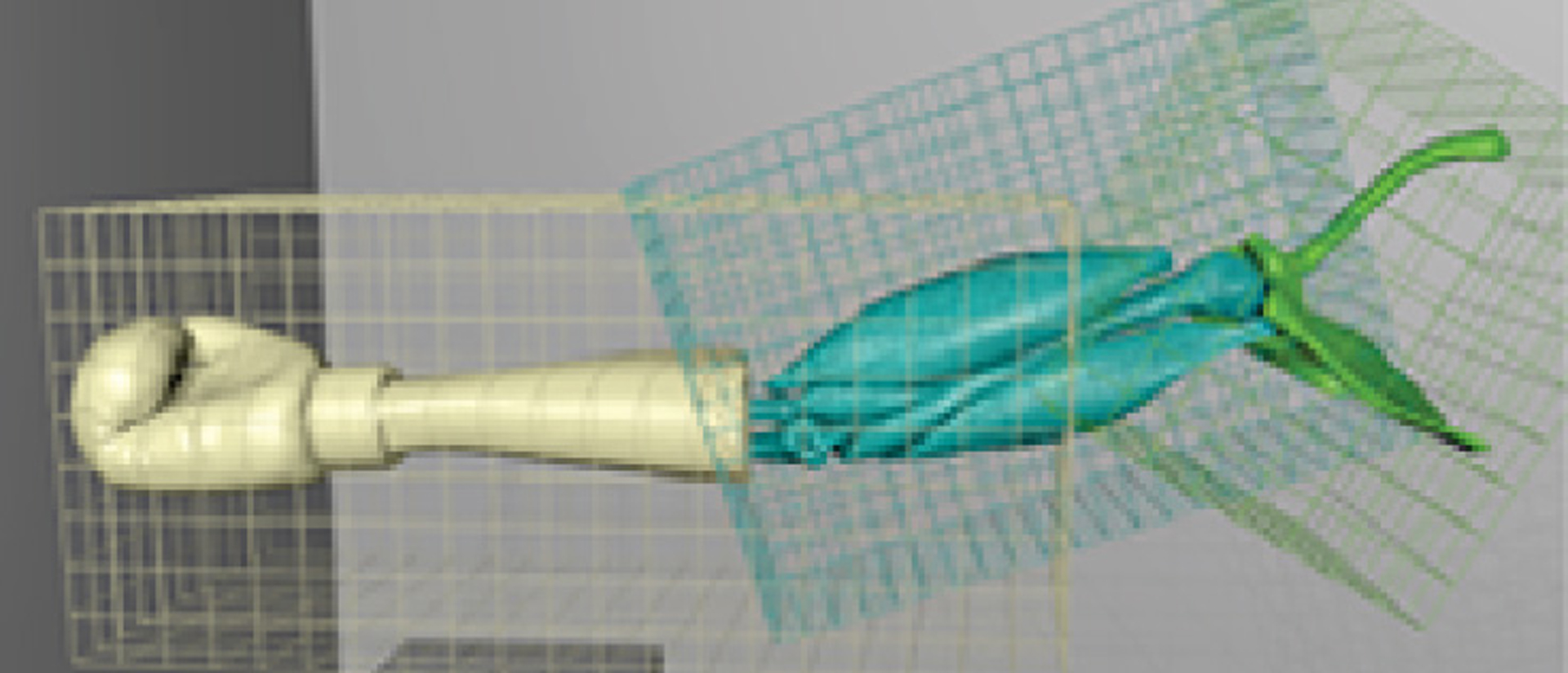
“Active volumetric musculoskeletal systems” by Fan, Litven and Pai
Conference:
Type(s):
Title:
- Active volumetric musculoskeletal systems
Session/Category Title: Stretching & Flowing
Presenter(s)/Author(s):
Abstract:
We introduce a new framework for simulating the dynamics of musculoskeletal systems, with volumetric muscles in close contact and a novel data-driven muscle activation model. Muscles are simulated using an Eulerian-on-Lagrangian discretization that handles volume preservation, large deformation, and close contact between adjacent tissues. Volume preservation is crucial for accurately capturing the dynamics of muscles and other biological tissues. We show how to couple the dynamics of soft tissues with Lagrangian multi-body dynamics simulators, which are widely available. Our physiologically based muscle activation model utilizes knowledge of the active shapes of muscles, which can be easily obtained from medical imaging data or designed to meet artistic needs. We demonstrate results with models derived from MRI data and models designed for artistic effect.
References:
1. Agur, A. M., Ng-Thow-Hing, V., Ball, K. A., Fiume, E., and McKee, N. H. 2003. Documentation and three-dimensional modelling of human soleus muscle architecture. Clinical Anatomy 16, 4, 285–293.Google ScholarCross Ref
2. Bargteil, A. W., Wojtan, C., Hodgins, J. K., and Turk, G. 2007. A Finite Element Method for Animating Large Viscoplastic Flow. ACM Trans. Graph. 26, 3 (July), 16:1–16:8. Google ScholarDigital Library
3. Blemker, S. S., and Delp, S. L. 2005. Three-dimensional representation of complex muscle architectures and geometries. Annals of Biomedical Engineering 33, 5 (May), 661–673.Google Scholar
4. Chen, D. T., and Zeltzer, D. 1992. Pump it up: Computer animation of a biomechanically based model of muscle using the finite element method. SIGGRAPH ’92, 89–98. Google ScholarDigital Library
5. Coros, S., Martin, S., Thomaszewski, B., Schumacher, C., Sumner, R., and Gross, M. 2012. Deformable objects alive! ACM Transactions on Graphics (TOG) 31, 4, 69. Google ScholarDigital Library
6. Delp, S. L., et al. 2007. OpenSim: open-source software to create and analyze dynamic simulations of movement. IEEE Trans Biomed Eng 54, 1940–1950.Google ScholarCross Ref
7. English, R. E., Qiu, L., Yu, Y., and Fedkiw, R. 2013. Chimera grids for water simulation. In Proceedings of the 12th ACM SIGGRAPH/Eurographics Symposium on Computer Animation, 85–94. Google ScholarDigital Library
8. Fan, Y., Litven, J., Levin, D. I. W., and Pai, D. K. 2013. Eulerian-on-lagrangian simulation. ACM Trans. Graph. 32, 3 (July), 22:1–22:9. Google ScholarDigital Library
9. Faure, F., Gilles, B., Bousquet, G., and Pai, D. K. 2011. Sparse meshless models of complex deformable solids. In ACM Transactions on Graphics (TOG), vol. 30, ACM, 73. Google ScholarDigital Library
10. Geijtenbeek, T., van de Panne, M., and van der Stappen, A. F. 2013. Flexible muscle-based locomotion for bipedal creatures. ACM Transactions on Graphics (TOG) 32, 6, 206. Google ScholarDigital Library
11. Gilles, B., Reveret, L., and Pai, D. 2010. Creating and animating subject-specific anatomical models. In Computer Graphics Forum, vol. 29, Wiley Online Library, 2340–2351.Google Scholar
12. Goldenthal, R., Harmon, D., Fattal, R., Bercovier, M., and Grinspun, E. 2007. Efficient simulation of inextensible cloth. ACM Transactions on Graphics (TOG) 26, 3, 49. Google ScholarDigital Library
13. Herzog, W. 2004. History dependence of skeletal muscle force production: implications for movement control. Hum Mov Sci 23 (Nov), 591–604.Google ScholarCross Ref
14. Holzbaur, K., Murray, W., and Delp, S. 2005. A model of the upper extremity for simulating musculoskeletal surgery and analyzing neuromuscular control. Annals of biomedical engineering 33, 6, 829–840.Google ScholarCross Ref
15. Hughes, T. 2000. The finite element method: linear static and dynamic finite element analysis. Dover Publications.Google Scholar
16. Irving, G., Schroeder, C., and Fedkiw, R. 2007. Volume conserving finite element simulations of deformable models. ACM Transactions on Graphics (TOG) 26, 3, 13. Google ScholarDigital Library
17. Ito, K., and Kunisch, K. 2008. Lagrange Multiplier Approach to Variational Problems and Applications. Google ScholarDigital Library
18. Lee, S.-H., and Terzopoulos, D. 2008. Spline joints for multibody dynamics. In ACM Transactions on Graphics (TOG), vol. 27, ACM, 22. Google ScholarDigital Library
19. Lee, S., Sifakis, E., and Terzopoulos, D. 2009. Comprehensive biomechanical modeling and simulation of the upper body. ACM Transactions on Graphics (TOG) 28, 4, 99. Google ScholarDigital Library
20. Lee, D., Glueck, M., Khan, A., Fiume, E., and Jackson, K. 2012. Modeling and simulation of skeletal muscle for computer graphics: A survey. Foundations and Trends in Computer Graphics and Vision 7, 4, 229–276. Google ScholarDigital Library
21. Levin, D. I. W., Litven, J., Jones, G. L., Sueda, S., and Pai, D. K. 2011. Eulerian solid simulation with contact. ACM Transactions on Graphics (TOG) 30, 4, 36. Google ScholarDigital Library
22. Levin, D. I. W., Gilles, B., Mädler, B., and Pai, D. K. 2011. Extracting skeletal muscle fiber fields from noisy diffusion tensor data. Medical Image Analysis 15, 3, 340–353.Google ScholarCross Ref
23. Li, D., Sueda, S., Neog, D. R., and Pai, D. K. 2013. Thin skin elastodynamics. ACM Transactions on Graphics (TOG) 32, 4, 49. Google ScholarDigital Library
24. Liu, L., Yin, K., Wang, B., and Guo, B. 2013. Simulation and control of skeleton-driven soft body characters. ACM Transactions on Graphics (TOG) 32, 6, 215. Google ScholarDigital Library
25. Maas, H., and Sandercock, T. G. 2010. Force transmission between synergistic skeletal muscles through connective tissue linkages. J. Biomed. Biotechnol. 2010, 575–672.Google ScholarCross Ref
26. Misztal, M., Erleben, K., Bargteil, A., Fursund, J., Christensen, B., Bærentzen, J., and Bridson, R. 2012. Multiphase flow of immiscible fluids on unstructured moving meshes. In Eurographics/ACM SIGGRAPH Symposium on Computer Animation, 97–106. Google ScholarDigital Library
27. Nardinocchi, P., and Teresi, L. 2007. On the active response of soft living tissues. Journal of Elasticity 88, 1, 27–39.Google ScholarCross Ref
28. Nealen, A., Müller, M., Keiser, R., Boxerman, E., and Carlson, M. 2006. Physically based deformable models in computer graphics. In Computer Graphics Forum, vol. 25, 809–836.Google ScholarCross Ref
29. Ng-Thow-Hing, V., and Fiume, E. 2002. Application-specific muscle representations. In Graphics Interface, vol. 2, Citeseer, 107–16.Google Scholar
30. Ng-Thow-Hing, V., Agur, A., and McKee, N. 2001. A muscle model that captures external shape, internal fibre architecture, and permits simulation of active contraction with volume preservation. In INTERNATIONAL SYMPOSIUM ON COMPUTER METHODS IN BIOMECHANICS & BIOMEDICAL ENGINEERING (5.: 2001: Rome). Anais. Rome.Google Scholar
31. Pai, D. K. 2010. Muscle mass in musculoskeletal models. J. Biomechanics 43, 11 (August), 2093–2098. DOI: 10.1016/j.jbiomech.2010.04.004.Google ScholarCross Ref
32. Patterson, T., Mitchell, N., and Sifakis, E. 2012. Simulation of complex nonlinear elastic bodies using lattice deformers. ACM Transactions on Graphics (TOG) 31, 6, 197. Google ScholarDigital Library
33. Schmid, J., Sandholm, A., Chung, F., Thalmann, D., Delingette, H., and Magnenat-Thalmann, N. 2009. Musculoskeletal simulation model generation from mri data sets and motion capture data. In Recent Advances in the 3D Physiological Human. Springer, 3–19.Google Scholar
34. Schumacher, C., Thomaszewski, B., Coros, S., Martin, S., Sumner, R., and Gross, M. 2012. Efficient simulation of example-based materials. In Proceedings of the ACM SIGGRAPH/Eurographics Symposium on Computer Animation, Eurographics Association, 1–8. Google ScholarDigital Library
35. Sifakis, E., and Barbic, J. 2012. Fem simulation of 3d deformable solids: a practitioner’s guide to theory, discretization and model reduction. In ACM SIGGRAPH 2012 Course Notes. Google ScholarDigital Library
36. Simo, J. C., and Taylor, R. L. 1991. Quasi-incompressible finite elasticity in principal stretches. continuum basis and numerical algorithms. Computer Methods in Applied Mechanics and Engineering 85, 3, 273–310. Google ScholarDigital Library
37. Sueda, S., Kaufman, A., and Pai, D. K. 2008. Musculotendon simulation for hand animation. ACM Transactions on Graphics (TOG) 27, 3, 83. Google ScholarDigital Library
38. Sueda, S., Jones, G. L., Levin, D. I., and Pai, D. K. 2011. Large-scale dynamic simulation of highly constrained strands. In ACM Transactions on Graphics (TOG), vol. 30, ACM, 39. Google ScholarDigital Library
39. Teran, J., Blemker, S., Hing, V., and Fedkiw, R. 2003. Finite volume methods for the simulation of skeletal muscle. In Proceedings of the 2003 ACM SIGGRAPH/Eurographics symposium on Computer animation, Eurographics Association, 68–74. Google ScholarDigital Library
40. Teran, J., Sifakis, E., Blemker, S., Ng-Thow-Hing, V., Lau, C., and Fedkiw, R. 2005. Creating and simulating skeletal muscle from the visible human data set. Visualization and Computer Graphics, IEEE Transactions on 11, 3, 317–328. Google ScholarDigital Library
41. Terzopoulos, D., Platt, J., Barr, A., and Fleischer, K. 1987. Elastically deformable models. In Computer Graphics (Proceedings of SIGGRAPH 87), vol. 21, ACM, 205–214. Google ScholarDigital Library
42. Wei, Q., and Pai, D. 2008. Physically consistent registration of extraocular muscle models from MRI. In Proc. IEEE Engineering in Medicine and Biology Society (EMBS) Annual Conference, 2237–2241.Google Scholar
43. Wei, Q., Sueda, S., Miller, J. M., Demer, J. L., and Pai, D. K. 2009. Template-based reconstruction of human extraocular muscles from magnetic resonance images. In ISBI’09. IEEE International Symposium on Biomedical Imaging, IEEE, 105–108. Google ScholarDigital Library
44. Weiss, J. A., Maker, B. N., and Govindjee, S. 1996. Finite element implementation of incompressible, transversely isotropic hyperelasticity. Computer methods in applied mechanics and engineering 135, 1, 107–128.Google Scholar
45. Wicke, M., Ritchie, D., Klingner, B. M., Burke, S., Shewchuk, J. R., and O’Brien, J. F. 2010. Dynamic local remeshing for elastoplastic simulation. ACM Trans. Graph. 29 (July), 49:1–49:11. Google ScholarDigital Library
46. Zajac, F. E. 1989. Muscle and tendon: Properties, models, scaling, and application to biomechanics and motor control. CRC Critical Reviews of Biomedical Engineering 17, 4, 359–411.Google Scholar