“Multi-species simulation of porous sand and water mixtures” by Tampubolon, Gast, Klár, Fu, Teran, et al. …
Conference:
Type(s):
Title:
- Multi-species simulation of porous sand and water mixtures
Session/Category Title: Fluids II
Presenter(s)/Author(s):
- Andre Pradhana Tampubolon
- Theodore Gast
- Gergely Klár
- Chuyuan Fu
- Joseph Teran
- Chenfanfu Jiang
- Ken Museth
Moderator(s):
Abstract:
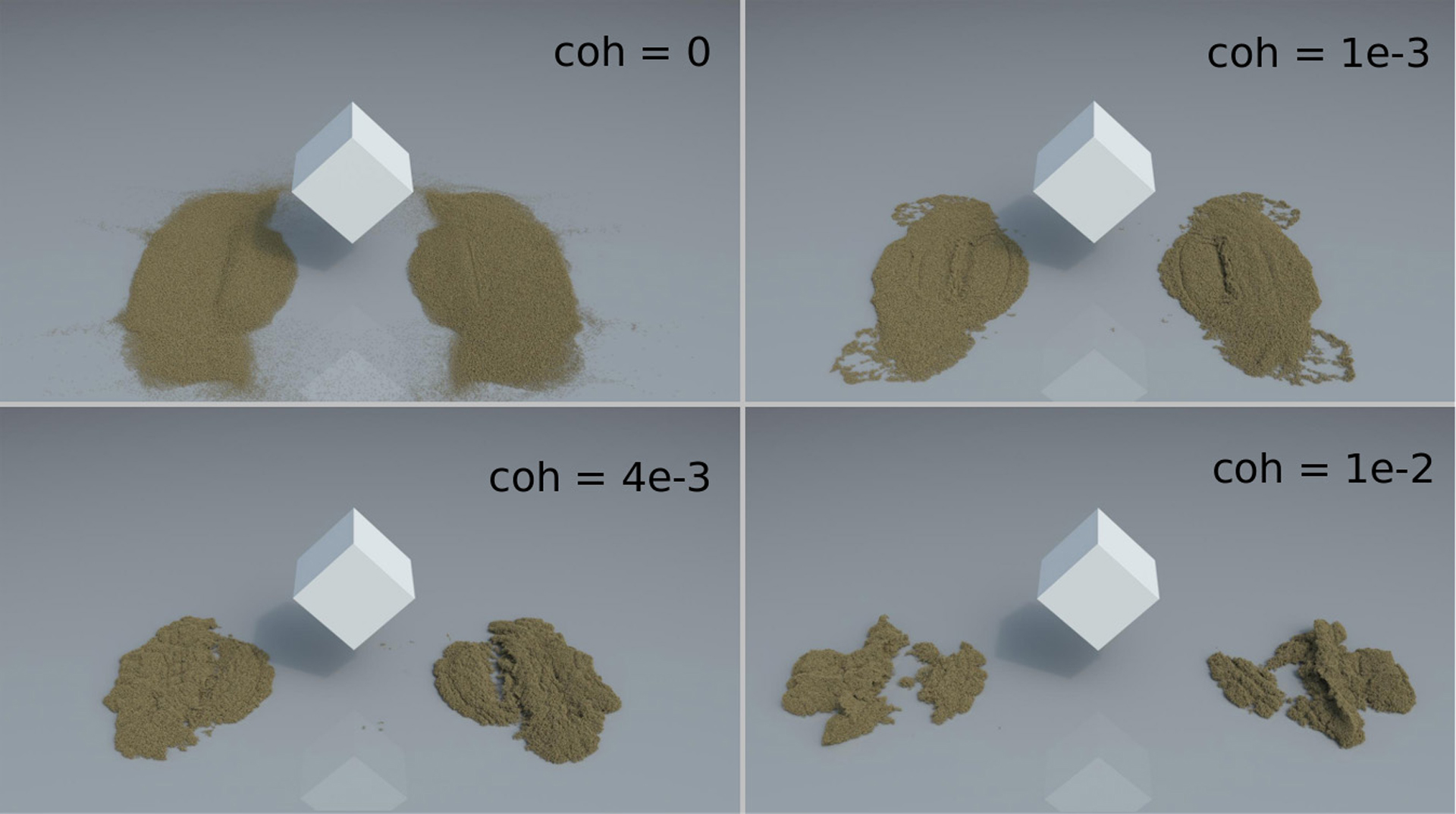
We present a multi-species model for the simulation of gravity driven landslides and debris flows with porous sand and water interactions. We use continuum mixture theory to describe individual phases where each species individually obeys conservation of mass and momentum and they are coupled through a momentum exchange term. Water is modeled as a weakly compressible fluid and sand is modeled with an elastoplastic law whose cohesion varies with water saturation. We use a two-grid Material Point Method to discretize the governing equations. The momentum exchange term in the mixture theory is relatively stiff and we use semi-implicit time stepping to avoid associated small time steps. Our semi-implicit treatment is explicit in plasticity and preserves symmetry of force linearizations. We develop a novel regularization of the elastic part of the sand constitutive model that better mimics plasticity during the implicit solve to prevent numerical cohesion artifacts that would otherwise have occurred. Lastly, we develop an improved return mapping for sand plasticity that prevents volume gain artifacts in the traditional Drucker-Prager model.
References:
1. K. Abe, K. Soga, and S. Bandara. 2014. Material Point Method for Coupled Hydromechanical Problems. J Geotech Geoenv Eng 140, 3 (2014), 04013033.Google ScholarCross Ref
2. I. Alduán and M. Otaduy. 2011. SPH granular flow with friction and cohesion. In Proc ACM SIGGRAPH/Eurograph Symp Comp Anim. 25–32. Google ScholarDigital Library
3. R. Atkin and R. Craine. 1976. Continuum theories of mixtures: basic theory and historical development. Quart J Mech App Math 29, 2 (1976), 209–244. Google ScholarCross Ref
4. S. Bandara, A. Ferrari, and L. Laloui. 2016. Modelling landslides in unsaturated slopes subjected to rainfall infiltration using material point method. Int J Num Anal Meth Geomech 40, 9 (2016), 1358–1380. Google ScholarCross Ref
5. S. Bandara and K. Soga. 2015. Coupling of soil deformation and pore fluid flow using material point method. Comp Geotech 63 (2015), 199–214. Google ScholarCross Ref
6. K. Bao, X. Wu, H. Zhang, and E. Wu. 2010. Volume fraction based miscible and immiscible fluid animation. Comp Anim Virtual Worlds 21, 3–4 (2010), 401–410.Google Scholar
7. M. Becker and M. Teschner. 2007. Weakly compressible SPH for free surface flows. In ACM SIGGRAPH/Eurograph Symp Comp Anim, D. Metaxas and J. Popovic (Eds.). The Eurographics Association.Google Scholar
8. J. Bonet and R. Wood. 2008. Nonlinear continuum mechanics for finite element analysis. Cambridge University Press. Google ScholarCross Ref
9. R. Borja. 2006. On the mechanical energy and effective stress in saturated and unsaturated porous continua. Int J Solids Struct 43 (2006), 1764–1786. Google ScholarCross Ref
10. G. Daviet and F. Bertails-Descoubes. 2016. A Semi-implicit Material Point Method for the Continuum Simulation of Granular Materials. ACM Trans Graph 35, 4 (2016), 102:1–102:13.Google ScholarDigital Library
11. D. Drucker and W. Prager. 1952. Soil mechanics and plasticity analysis or limit design. Quart App Math 10 (1952), 157–165. Google ScholarCross Ref
12. D. Drumheller. 2000. On theories for reacting immiscible mixtures. Int J Eng Sci 38, 3 (2000), 347 — 382. Google ScholarCross Ref
13. S. Dunatunga and K. Kamrin. 2015. Continuum modelling and simulation of granular flows through their many phases. J Fluid Mech 779 (2015), 483–513. Google ScholarCross Ref
14. X. He, H. Wang, F. Zhang, H. Wang, G. Wang, K. Zhou, and E. Wu. 2015. Simulation of Fluid Mixing with Interface Control. In Proc ACM SIGGRAPH / Eurograph Symp Comp Anim. ACM, 129–135. Google ScholarDigital Library
15. M. Ihmsen, A. Wahl, and M. Teschner. 2013. A Lagrangian framework for simulating granular material with high detail. Comp Graph 37, 7 (2013), 800–808. Google ScholarDigital Library
16. I. Jassim, D. Stolle, and P. Vermeer. 2013. Two-phase dynamic analysis by material point method. Int J Num Anal Meth Geomech 37 (2013), 2502–2522. Google ScholarCross Ref
17. C. Jiang, C. Schroeder, A. Selle, J. Teran, and A. Stomakhin. 2015. The Affine Particle-In-Cell Method. ACM Trans Graph 34, 4 (2015), 51:1–51:10.Google ScholarDigital Library
18. N. Kang, J. Park, J. Noh, and S. Shin. 2010. A Hybrid Approach to Multiple Fluid Simulation using Volume Fractions. Comp Graph Forum 29, 2 (2010), 685–694.Google ScholarCross Ref
19. G. Klár, T. Gast, A. Pradhana, C. Fu, C. Schroeder, C. Jiang, and J. Teran. 2016. Druckerprager Elastoplasticity for Sand Animation. ACM Trans Graph 35, 4 (2016), 103:1–103:12.Google ScholarDigital Library
20. T. Lenaerts and P. Dutré. 2009. Mixing Fluids and Granular Materials. Comp Graph Forum 28, 2 (2009), 213–218.Google ScholarCross Ref
21. S. Liu, Z. Wang, Z. Gong, and Q. Peng. 2008. Simulation of Atmospheric Binary Mixtures Based on Two-fluid Model. Graph Mod 70, 6 (2008), 117–124. Google ScholarDigital Library
22. F. Losasso, J. Talton, N. Kwatra, and R. Fedkiw. 2008. Two-Way Coupled SPH and Particle Level Set Fluid Simulation. IEEE Trans Visu Comp Graph 14, 4 (2008), 797–804. Google ScholarDigital Library
23. P. Mackenzie-Helnwein, P. Arduino, W. Shin, J. Moore, and G. Miller. 2010. Modeling strategies for multiphase drag interactions using the material point method. Int J Num Meth Eng 83, 3 (2010), 295–322. Google ScholarCross Ref
24. C. Mast, P. Arduino, G. Miller, and M. Peter. 2014. Avalanche and landslide simulation using the material point method: flow dynamics and force interaction with structures. Comp Geosci 18, 5 (2014), 817–830. Google ScholarCross Ref
25. V. Mihalef, D. Metaxas, and M. Sussman. 2009. Simulation of two-phase flow with sub-scale droplet and bubble effects. Comp GraphForum 28, 2 (2009), 229–238. Google ScholarCross Ref
26. Ken Museth. 2013. VDB: High-resolution Sparse Volumes with Dynamic Topology. ACM Trans. Graph. 32, 3, Article 27 (July 2013), 22 pages.Google ScholarDigital Library
27. R. Narain, A. Golas, and M. Lin. 2010. Free-flowing granular materials with two-way solid coupling. ACM Trans Graph 29, 6 (2010), 173:1–173:10.Google ScholarDigital Library
28. M. Nielsen and O. Osterby. 2013. A Two-continua Approach to Eulerian Simulation of Water Spray. ACM Trans Graph 32, 4 (2013), 67:1–67:10.Google ScholarDigital Library
29. D. Peachey. 1986. Modeling Waves and Surf. SIGGRAPH Comput Graph 20, 4 (1986), 65–74. Google ScholarDigital Library
30. D. Ram, T. Gast, C. Jiang, C. Schroeder, A. Stomakhin, J. Teran, and P. Kavehpour. 2015. A material point method for viscoelastic fluids, foams and sponges. In Proc ACM SIGGRAPH/Eurograph Symp Comp Anim. 157–163. Google ScholarDigital Library
31. B. Ren, Y. Jiang, C. Li, and M. Lin. 2015. A simple approach for bubble modelling from multiphase fluid simulation. Comp Vis Media 1, 2 (2015), 171–181. Google ScholarCross Ref
32. B. Ren, C. Li, X. Yan, M. Lin, J. Bonet, and S. Hu. 2014. Multiple-Fluid SPH Simulation Using a Mixture Model. ACM Trans Graph 33, 5 (2014), 171:1–171:11.Google ScholarDigital Library
33. D. Robert and K. Soga. 2013. Soil-Pipeline Interaction in Unsaturated Soils. In Mechanics of Unsaturated Geomaterials, Lyesse Laloui (Ed.). Wiley Online Library, Chapter 13, 303–325. Google ScholarCross Ref
34. W. Rungjiratananon, Z. Szego, Y. Kanamori, and T. Nishita. 2008. Real-time Animation of Sand-Water Interaction. Comp Graph Forum 27, 7 (2008), 1887–1893.Google ScholarCross Ref
35. O. Song, H. Shin, and H. Ko. 2005. Stable but Nondissipative Water. ACM Trans Graph 24, 1 (2005), 81–97. Google ScholarDigital Library
36. A. Stomakhin, C. Schroeder, L. Chai, J. Teran, and A. Selle. 2013. A Material Point Method for snow simulation. ACM Trans Graph 32, 4 (2013), 102:1–102:10.Google ScholarDigital Library
37. A. Stomakhin, C. Schroeder, C. Jiang, L. Chai, J. Teran, and A. Selle. 2014. Augmented MPM for phase-change and varied materials. ACM Trans Graph 33, 4 (2014), 138:1–138:11.Google ScholarDigital Library
38. D. Sulsky, S. Zhou, and H. Schreyer. 1995. Application of a particle-in-cell method to solid mechanics. Comp Phys Comm 87, 1 (1995), 236–252. Google ScholarCross Ref
39. T. Takahashi, H. Fujii, A. Kunimatsu, K. Hiwada, T. Saito, K. Tanaka, and H. Ueki. 2003. Realistic Animation of Fluid with Splash and Foam. Comp Graph Forum 22, 3 (2003), 391–400.Google ScholarCross Ref
40. N. Thürey, F. Sadlo, S. Schirm, M. Müller-Fischer, and M. Gross. 2007. Real-time Simulations of Bubbles and Foam Within a Shallow Water Framework. In Proc ACM SIGGRAPH/Eurograph Symp Comp Anim. Eurographics Association, 191–198.Google Scholar
41. L. Yang, S. Li, A. Hao, and H. Qin. 2014. Hybrid Particle-grid Modeling for Multi-scale Droplet/Spray Simulation. Comp Graph Forum 33, 7 (2014), 199–208.Google ScholarDigital Library
42. T. Yang, J. Chang, B. Ren, M. Lin, J. Zhang, and S. Hu. 2015. Fast Multiple-fluid Simulation Using Helmholtz Free Energy. ACM Trans Graph 34, 6 (2015), 201:1–201:11.Google ScholarDigital Library
43. Y. Yue, B. Smith, C. Batty, C. Zheng, and E. Grinspun. 2015. Continuum foam: a material point method for shear-dependent flows. ACM Trans Graph 34, 5 (2015), 160:1–160:20.Google ScholarDigital Library
44. Y. Zhu and R. Bridson. 2005. Animating sand as a fluid. ACM Trans Graph 24, 3 (2005), 965–972. Google ScholarDigital Library